What is Calculus - Lesson 2 | Limits | Don't Memorise

Infinity Learn NEET
2 Feb 201911:14
EducationalLearning
32 Likes 10 Comments

TLDRThe video script delves into the intriguing world of calculus to unravel common puzzles, such as calculating instantaneous speed and tackling Zeno's Dichotomy paradox. It explains how calculus can be used to find the optimal angle for throwing a stone to cover maximum distance and to determine the area of shapes with curved boundaries. The script clarifies the misconception that a falling ball should never reach the floor by illustrating the concept of limits and the summation of infinite terms, which approach a definite value. The example of a square being divided into infinite parts demonstrates how the sum of these parts can equal a finite area, paralleling the finite time it takes for a ball to hit the ground. This exploration of limits is fundamental to understanding calculus and its applications.

Takeaways
  • 🔍 The script discusses how calculus can be used to solve complex problems, such as finding the speed of a falling object at an instant.
  • 💡 It introduces the Zeno’s Dichotomy paradox, which questions why a falling ball should ever reach the floor if it must first cover an infinite number of diminishing distances.
  • 🤔 Nora's confusion is highlighted, as she believes the ball should never reach the floor due to the infinite steps it must take during its fall.
  • 📚 The concept of 'infinite' is clarified, emphasizing that it does not represent a number but rather something that never ends or is limitless.
  • ⏱ The script explains that to find the total time it takes for the ball to reach the floor, one must consider the sum of an infinite series of time intervals.
  • 🧩 The process of taking the limit in calculus is introduced as a method to solve problems involving infinite series, such as the total time for the ball to reach the floor.
  • 📉 By simplifying the problem and assuming constant speed, the script demonstrates that the time taken for each step gets closer to zero but never actually becomes zero.
  • 📏 An analogy is made with dividing a square into smaller and smaller parts to illustrate how the sum of an infinite series of areas can equal a finite number.
  • 📉 The script shows that the sum of the time taken for the ball to cover each step approaches a definite number (2 seconds), contrary to the initial assumption of infinite time.
  • 📚 The idea of limits is presented as central to understanding calculus and resolving paradoxes like Zeno’s Dichotomy.
  • 🔄 The script ends with a teaser for the next part, where the instantaneous speed of the ball will be discussed using calculus.
Q & A

  • What is the Zeno's Dichotomy paradox mentioned in the script?

    -The Zeno's Dichotomy paradox is a philosophical problem that suggests that an object in motion, like a falling ball, should never reach its destination because it must first cover half the distance, then half of the remaining distance, and so on, creating an infinite number of steps that it must complete before reaching the floor.

  • Why does Nora think that the ball should never reach the floor?

    -Nora is convinced that mathematically, the ball should never reach the floor because she believes that the ball has to cover an infinite number of steps, each being half the distance of the previous one, which implies that the process of falling never ends.

  • What is the mistake in Nora's logic according to the script?

    -The mistake in Nora's logic is that she assumes the total time taken by the ball to cover an infinite number of steps will be infinite. However, in reality, the sum of the time taken for each step approaches a finite number, which is the actual time it takes for the ball to reach the floor.

  • How does the script explain the concept of infinite in the context of the falling ball?

    -The script explains that 'infinite' does not mean a number but rather something that never ends or is limitless. In the context of the falling ball, it means that there are an infinite number of steps the ball has to perform, but the total time taken approaches a definite finite number, not infinity.

  • What is the process known as 'taking the limit' mentioned in the script?

    -The process known as 'taking the limit' is a central idea in calculus used to solve problems involving infinite series or processes. It involves finding the value that a function or sequence approaches as the input approaches infinity or some other value.

  • How does the script simplify the problem of the falling ball to make it more understandable?

    -The script simplifies the problem by assuming the ball falls with a constant speed of half a meter per second, which makes the calculations of time taken for each step straightforward and helps to illustrate the concept of limits and infinite series.

  • What is the significance of the time taken by the ball to perform each step getting closer to zero?

    -The significance is that even though each step requires some time, the time taken for each subsequent step gets closer to zero. This means that the total time taken by the ball to reach the floor approaches a definite finite number, which contradicts the idea that it would take an infinite amount of time.

  • How does the script use the example of a square to illustrate the concept of infinite sums?

    -The script divides a square into halves repeatedly, creating an infinite number of smaller squares. It demonstrates that even though the process of dividing is never-ending, the sum of the areas of these parts approaches the total area of the original square, which is a definite number.

  • What is the relationship between the time taken by the ball to reach the floor and the areas of the parts of the square?

    -The relationship is that both involve infinite sums. The time taken by the ball to reach the floor is the sum of the time taken for each step, which approaches a finite number. Similarly, the areas of the parts of the square, when added together, approach the total area of the square, which is also a finite number.

  • How does the script conclude that Nora's understanding of the infinite steps leading to an infinite time is incorrect?

    -The script concludes that Nora's understanding is incorrect by demonstrating that the total time taken by the ball to cover the infinite steps approaches a definite finite number, which is the actual time it takes for the ball to reach the floor, not infinity.

Outlines
00:00
🤔 Zeno's Paradox and Calculus

This paragraph introduces the central theme of the video script: the application of calculus to solve age-old problems that have puzzled people, such as Zeno's Dichotomy paradox. It discusses Nora's confusion regarding the concept that a falling ball should never reach the floor if one considers the infinite number of steps it must take to do so. The script also mentions other problems like finding the optimal angle to throw a stone for maximum distance and calculating the area of shapes with curved boundaries. The focus is on the Zeno's paradox and the introduction of the concept of limits in calculus, which is essential to understanding how an infinite series of actions can lead to a finite result, debunking Nora's paradoxical thought.

05:02
📏 The Infinite Steps and the Concept of Limits

The second paragraph delves deeper into the Zeno's Dichotomy paradox, explaining Nora's thought process that leads her to believe the ball should never reach the floor due to the infinite number of half distances it must cover. It discusses the concept of infinite steps and the associated time periods, emphasizing that although the number of steps is infinite, the total time taken for the ball to reach the floor is finite. The script uses the example of a constant speed to simplify the explanation and introduces the idea of adding an infinite series of time periods, which is a central concept in calculus. It also provides a practical example with a square's area to illustrate how an infinite number of parts can sum up to a finite area, highlighting the importance of the limit process in calculus.

10:02
🏀 Understanding Instantaneous Speed and the Limit Process

The final paragraph builds upon the previous discussions to explain how calculus can be used to find the instantaneous speed of a falling ball, which was one of Nora's initial questions. It emphasizes that while the ball's motion is divided into an infinite number of steps, the total time taken to cover these steps approaches a definite number, not infinity. The script compares this to the distance covered by the ball, which also approaches a finite value despite being split into infinite parts. The paragraph concludes with a teaser for the next part of the discussion, where the instantaneous speed will be explored further, leaving the audience intrigued and looking forward to the continuation of the topic.

Mindmap
Keywords
💡Calculus
Calculus is a branch of mathematics that deals with rates of change and accumulation. In the video, it is presented as the key to solving various problems, such as finding the speed of a falling object at an instant or determining the area of shapes with curved boundaries. Calculus is central to the discussion, as it provides the mathematical tools to address the paradoxes and problems presented, like the Zeno's Dichotomy paradox and the process of finding the area under a curve.
💡Instantaneous Speed
Instantaneous speed refers to the speed of an object at a specific moment in time, as opposed to its average speed over a period. In the context of the video, Nora is curious about how to determine the speed of a ball at an instant as it falls. This concept is fundamental to understanding motion in calculus, where the rate of change of distance with respect to time at a particular instant is calculated using derivatives.
💡Zeno’s Dichotomy Paradox
Zeno’s Dichotomy Paradox is a philosophical problem that suggests an object in motion, like a falling ball, should never reach its destination because it must first cover half the distance, then half of the remaining distance, and so on ad infinitum. The video uses this paradox to illustrate the limitations of a naive understanding of infinity and the need for calculus to resolve such paradoxes by summing an infinite series of distances or times.
💡Infinite
The term 'infinite' in the video is used to describe a quantity that never ends or is limitless. Nora's confusion arises from the idea that an infinite number of steps implies an infinite amount of time to reach the floor. However, the video explains that while the steps are infinite in number, the total time taken can still be finite, which is a key concept in understanding calculus and its handling of infinite series.
💡Limit
In calculus, a limit is the value that a function or sequence 'approaches' as the input or index approaches some value. The video script discusses the concept of limits as a way to understand the sum of an infinite series, such as the time it takes for a ball to hit the floor when considering an infinite number of steps. By taking the limit, one can find a finite answer to a problem that initially seems to involve an infinite process.
💡Area Under a Curve
The area under a curve is a concept in calculus that involves finding the total area between a curve and the x-axis. The video mentions this concept in relation to finding the area of shapes with curved boundaries. This is typically done using integral calculus, which can sum the areas of infinitesimally small rectangles under the curve to find the total area.
💡Constant Speed
In the video, to simplify the explanation of the Zeno's Dichotomy paradox, the ball's speed is assumed to be constant. This assumption allows for a straightforward calculation of the time it takes for the ball to fall one meter, which is two seconds at a speed of half a meter per second. The concept of constant speed is used here to illustrate the idea that even with a simplified model, calculus can provide a clear answer to seemingly paradoxical problems.
💡Successive Steps
Successive steps refer to the individual parts or intervals into which a process is divided. In the context of the video, the ball's fall is conceptualized as a series of successive steps, each covering a smaller distance than the last. The time taken for each step gets smaller and smaller, approaching zero, but never actually reaching it, which is a key point in understanding the resolution of Zeno's paradox using calculus.
💡Approach
The term 'approach' in the video is used to describe how the sum of an infinite series of terms gets closer and closer to a certain value without ever reaching it. For example, as the ball completes successive steps, the total time taken approaches a definite number, which is two seconds in this case. This concept is crucial for understanding limits and the behavior of infinite series in calculus.
💡Derivatives
Although not explicitly mentioned in the transcript, derivatives are a fundamental concept in calculus that would be necessary for finding the instantaneous speed of the ball, as mentioned in the video. Derivatives represent the rate at which one quantity changes with respect to another, and in the context of motion, they can be used to determine the speed of an object at any given instant.
Highlights

Nora's curiosity about finding the speed of a falling ball at an instant is introduced as a key problem.

The Zeno’s Dichotomy paradox is presented, questioning why a mathematically never-ending process of halving distances allows a ball to reach the floor.

Calculus is identified as the mathematical tool capable of resolving the paradoxes and problems discussed.

The concept of throwing a stone to maximize distance from a cliff is introduced as a problem that requires calculus for the solution.

Finding the area of shapes with curved boundaries is another problem that calculus can solve.

The importance of understanding limits in calculus to address the infinite steps in the Zeno’s Dichotomy paradox is emphasized.

The process of taking the limit in calculus is introduced as a method to sum an infinite series of values.

A simplified model of a ball falling with a constant speed is used to illustrate the concept of limits.

The time taken for the ball to cover each successive step gets closer to zero, illustrating the limit process.

The sum of the time periods for the ball's steps is shown to approach a definite number, contradicting the infinite time assumption.

An analogy of dividing a square into infinite parts is used to demonstrate that the sum of infinite terms can equal a definite number.

The concept of 'approaching' a number is highlighted as crucial in understanding the limit process.

Nora's incorrect assumption of infinite time for an infinite number of steps is corrected using the limit process.

A demonstration is provided to show how the cumulative time taken by the ball approaches two seconds for each step.

The idea that the sum of infinite terms can approach a definite number is used to explain the ball's motion and the area of a square.

The instantaneous speed of the ball is mentioned as a future topic to be explored with calculus.

Transcripts

Browse More Related Video

Why Calculus? - Lesson 1 | Infinity Learn NEET

What is Calculus - Lesson 3 | Differentiation | Don't Memorise

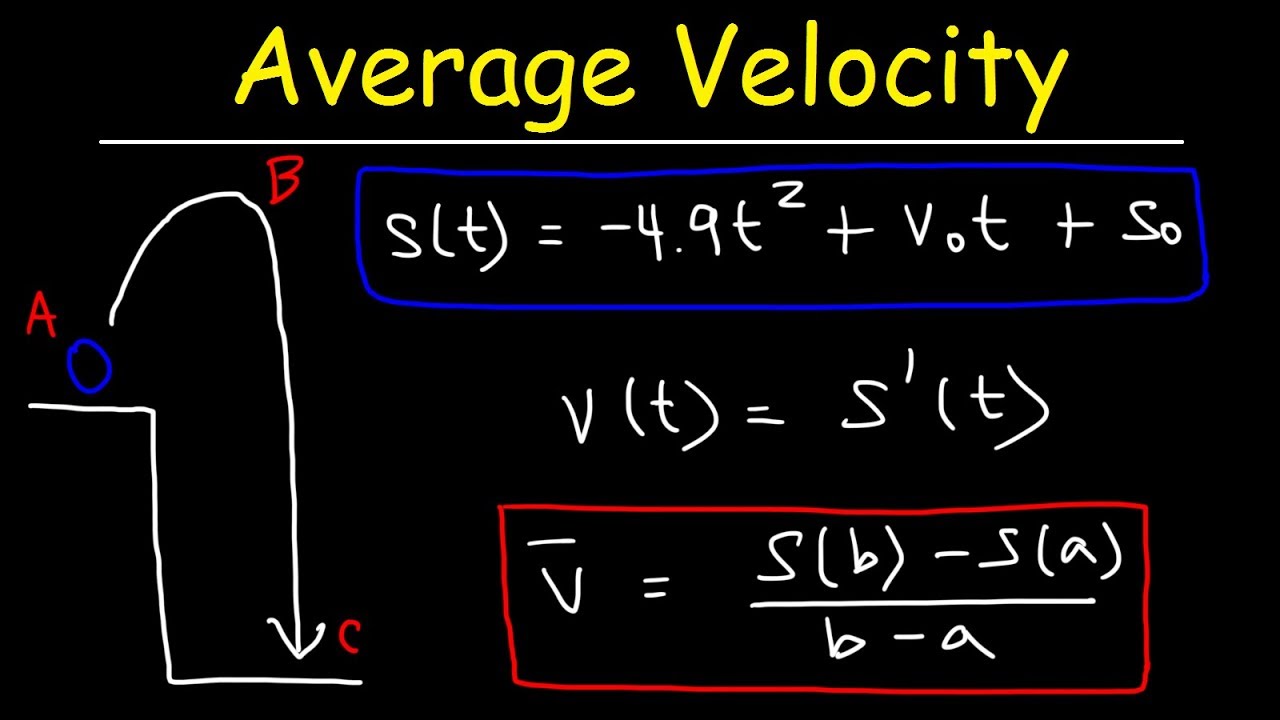
Average Velocity and Instantaneous Velocity

Business Calculus - Math 1329 - Sections 5.4 & 5.5 - Applications of the Definite Integral

Calculus Chapter 4 Lecture 36 Surface Area

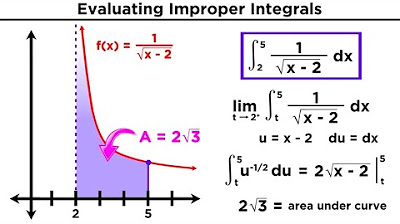
Evaluating Improper Integrals

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
CalculusZeno's ParadoxInstantaneous SpeedProjectile MotionArea CalculationLimitsInfinite SeriesParadox SolvingMathematical ConceptsEducational Content