The Mandelbrot Set: Atheists’ WORST Nightmare
TLDRThe speaker explores the intricate relationship between mathematics and the Christian worldview, suggesting that the abstract nature of numbers and the complex beauty of fractals like the Mandelbrot set imply a divine intelligence. They delve into the concept of sets in mathematics, the discovery of the Mandelbrot set, and its infinite complexity, which includes the presence of complex and imaginary numbers. The talk highlights the unexpected and stunning visual patterns that emerge from mathematical formulas, which the speaker argues reflect the infinite intelligence of God. They also touch upon the broader implications of why the physical universe follows mathematical laws, proposing that it is because the universe is upheld by the mind of God, who thinks in mathematical terms. The speaker concludes by emphasizing the harmony between the Christian perspective and the existence of mathematical truths, suggesting that the beauty and complexity found within numbers and fractals are a testament to God's nature.
Takeaways
- 🌟 The speaker suggests that the complexity and beauty found within mathematical concepts like the Mandelbrot set imply an intelligent design, specifically within a Christian worldview.
- 🔍 The Mandelbrot set, discovered in the 1970s-1980s, is a collection of numbers that, when plotted, form an infinitely complex and beautiful fractal pattern.
- 📐 The set is defined by a simple mathematical formula involving iterations of numbers, where those that remain stable are part of the set, and those that diverge are not.
- 🎨 The visual representation of the Mandelbrot set includes complex and imaginary numbers, which are real components in the mathematical structure despite their abstract nature.
- 🌐 The infinite complexity and self-similar patterns (fractals) within the Mandelbrot set at various scales are indicative of God's infinite intelligence and mind, as per the speaker's perspective.
- 🌌 The speaker draws parallels between the mathematical patterns found in the abstract and those observed in the physical world, such as in snowflakes, ferns, and coastlines, suggesting a divine connection.
- ❓ The question of why the physical universe obeys mathematical laws is posed as a challenge to secular worldviews, with the speaker arguing that it makes sense in the context of a Christian God who thinks mathematically.
- 🤔 The speaker ponders the reason behind the existence of mathematical truths and their abstract nature, suggesting that they are a reflection of God's mind and therefore exist prior to humans.
- 💡 The concept of numbers as abstract conceptions of quantity that exist in the mind is emphasized, highlighting that they are not physical entities but rather conceptual ones.
- 🌿 The idea that the physical world contains approximations of fractals, unlike the perfect fractals found in mathematics, is discussed as an expected outcome in the Christian view of creation.
- 📈 The speaker concludes that the ability to do science and understand mathematical laws is a gift from God, allowing humans to access some of His thoughts, which is seen as part of divine revelation.
Q & A
What is the main idea presented in the script about the relationship between numbers and the Christian worldview?
-The script suggests that numbers, being abstract concepts, are part of God's creation and reflect His infinite intelligence. It implies that the complexity and beauty found within mathematical structures, such as the Mandelbrot set, are indicative of the mind of God and make sense within a Christian worldview.
What is the significance of the Mandelbrot set in the context of the script?
-The Mandelbrot set is highlighted as an example of a mathematical structure that demonstrates infinite complexity and beauty, which the speaker correlates with the infinite nature of God's mind. The set's ability to repeat its pattern infinitely at various scales is seen as a reflection of divine intelligence.
How does the speaker use the concept of fractals to illustrate a point about God's mind?
-The speaker uses fractals, specifically the self-similarity and infinite complexity found in the Mandelbrot set, to illustrate the concept of an infinite mind. The idea is that just as fractals repeat their patterns without end, God's mind is also infinite and complex beyond human comprehension.
What role does the speaker believe God plays in the creation of numbers?
-The speaker believes that God is responsible for the creation of numbers, as they are abstract concepts that exist in the mind. This implies that God's sovereignty extends over the abstract, just as it does over the physical universe.
How does the script connect the abstract nature of numbers to the concept of God's sovereignty?
-The script connects the abstract nature of numbers to God's sovereignty by asserting that since numbers are abstract and conceptual, and God is responsible for all creation, He must also be responsible for the existence of numbers. This reflects His dominion over both the physical and the abstract.
What is the 'unreasonable effectiveness of mathematics' as mentioned by Dr. Eugene Wigner?
-Dr. Eugene Wigner refers to the 'unreasonable effectiveness of mathematics' in describing the surprising and perplexing fact that abstract mathematical concepts so accurately describe and predict the physical world. This effectiveness is seen as a 'miracle' or 'wonderful gift' that science has yet to fully explain.
Why does the speaker argue that the existence and properties of mathematical truths make sense within a Christian worldview?
-The speaker argues that mathematical truths, being abstract and universal, make sense within a Christian worldview because they reflect the mind of God. In Christianity, God is seen as omnipresent, unchanging, and the source of all truth, which aligns with the invariant and abstract nature of mathematical laws.
What is the main difference between the set of even numbers and the set of negative numbers as described in the script?
-The main difference lies in the defined property that characterizes each set. The set of even numbers includes numbers that are evenly divisible by two, while the set of negative numbers includes numbers that are less than zero. The determination of whether a number belongs to these sets is based on these properties.
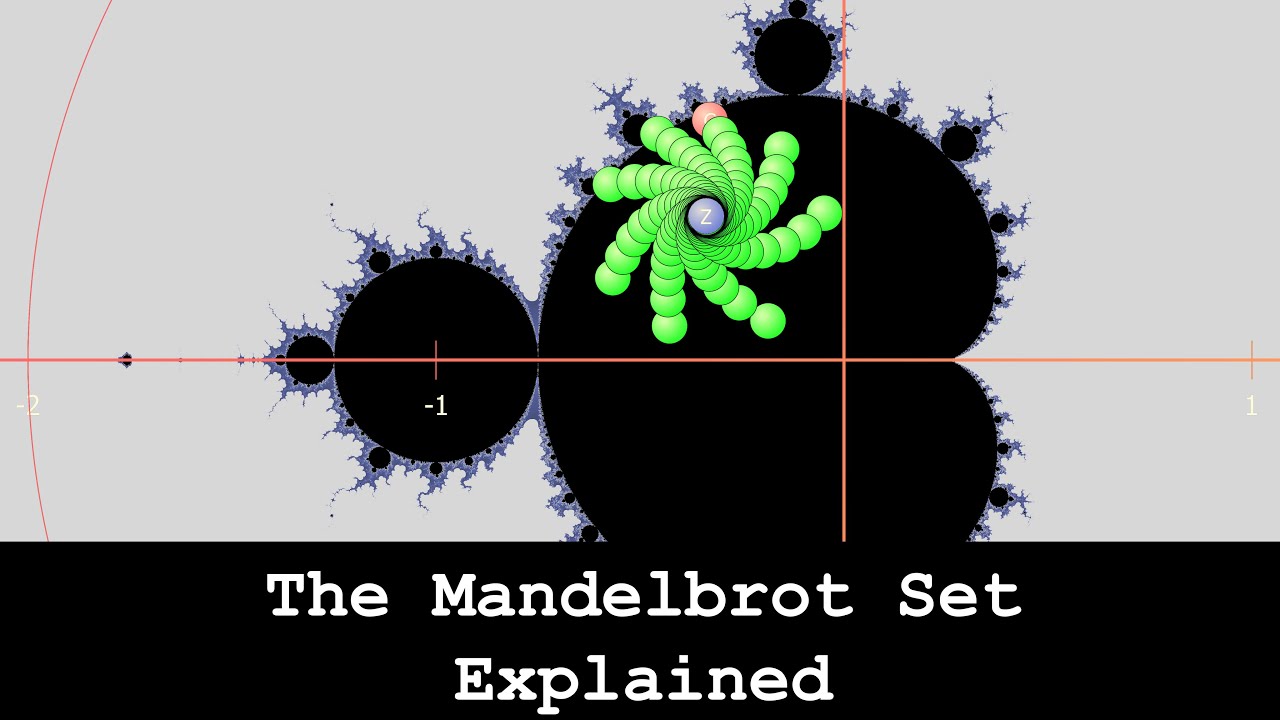
How does the speaker describe the process of determining if a number is part of the Mandelbrot set?
-The speaker describes a process involving a specific formula where a number 'c' is tested to see if it belongs to the Mandelbrot set by checking if another number 'Z' remains small after successive iterations according to the formula. If the sequence of 'Z' values stays small, then 'c' is part of the set; if the sequence grows very large, then 'c' is not.
What is the significance of the term 'fractal' as used in the script?
-The term 'fractal' is used to describe a pattern or shape that repeats itself at different scales or sizes, often indefinitely. In the context of the script, the Mandelbrot set is an example of a fractal, demonstrating infinite complexity and self-similarity at varying levels of magnification.
How does the speaker use the concept of the Mandelbrot set to argue for the existence of God?
-The speaker uses the Mandelbrot set's intricate and infinitely complex design as evidence of a designer, arguing that such complexity and beauty imply an intelligent creator. This aligns with the Christian belief in a God whose mind is infinite and who is the source of all creation, including the abstract concepts of mathematics.
What is the role of computers in revealing the complexity and beauty of the Mandelbrot set?
-Computers play a crucial role in revealing the complexity and beauty of the Mandelbrot set by allowing for the rapid iteration of the set's formula across numerous points. This enables the visualization of the set's intricate patterns and self-similar structures, which would be impractical to achieve manually.
Outlines
😃 The Christian View of a Secret Code in Numbers
The speaker discusses the idea that a secret code embedded in numbers can only be understood within a Christian worldview. They reference the movie 'National Treasure' as an analogy for discovering hidden codes and suggest that finding such a code in numbers would imply the intelligence of the Creator. The speaker emphasizes that God is responsible for all creation, including abstract concepts like numbers. They introduce the concept of sets in mathematics and use the Mandelbrot set as an example of a complex set that includes real and complex numbers, defined by a specific formula. The speaker shares their fascination with the 'mind-blowing' secret code in numbers, hinting at the intricate connection between mathematics and the Christian understanding of God's sovereignty over the universe.
🧮 Exploring the Mystifying World of Complex Numbers
The paragraph delves into the concept of complex and imaginary numbers, which are numbers that result in a negative value when squared. The speaker clarifies that these numbers are indeed real and exist, despite their name suggesting otherwise. They explain that imaginary numbers are represented by the symbol 'i' and are neither positive, negative, nor zero. To help understand these abstract concepts, the speaker suggests visualizing them on a different axis from real numbers. The paragraph also touches on the idea of complex numbers, which have both real and imaginary parts. The speaker then discusses the use of computers to analyze the Mandelbrot set, a set of complex numbers, and how it has revealed patterns that were not previously apparent, highlighting the interplay between mathematical concepts and the physical representation of these through technology.
🌀 The Fractal Nature of the Mandelbrot Set
The speaker describes the intricate and infinitely complex nature of the Mandelbrot set, a mathematical fractal that exhibits self-similarity at different scales. They explain how the set, when plotted, reveals a heart-shaped main body known as a cardioid, with additional circular features and tendrils that extend from it. The speaker points out the unexpected ability of the set to 'count' as evidenced by the number of branches extending from certain points. They also discuss the presence of mini Mandelbrot sets within the larger set, each repeating the pattern on a smaller scale. The speaker concludes by suggesting that the shape of the Mandelbrot set reflects the infinite intelligence and nature of God, as understood in the Christian worldview, and that the study of numbers and their inherent patterns can provide insight into the divine.
🎨 The Aesthetic and Spiritual Significance of the Mandelbrot Set
The speaker explores the aesthetic appeal of the Mandelbrot set, noting its intricate beauty and the sense of wonder it inspires. They discuss the various patterns within the set, such as the 'Valley of the Seahorses' and the 'Valley of the Double Spirals,' highlighting the natural beauty found within mathematical structures. The speaker also touches on the concept of fractals in nature, such as in snowflakes and ferns, and how these natural occurrences mirror the mathematical fractals. They emphasize that the beauty and complexity found in the Mandelbrot set and other fractals are not a product of human creation or computer rendering but are inherent in the mathematical concepts themselves. The speaker concludes by suggesting that the presence of such beauty in numbers and mathematical structures points to a divine creator who values beauty and is the source of the order and beauty observed in both the mathematical and natural worlds.
🤔 The Origins and Impact of Mathematical Laws
The speaker ponders the origins of mathematical laws and questions whether they evolved or were created. They argue against the idea that humans created mathematical laws, stating that if this were true, humans could alter them at will, which is not the case. The speaker asserts that mathematical laws are universal, invariant, and abstract, and therefore, they must have always existed. They propose that the existence of mathematical laws and the ability of humans to discover them can be best explained within a Christian framework, where God's mind is seen as the source of these laws. The speaker also addresses the question of why the physical universe obeys mathematical laws, suggesting that this is because the universe is upheld by God's mind, which thinks mathematically. They contrast this with the secular perspective, which struggles to explain the reason behind the physical universe's adherence to mathematical laws.
❓ The Existence of Fractals in Both the Conceptual and Physical Realms
The speaker questions why fractals, which are conceptual shapes found in mathematics, also appear in the physical world. They provide examples of fractals in nature, such as frost patterns, ferns, Romanesco broccoli, coastlines, mountaintops, and clouds. The speaker points out that these natural fractals are approximations of the perfect fractals found in mathematics, and they question why the physical universe would exhibit patterns that exist only in the conceptual realm. They argue that the existence of fractals in both realms can be explained within a Christian worldview, where God's mind, which thinks in mathematical terms, upholds the physical universe. The speaker suggests that the ability to do science and understand the universe is a gift from God, allowing humans to access some of His thoughts. They conclude by emphasizing that the Christian worldview provides a coherent explanation for the beauty, complexity, and existence of mathematical truths and their reflection in the physical world.
Mindmap
Keywords
💡Mandelbrot Set
💡Fractals
💡Complex Numbers
💡Imaginary Numbers
💡Cardioid
💡Numbers as Abstract Concepts
💡Christian Worldview
💡Secular Viewpoint
💡Eugene Wigner
💡Beauty in Mathematics
💡Zooming and Scaling
Highlights
The speaker suggests that a secret code embedded in numbers can only be understood within a Christian worldview.
A comparison is made to the movie 'National Treasure,' where a code on the Declaration of Independence leads to further clues.
The existence of a secret code within numbers implies the intelligence of the creator, suggesting that God is responsible for numbers as well as the physical universe.
The concept of a set in mathematics is introduced, defined by numbers with a common property.
The Mendelbrot set is described as a complex set defined by a specific formula, which includes real and complex numbers.
The process of determining if a number is part of the Mendelbrot set is explained through an iterative formula.
Complex numbers and their role in the Mendelbrot set are discussed, explaining their existence despite being abstract.
The speaker shares his astonishment at the discovery of the Mendelbrot set and its implications for understanding the nature of God's intelligence.
The visual representation of the Mendelbrot set is described, noting its unexpected and intricate beauty.
The pattern within the Mendelbrot set, including the number of branches corresponding to odd numbers, is highlighted.
The concept of a fractal is introduced, noting the infinite repetition and complexity within the set.
The speaker proposes that the shape of the Mandelbrot set reflects the infinite intelligence of God.
The exploration of changing the formula of the Mandelbrot set to observe different mathematical shapes is mentioned.
The beauty and complexity found in fractals are attributed to the inherent properties of mathematics rather than human creation or computer rendering.
The question of why the physical universe obeys mathematical laws is posed, with the speaker suggesting a Christian perspective provides a coherent explanation.
Dr. Eugene Wigner's article 'The Unreasonable Effectiveness of Mathematics' is referenced, highlighting the perplexity from a secular viewpoint.
The speaker concludes that the Christian worldview aligns with the existence and properties of numbers and mathematical truths, suggesting they are part of God's mind.
Transcripts
Browse More Related Video

Is the Logistic Map hiding in the Mandelbrot Set? | #SoME3

Benoit Mandelbrot: Fractals and the art of roughness

The Beauty of Math and Music | Marcus Miller | TEDxOttawa

Mathematician Explains Infinity in 5 Levels of Difficulty | WIRED

Why Do People Hate Mathematics? Efim Zelmanov (Fields Medal 1994)
The Mandelbrot Set Explained
5.0 / 5 (0 votes)
Thanks for rating: