Seven Dimensions
TLDRThe video script delves into the concept of the International System of Units (SI), which comprises seven base units defined by physical constants. It explores alternative unit systems, such as the humorous '7 Cs' and the more practical Planck units, which simplify physics calculations by setting key constants to 1. The script introduces a novel approach to understanding and converting between unit systems by treating them as vector spaces. This perspective allows for the application of linear algebra, making conversions more elegant and manageable, especially with the aid of matrices. The video also discusses the importance of 'coherence' in unit systems, where every quantity can be expressed in a unique way through base units, and presents a spreadsheet tool that automates the matrix calculations for any user-defined unit system. The summary concludes by emphasizing the flexibility of mathematical constructs like vectors, which can be adapted to represent various concepts to solve problems effectively.
Takeaways
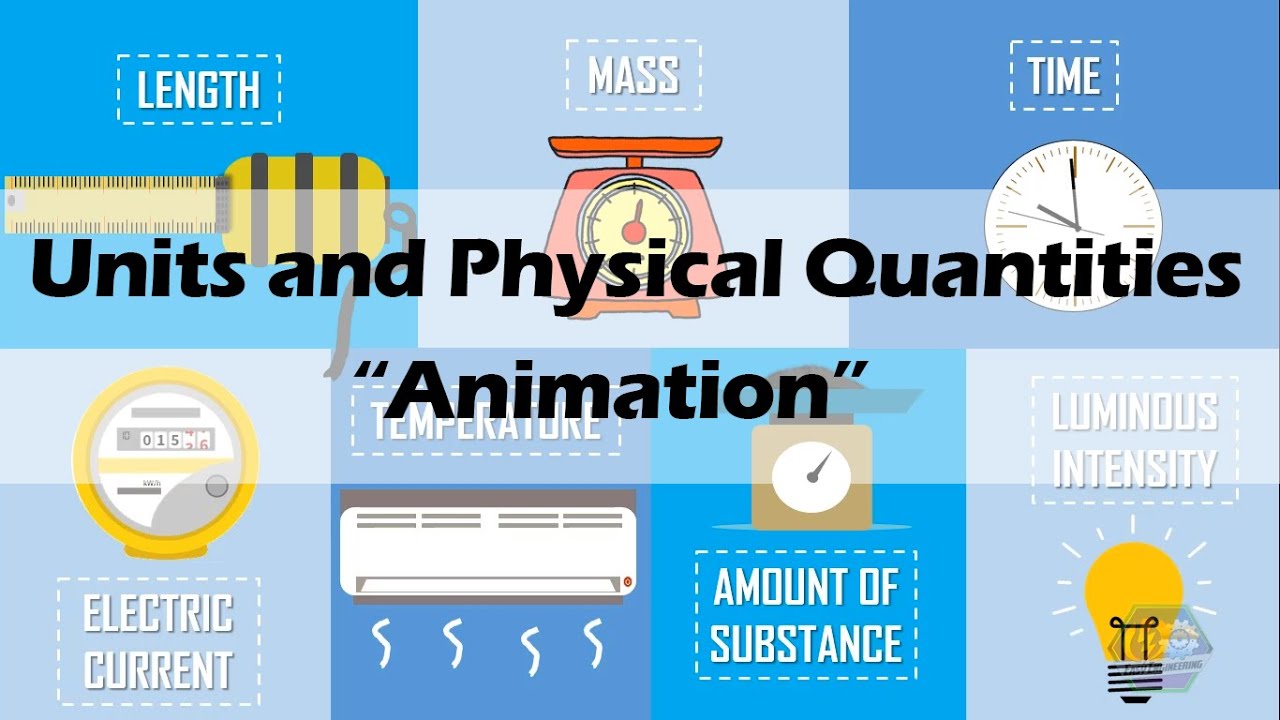
- 📏 The SI system of units is based on seven base units, each defined by a physical constant, and all other units can be derived from these base units in a unique way.
- 🎢 There are alternative systems to SI, such as the 7 Cs joke proposal and Planck units, which use different base quantities and are useful for specific applications in physics.
- 🧮 Thinking of units as coordinates in an abstract space allows for the application of linear algebra to unit systems, simplifying the mathematics behind conversions.
- 📈 Base quantities in a coherent system of units are represented as basis vectors in a multi-dimensional space, with each derived quantity having a unique vector.
- 🔍 The concept of 'coherence' in unit systems refers to the ability to express every quantity either uniquely or not at all through multiplication and division of base units.
- 📐 Vector addition in this context corresponds to multiplying quantities, and scalar multiplication corresponds to raising a quantity to a power.
- 🧬 The axioms of linear algebra must be satisfied for the system to be valid, and they provide a framework for verifying the system's consistency.
- 🔗 Matrices are used to convert between different unit systems by performing a 'change of basis', where each row and column represent base quantities of the respective systems.
- ↔️ Inverting the conversion matrix allows for the transformation from one system to another, with computers facilitating this process.
- 🌐 The spreadsheet tool mentioned in the script automates the matrix calculations for any coherent system of units, providing a practical application of the theoretical framework.
- ⚖️ Coherence can also be tested by checking the determinant of the conversion matrix from the system to SI; a non-zero determinant indicates a coherent system.
Q & A
What are the seven base units of the SI system?
-The seven base units of the SI system are units for time (second), length (meter), mass (kilogram), electric current (ampere), temperature (kelvin), luminous intensity (candela), and amount of substance (mole).
What is a coherent system of units?
-A coherent system of units is one where all derived units are obtained by multiplying and dividing the base units without the need for any conversion factors.
What is the basis of the 7 Cs units system proposed by Jan Misali?
-The 7 Cs units system is based on frequency, velocity, and energy, with all base units represented by some variation on the letter C, and is meant as a humorous alternative to the SI system.
How are the base quantities of the Planck units expressed in terms of SI?
-The base quantities of the Planck units are quite complicated to express in terms of SI and are not immediately obvious to untangle for deriving units of length, mass, and time.
What does treating units as vectors allow us to do?
-Treating units as vectors allows us to apply linear algebra to the system of units, making the mathematics behind using systems based on different units and converting between them more elegant.
What is a matrix in the context of unit systems?
-In the context of unit systems, a matrix represents a change of basis, allowing the conversion between two systems with different basis vectors.
How does the concept of a determinant help us determine the coherence of a unit system?
-The determinant of the matrix that converts a unit system into SI can be used to test for coherence. If the determinant is zero, the system is not coherent as it cannot be uniquely expressed by multiplying base units.
What is the significance of the order of writing SI base units in a fixed format?
-Writing SI base units in a fixed format (seconds, metres, kilograms, amps, kelvin, candelas, moles) ensures consistency and allows us to treat the units as labels in an ordered list, which is helpful for applying linear algebra.
How does the concept of a vector space help in understanding units?
-The concept of a vector space allows us to represent every possible quantity within a unit system as a unique vector. This representation aids in visualizing and calculating relationships between different quantities using the tools of linear algebra.
Why is the concept of linear independence important in defining a coherent system?
-Linear independence is important because it ensures that the basis vectors of a unit system are not redundant and that each derived unit can be expressed in exactly one way by multiplying base units, which is a requirement for a system to be coherent.
What practical use case is provided for the conversion matrix in the script?
-The Planck units, designed for ease in certain areas of physics, are provided as a practical use case. The conversion matrix allows expressing SI quantities in terms of Planck units, simplifying calculations in specific physical contexts.
How can one use the concepts discussed in the script to create their own unit system?
-One can use the concepts of vector spaces and linear algebra to define a new system of units by choosing a set of base quantities, representing them as basis vectors, and then using matrix operations to derive and convert all other quantities within that system.
Outlines
📏 The SI System and Unit Coherence
The SI system is a set of seven base units defined by physical constants, from which all other units can be derived uniquely. The base quantities include time, length, mass, current, temperature, luminous intensity, and amount of substance. The paragraph discusses alternative unit systems like the 7 Cs units and Planck units, which are more complex to express in SI terms. It introduces the concept of treating units as vectors in a multi-dimensional space, allowing for a new perspective on unit conversion and coherence. The mathematical elegance of using linear algebra for unit conversions is highlighted.
🧮 Linear Algebra in Unit Conversions
Linear algebra principles are applied to unit systems, with the paragraph explaining how basic operations like vector addition and scalar multiplication correspond to multiplying quantities and raising them to powers, respectively. The axioms of linear algebra are shown to apply to coherent unit systems. The concept of matrices in this context is introduced, with matrices representing the conversion between different unit systems, termed 'change of basis'. The example of converting between the 7 Cs system and SI is used to illustrate the process, emphasizing the utility of matrices for such conversions.
⚙️ Coherence and Inverting Matrices for Unit Systems
The concept of coherence in unit systems is refined to mean that every possible quantity can be expressed in exactly one way by multiplying base units. The determinant of the conversion matrix from a unit system to SI is proposed as a test for coherence. The paragraph discusses the process of inverting matrices to convert from one system to another, noting that modern computers can perform these calculations efficiently. The practicality of such conversions is illustrated with Planck units, which simplify certain physics calculations by setting fundamental constants to 1. The spreadsheet tool mentioned is a practical application that automates these matrix operations, allowing users to define custom unit systems and convert between them and SI units.
Mindmap
Keywords
💡SI system of units
💡Base units
💡Natural units
💡Vector space
💡Coherent system of units
💡Linear algebra
💡Matrix
💡Change of basis
💡Planck units
💡Capacitance
💡Determinant
Highlights
The SI system of units is based on seven base units defined by physical constants, allowing any other unit to be expressed as a product of powers of these base units.
Base quantities of the SI system include time, length, mass, current, temperature, luminous intensity, and amount of substance.
An alternative system proposed by Jan Misali derives units from frequency, velocity, and energy, with base units represented by variations on the letter C.
Planck units are a system of 'natural units' where base quantities are complex to express in SI and are used to simplify physics calculations.
A coherent system of units is defined as one where base units are multiplied and divided to derive all other units.
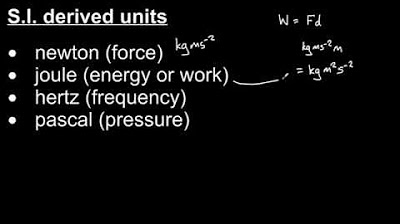
Capacitance, as an example, is expressed in SI base units as a product of time, length, and mass, highlighting the system's complexity.
Representing units as vectors in a seven-dimensional space allows for a unique vector for every possible quantity within the SI system.
Linear algebra can be applied to unit systems, making mathematics more elegant and simplifying conversions between different unit systems.
Basis vectors in the unit system represent quantities, not specific units, maintaining consistency across different measurement systems.
A change of basis, represented by a square matrix, allows conversion between different unit systems like the 7 Cs and SI systems.
The determinant of a conversion matrix can indicate the coherence of a unit system, with a non-zero determinant allowing for a unique expression of quantities.
Inverting the conversion matrix enables the conversion of SI units into any other coherent system of units.
Planck units, while not practical for everyday measurements, provide a simplified system for complex physics calculations.
The concept of coherence ensures that every quantity in a unit system can be expressed in exactly one way, avoiding ambiguity.
A spreadsheet tool is available for automatic matrix calculations, allowing users to create and analyze custom unit systems.
The use of vector spaces to represent unit systems demonstrates the flexibility of mathematical constructs and their application to novel problems.
Vectors are versatile mathematical tools that can represent a variety of concepts, extending beyond their traditional interpretation as arrows in space.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: