AP CALCULUS AB 2022 Exam Full Solution FRQ#4(a,b)

Weily Lin
17 May 202205:39
EducationalLearning
32 Likes 10 Comments

TLDRThe video script discusses a mathematical problem involving the rate of change in the radius of an ice sculpture that maintains a conical shape as it melts. The radius is modeled by a twice differentiable function, r(t), and the rate of change of the radius, r'(t), is given for a time interval from 0 to 12 days. Part A of the problem involves approximating the second derivative of r, r''(8.5), using the average rate of change of r' over the interval from 7 to 10 days. The calculation results in an approximate value of 0.2 cm/day^2. Part B explores whether there is a time between t=0 and t=3 when r' equals -6 cm/day, using the intermediate value theorem. Given that r' is continuous and smooth, and considering the values of r' at t=0 and t=3, it is concluded that there must be a time within the interval [0, 3] when r' equals -6 cm/day.

Takeaways
  • 🔍 The problem involves analyzing the melting of an ice sculpture modeled as a cone, focusing on the change in radius over time.
  • 📏 The radius 'r' of the cone's base changes according to a twice differentiable function, implying the function for radius and its derivative (rate of change) are smooth and continuous.
  • 📊 'r prime' (r') represents the rate of change of the radius in centimeters per day, as provided in a table for selected days.
  • 🔢 Part A of the problem requires calculating the second derivative of the radius 'r double prime' (r'') at day 8.5, using average rate of change of r' over the interval from day 7 to day 10.
  • 🧮 The calculation for r'' at 8.5 is performed by determining the slope of r' between days 7 and 10, yielding an approximation of 0.2 cm/day².
  • 🔄 Part B explores whether there is a time between day 0 and day 3 where r' equals -6 cm/day, leveraging the concept of the Intermediate Value Theorem.
  • 📈 Given the continuity of r' and the values at days 0 and 3, it's concluded that r' must reach -6 cm/day between these days due to the theorem.
  • 🌡️ The context of the problem, involving a melting ice sculpture, adds practical application to the mathematical concepts discussed.
  • 💡 The problem demonstrates the use of differential calculus in real-world modeling, specifically in understanding dynamics of changing physical dimensions.
  • 📝 The methodology used includes approximation techniques and theoretical mathematical theorems to address questions about rates of change and specific value occurrences.
Q & A

  • What is the topic of the discussion in the transcript?

    -The topic of the discussion is the mathematical modeling of an ice sculpture that melts while maintaining a conical shape. It involves the use of differential calculus to analyze the rate of change of the radius of the base of the cone over time.

  • What does 'r prime' represent in the context of the transcript?

    -In the transcript, 'r prime' (r') represents the rate of change of the radius of the ice sculpture's base, measured in centimeters per day.

  • Why is it important that r is a twice differentiable function?

    -It is important because being twice differentiable implies that the function r(t) is smooth and has a well-defined rate of change (r prime) and acceleration (r double prime), which are necessary for the mathematical modeling of the melting process.

  • How is r double prime (r'') estimated at t=8.5?

    -R double prime at t=8.5 is estimated using the average rate of change of r prime over the interval from t=7 to t=10. This is done by calculating the difference in r prime values at t=10 and t=7, then dividing by the difference in time (3 days).

  • What is the approximate value of r double prime at t=8.5?

    -The approximate value of r double prime at t=8.5 is 0.2 centimeters per day squared.

  • What does the question in part B of the transcript ask?

    -Part B asks if there is a time between t=0 and t=3 when r prime is equal to negative 6, and to justify the answer.

  • How is the Intermediate Value Theorem applied to answer part B?

    -The Intermediate Value Theorem is applied by noting that since r prime is continuous and changes from a value greater than -6 to a value less than -6 between t=0 and t=3, it must cross the value of -6 at some point within that interval.

  • What is the significance of the continuity of r prime in applying the Intermediate Value Theorem?

    -The continuity of r prime ensures that there are no gaps or jumps in its value, which is a prerequisite for applying the Intermediate Value Theorem. This theorem guarantees that if a continuous function takes two values, it will take every value in between at some point in the interval.

  • What does the term 'secant slope' refer to in the context of the transcript?

    -In the context of the transcript, 'secant slope' refers to the slope of the line that passes through two points on the graph of r prime, which in this case is used to approximate the average rate of change of r prime over an interval.

  • null

    -null

  • Why is it an approximation to use the secant slope to find r double prime at t=8.5?

    -It is an approximation because the actual value of r double prime at t=8.5 would require knowing the exact function for r prime, not just the values at specific points. The secant slope provides an estimate based on the given data points.

  • What is the unit of measurement for the rate of change of the radius as discussed in the transcript?

    -The unit of measurement for the rate of change of the radius, as discussed in the transcript, is centimeters per day.

  • How does the rate of change of the radius (r prime) relate to the physical process of the ice sculpture melting?

    -The rate of change of the radius (r prime) relates to the physical process of the ice sculpture melting by providing a mathematical representation of how quickly the size of the base of the ice sculpture is decreasing over time.

  • What is the role of the table of values given in the transcript?

    -The table of values given in the transcript provides selected data points for r prime at different times, which are used to perform calculations and make estimations about the behavior of the ice sculpture's melting process.

Outlines
00:00
⚙️ Calculating Approximate Second Derivative

In this paragraph, the narrator discusses the process of approximating the second derivative of a function representing the rate of change of radius of an ice sculpture modeled as a cone. The narrator explains that since the rate of change function is twice differentiable, it must be smooth and continuous. The task is to approximate the second derivative at a specific point using the average rate of change of the first derivative over an interval. The narrator calculates the average rate of change between two given points to approximate the second derivative at a specific value within the interval.

05:02
📉 Application of Intermediate Value Theorem

This paragraph focuses on the application of the intermediate value theorem to determine if there exists a time interval between 0 and 3 during which the rate of change of the radius is equal to -6. The narrator establishes that the rate of change function is continuous since the radius function is twice differentiable. By analyzing the given values of the rate of change function at t = 0 and t = 3, the narrator concludes that there must be a time within the interval where the rate of change is equal to -6, based on the intermediate value theorem.

Mindmap
Keywords
💡r prime
The term 'r prime' refers to the rate of change of the radius of the ice sculpture with respect to time, measured in centimeters per day. It is a key concept in the video as it is used to model the melting process of the ice sculpture, which maintains a conical shape as it decreases in size. The script mentions that 'r prime' is a twice differentiable function, which implies it is smooth and continuous, a necessary condition for applying calculus to find further derivatives such as 'r double prime'.
💡twice differentiable function
A 'twice differentiable function' is a mathematical function that has two derivatives, meaning it is smooth and has a well-defined rate of change and rate of change of that rate of change. In the context of the video, the function r(t) representing the radius of the ice sculpture is twice differentiable, which allows for the calculation of 'r prime' and 'r double prime'. This property is crucial for the mathematical modeling of the ice sculpture's melting process.
💡r double prime
The term 'r double prime' denotes the second derivative of the radius function r(t) with respect to time, which represents the acceleration in the rate of change of the radius. In the video, it is approximated using the average rate of change of 'r prime' over an interval from 7 to 10 days. This approximation is significant as it helps in understanding the rapidity of the ice sculpture's melting at a specific point in time, which is 8.5 days into the process.
💡average rate of change
The 'average rate of change' is a mathematical concept that describes the average rate at which a function's value changes over an interval. In the video, it is used to approximate 'r double prime' at 8.5 days by calculating the average slope of 'r prime' between 7 and 10 days. This approximation is a practical method to estimate the second derivative when exact values are not available.
💡conical shape
A 'conical shape' is a geometric shape that tapers smoothly from a circular base to a single point at the top, resembling the shape of a cone. The video discusses an ice sculpture that maintains a conical shape as it melts, which is important for the mathematical modeling of its changing dimensions over time.
💡ice sculpture
An 'ice sculpture' is a piece of art created by carving ice into various shapes and forms. In the video, the ice sculpture is the subject of a mathematical problem where its melting is modeled over time. The sculpture's conical shape and the rate at which it melts are central to the discussion and calculations presented.
💡intermediate value theorem
The 'intermediate value theorem' is a fundamental theorem in calculus that states if a function is continuous on a closed interval and takes on two values, then it also takes on every value between those two values. In the video, this theorem is used to justify that there exists a time between t=0 and t=3 when 'r prime' equals negative 6, given that 'r prime' is continuous and changes from negative 6.1 to negative 5.0 within that interval.
💡continuous function
A 'continuous function' is a function that does not have any abrupt changes in value and has a well-defined limit at every point in its domain. In the context of the video, the continuity of 'r prime' is essential for applying the intermediate value theorem to find a specific value of the function within a given interval.
💡calculus
Calculus is a branch of mathematics that deals with the study of change and motion, focusing on the concepts of derivatives and integrals. The video uses calculus to model the melting of an ice sculpture, specifically employing derivatives to understand the rate of change of the radius and its acceleration over time.
💡secant slope
The 'secant slope' is the slope of the line passing through two points on a curve, representing the average rate of change over that interval. In the video, the secant slope from t=7 to t=10 is used to approximate the 'r double prime' at t=8.5, which is a method to estimate the second derivative when exact values are not known.
💡centimeters per day
The term 'centimeters per day' is a unit of measurement used to express the rate of change of a quantity in centimeters for each day. In the video, it is used to quantify the rate at which the radius of the ice sculpture changes as it melts, which is crucial for the mathematical modeling of the sculpture's decay.
Highlights

The ice sculpture melts in a way that it can be modeled as a cone that maintains a conical shape as it decreases in size.

The radius of the base of the cone is given by a twice differentiable function r(t).

r'(t) is the rate of change of the radius, measured in centimeters per day.

r(t) is measured in centimeters and t is measured in days.

The table provides selected values of r'(t) over a time interval from 0 to 12 days.

Part a asks to approximate r''(8.5) using the average rate of change of r' over the interval from 7 to 10 days.

The average rate of change is the average slope of r', so r'' is the slope of r'.

Calculate the slope of r' from t=7 to t=10 to approximate r''(8.5).

r''(8.5) is approximately 0.2 cm/day^2, an approximation using tabular values.

Part b asks if there is a time between t=0 and t=3 when r' equals -6 cm/day.

Since r is twice differentiable, r' is smooth and continuous.

By the intermediate value theorem, since r'(0) = -6.1 and r'(3) = -5.0, r'(t) must equal -6 at some point in the interval 0 < t < 3.

The continuity of r' is a condition needed to use the intermediate value theorem.

The problem involves using the concept of derivatives to model a real-world scenario - the melting of an ice sculpture.

The problem requires approximating higher-order derivatives using basic principles of calculus.

The problem demonstrates the application of the intermediate value theorem in a practical context.

The problem illustrates the mathematical modeling of physical phenomena using calculus.

The problem requires interpreting and manipulating tabular data to solve a calculus problem.

The problem involves using the concept of rates of change to approximate quantities of interest.

The problem shows how to use basic calculus to analyze the behavior of a function over time.

The problem demonstrates the mathematical modeling of a physical process using differential equations.

Transcripts

Browse More Related Video

2022 AP Calculus AB BC Free Response #4

AP CALCULUS AB 2022 Exam Full Solution FRQ#4d

2007 AP Calculus AB Free Response #5

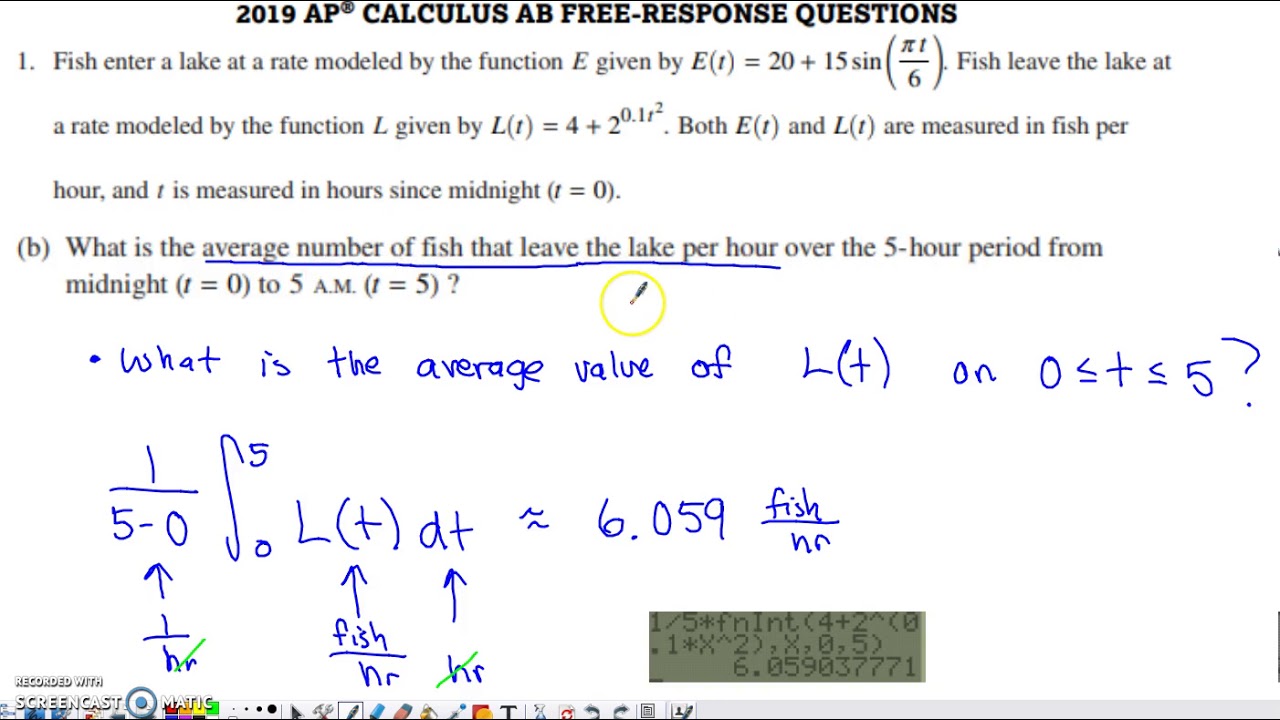
2019 AP Calculus AB & BC Free Response Question #1

Calculus BC 2008 2d | AP Calculus BC | Khan Academy

Applied rate of change: forgetfulness | Applications of derivatives | AP Calculus AB | Khan Academy

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Differential CalculusIce SculptureRadius ChangeConical ShapeMathematical ModelingRate of ChangeSecant SlopeApproximationContinuous FunctionIntermediate ValueTwice DifferentiableCentimeters per Day