Proof of the Calculus Power Rule using the Limit Definition of a Derivative.

Inobis Academy

4 Sept 202220:40

EducationalLearning

32 Likes 10 Comments

TLDRIn this educational video from Novus Academy, the presenter dives into the intricacies of derivatives, aiming to provide viewers with a deep understanding of the concept beyond mere memorization of formulas. The video begins with a review of the limit definition of a derivative, illustrating the concept with a clear graphical representation. The presenter then proceeds to derive the difference quotient, a fundamental step in understanding derivatives. The crux of the video is the proof of the power rule, a staple in calculus, which states that the derivative of x to the power of n is n times x to the power of n minus one. Using the binomial theorem and a bit of algebraic manipulation, the presenter simplifies the expression and applies the limit as delta x approaches zero to isolate the coefficient that defines the power rule. The video concludes with an encouragement to re-watch for clarity, emphasizing the importance of understanding the foundational rules of calculus for those delving into more advanced mathematical concepts.

Takeaways

📚 The video aims to provide a deep understanding of derivatives, not just the power rule and slope, but also to prove these concepts.
🔢 The limit definition of a derivative is introduced as the foundational concept, defined as the limit as delta x approaches zero of [f(x + delta x) - f(x)] / delta x.
📈 The concept of slope is used as an analogy to understand derivatives, with the slope being in the format of delta y over delta x.
📈 The function f(x) is equated to y, which helps in visualizing the function's value at a given x as a point on the graph.
📈 A graphical representation is used to illustrate the concept of the slope between two points as delta x approaches zero, emphasizing the instantaneous rate of change.
🧮 The difference quotient, denoted as delta f over delta x, is derived from the concept of slope and is used to find the slope between two points on the function's graph.
📐 The binomial theorem is applied to expand (x + delta x)^n, which is crucial for generalizing the power rule for any exponent n.
📐 The binomial expansion leads to a series of terms, where only the first two terms (related to n and n-1) are relevant as delta x approaches zero, with the rest considered 'junk' terms that approach zero.
🔑 The power rule of derivatives is proven by applying the limit definition and considering the behavior of the binomial expansion terms as delta x approaches zero.
🔑 The final result of the proof confirms the power rule: the derivative of x to the power of n (d/dx[x^n]) is equal to n times x to the power of n minus one (nx^(n-1)).
📝 The presenter encourages re-watching the video for clarity, as the proof of the power rule is fundamental to calculus and may be complex on the first viewing.

Q & A

What is the main purpose of the video?
-The main purpose of the video is to provide a deep understanding of derivatives, to prove the power rule of a derivative, and to showcase the limit definition of a derivative.
What is the limit definition of a derivative?
-The limit definition of a derivative is the limit as delta x approaches 0 of [f(x + delta x) - f(x)] / delta x, which represents the instantaneous rate of change of a function at a given point.
How is the concept of slope related to derivatives?
-The concept of slope is directly related to derivatives because a derivative, at a given point, represents the slope of the tangent line to the graph of the function at that point.
What is the difference quotient?
-The difference quotient is the expression (f(x + delta x) - f(x)) / delta x, which is used to find the slope between two points on the graph of a function and is a key component in deriving the limit definition of a derivative.
What is the power rule of differentiation?
-The power rule of differentiation states that the derivative of x to the power of n, denoted as d/dx(x^n), is equal to n times x to the power of (n - 1), or nx^(n-1).
How does the binomial theorem help in proving the power rule?
-The binomial theorem helps in proving the power rule by allowing the expansion of (x + delta x)^n into a series of terms, which can then be simplified and analyzed as delta x approaches zero to show that the remaining term is nx^(n-1).
What is the significance of the term 'junk' in the context of the video?
-In the context of the video, 'junk' refers to the terms in the binomial expansion that contain more than one delta x and thus approach zero as delta x approaches zero. These terms do not contribute to the final derivative and can be ignored in the limit.
Why is it important to understand the limit definition of a derivative?
-Understanding the limit definition of a derivative is important because it provides a rigorous foundation for the concept of a derivative, showing how it represents the instantaneous rate of change of a function, which is fundamental to calculus.
How does the video use the concept of instantaneous change in slope?
-The video uses the concept of instantaneous change in slope to explain how as delta x becomes infinitesimally small, the average slope between two points approaches the slope of the function at a single point, which is the derivative at that point.
What does the video suggest for those who find the material confusing at first?
-The video suggests re-watching it to gain clarity, as the concepts involved in understanding the power rule and the limit definition of a derivative can be complex and may require multiple viewings for comprehension.
Why is the power rule considered a fundamental rule of calculus?
-The power rule is considered a fundamental rule of calculus because it provides a straightforward method for finding the derivatives of power functions, which are common in mathematical and real-world applications.

Outlines

00:00

📚 Introduction to Derivatives and the Power Rule

The video begins with an introduction to the power rule and the limit definition of a derivative. The presenter aims to provide a deep understanding of derivatives, not just their calculation. The limit definition of a derivative is presented, which is a foundational concept in calculus. The presenter uses a graph to illustrate the concept of a derivative as a slope and introduces the difference quotient, which is a preliminary step to proving the power rule.

05:01

🔍 Deriving the Difference Quotient and Tangent Line

The presenter continues by deriving the difference quotient, denoted as delta f over delta x, which represents the change in y (or f) over the change in x. Using the concept of a tangent line, the video explains how the slope of this line can be found using the difference quotient. The video emphasizes the importance of understanding the difference quotient as it is key to the limit definition of a derivative.

10:03

🧮 Applying the Binomial Theorem to Prove the Power Rule

The video then delves into proving the power rule using the limit definition of a derivative. The power rule states that the derivative of x to the power of n is nx^(n-1). To prove this, the presenter plugs the function x^n into the limit definition and applies the binomial theorem. A trick is introduced to ensure terms cancel out, leading to a generalizable form that applies to any power n.

15:04

🎓 Simplifying the Expression and Proving the Power Rule

The presenter simplifies the expression obtained from applying the binomial theorem, focusing on terms that do not vanish as delta x approaches zero. The 'junk' terms, which contain more than one delta x, are shown to approach zero and thus can be ignored. The video concludes with the derivation of the power rule, showing that the derivative of x to the power of n simplifies to nx^(n-1), thus proving the rule.

20:04

🏁 Conclusion and Encouragement for Further Understanding

The video concludes by emphasizing the importance of understanding the power rule, a fundamental concept in calculus. The presenter encourages viewers to re-watch the video for clarity, as the material may be confusing on the first attempt. The aim is to ensure viewers gain a solid understanding of not just the power rule, but also the underlying principles that make it work.

Mindmap

Keywords

💡Derivative

A derivative in calculus is a measure of how a function changes as its input changes. It is the slope of the line tangent to the graph of the function at a certain point. In the video, the concept of the derivative is central to understanding the power rule and the limit definition, with the aim to prove these mathematical principles.

💡Limit Definition

The limit definition of a derivative is a formal way to define the derivative using limits. It is expressed as the limit as 'delta x' approaches zero of [f(x + delta x) - f(x)] / delta x. The video explains this concept in detail, showing how it leads to the instantaneous rate of change of a function at a given point.

💡Power Rule

The power rule is a basic theorem in calculus that states the derivative of x raised to the power of n is n times x to the power of n minus one (d/dx(x^n) = nx^(n-1)). The video aims to prove this rule using the limit definition of a derivative, which is crucial for understanding the generalization of derivatives for power functions.

💡Slope

Slope in the context of the video refers to the steepness or gradient of a line, which in calculus is used to describe the rate of change of a function. The concept of slope is foundational to understanding derivatives, as it represents the instantaneous slope of the tangent line to a curve at a given point.

💡Delta (Δ)

In the video, 'delta' is used to represent a change in a variable, often written as 'delta x' (Δx) to denote a small change in the variable x. It is a key component in the limit definition and the difference quotient, illustrating the concept of an infinitesimally small change that leads to the definition of a derivative.

💡Difference Quotient

The difference quotient is a term used to describe the ratio of the difference in the dependent variable to the difference in the independent variable between two points on a function. It is represented as [f(x + Δx) - f(x)] / Δx. The video demonstrates how the difference quotient is used to derive the limit definition of a derivative.

💡Binomial Theorem

The binomial theorem is a mathematical formula that describes the expansion of powers of a binomial. In the video, it is used to expand the term (x + Δx)^n, which is crucial for the proof of the power rule. The theorem allows for the generalization of the power rule to any power n.

💡Instantaneous Rate of Change

The instantaneous rate of change refers to the derivative at a specific point, which is the limit of the average rate of change as the interval size approaches zero. The video emphasizes this concept to explain how the limit definition of a derivative captures the exact momentary rate at which a function is changing.

💡Binomial Expansion

Binomial expansion is the application of the binomial theorem to expand an expression of the form (a + b)^n. In the script, the binomial expansion is used to simplify the expression for the power rule proof, showing how each term of the expansion contributes to the final form of the derivative.

💡Factorial

A factorial, denoted by 'n!', is the product of all positive integers less than or equal to n. It is used within the binomial theorem to describe the coefficients of the terms in the expansion. The video script uses the concept of factorial to explain the terms of the binomial expansion when proving the power rule.

💡Generalization

Generalization in the context of the video refers to the process of proving a mathematical rule or theorem that applies to all possible cases, not just specific instances. The power rule is generalized for any power n, and the video demonstrates this through the use of the limit definition and binomial theorem.

Highlights

The video aims to provide a deep understanding of derivatives by proving the power rule and showcasing the limit definition of a derivative.

The limit definition of a derivative is introduced, which is a foundational concept in calculus.

The concept of slope as the rate of change is used to derive the limit definition of a derivative.

A graphical representation is used to illustrate the concept of slope and the tangent line to a curve at a point.

The difference quotient, denoted as delta f over delta x, is derived from the concept of slope.

The binomial theorem is applied to generalize the power rule for any exponent n.

An interesting trick using the binomial theorem ensures that most terms cancel out, leaving the general form of the power rule.

The video explains why terms with more than one delta x approach zero as delta x approaches zero.

The limit definition of a derivative is used to prove the power rule, showing that the derivative of x^n is nx^(n-1).

The video emphasizes the importance of understanding the underlying theory behind calculus rules rather than just memorizing them.

The presenter encourages re-watching the video for better comprehension, acknowledging the complexity of the topic.

The video concludes with a proof that reinforces the power rule as a fundamental principle of calculus.

The use of color and visual aids throughout the video helps to clarify complex mathematical concepts.

The presenter provides a step-by-step derivation of the power rule from the limit definition of a derivative.

The concept of instantaneous slope is explained as delta x approaches zero, providing insight into the limit definition.

The video uses the difference quotient to bridge the gap between the concept of slope and the limit definition of a derivative.

The binomial expansion is shown to be a key component in the proof of the power rule for derivatives.

The presenter emphasizes the generalizability of the power rule to any power n, highlighting its utility in calculus.

Transcripts

Browse More Related Video

Proof: d/dx(x^n) | Taking derivatives | Differential Calculus | Khan Academy

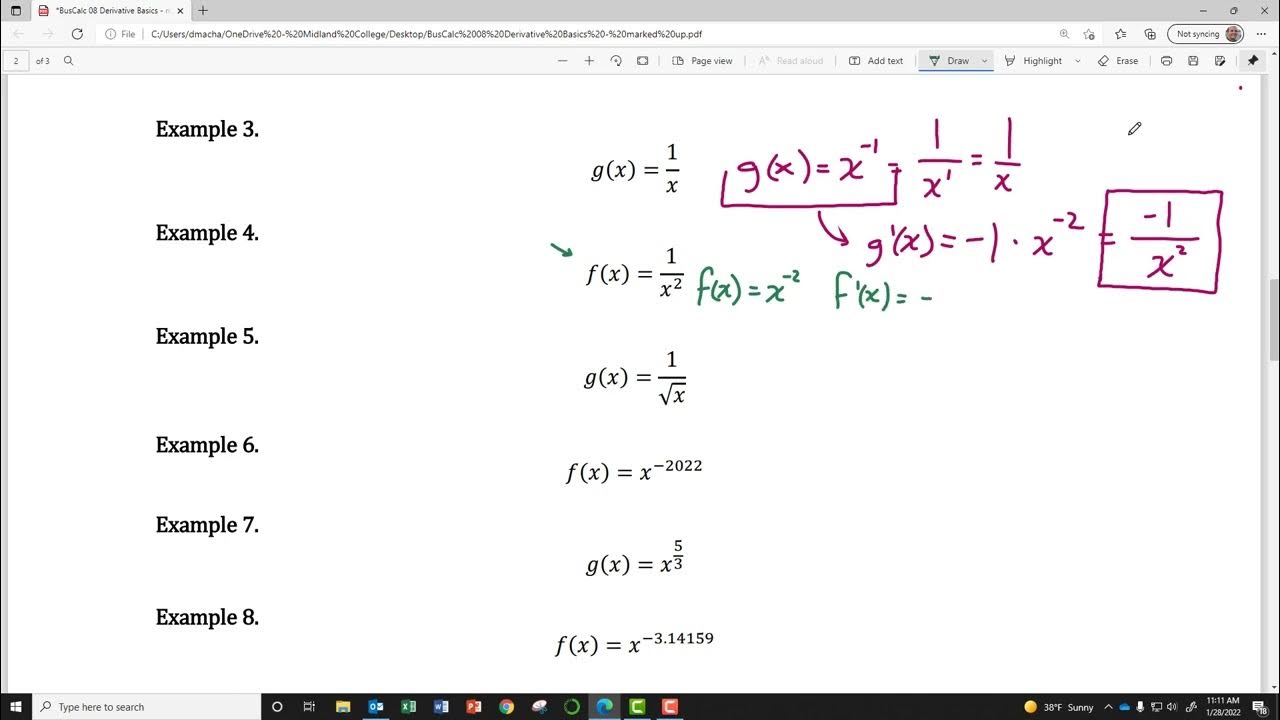
BusCalc 08 Derivative Basics

Basic Derivative Rules - Part 1

Power rule introduction (old) | Taking derivatives | Differential Calculus | Khan Academy

Proof: d/dx(sqrt(x)) | Taking derivatives | Differential Calculus | Khan Academy

DERIVATIVE OF RADICAL: THE CHAIN RULE

Related Tags

Calculus Derivatives Power Rule Limit Definition Math Education Binomial Theorem Slope Intercept Educational Video Math Proof Instantaneous Slope Binomial Expansion