Calculus AB/BC – 4.4 Introduction to Related Rates
TLDRIn this engaging lesson, Mr. Bean introduces the concept of related rates, using the Pythagorean theorem as a foundation. The video explores how variables relate to each other, particularly in dynamic situations such as a triangle's sides changing over time. Through examples involving a police car chase and an airplane approaching an observer, the lesson demonstrates setting up equations to find the related rates of change with respect to time. The focus is on understanding the relationship between variables and their rates of change, emphasizing the importance of considering constants and the chain rule in differentiation.
Takeaways
- 📚 Introduction to related rates, a concept in calculus that involves understanding how rates of change relate to each other.
- 🔄 The foundational concept is that variables often have relationships, like in the Pythagorean theorem, which can be static or dynamic.
- 📈 The change in variables can be represented as rates of change (derivatives) with respect to time, which are crucial in related rates problems.
- 🔽 The derivative with respect to time is denoted as d/dt, which is used to find the rate of change for different variables.
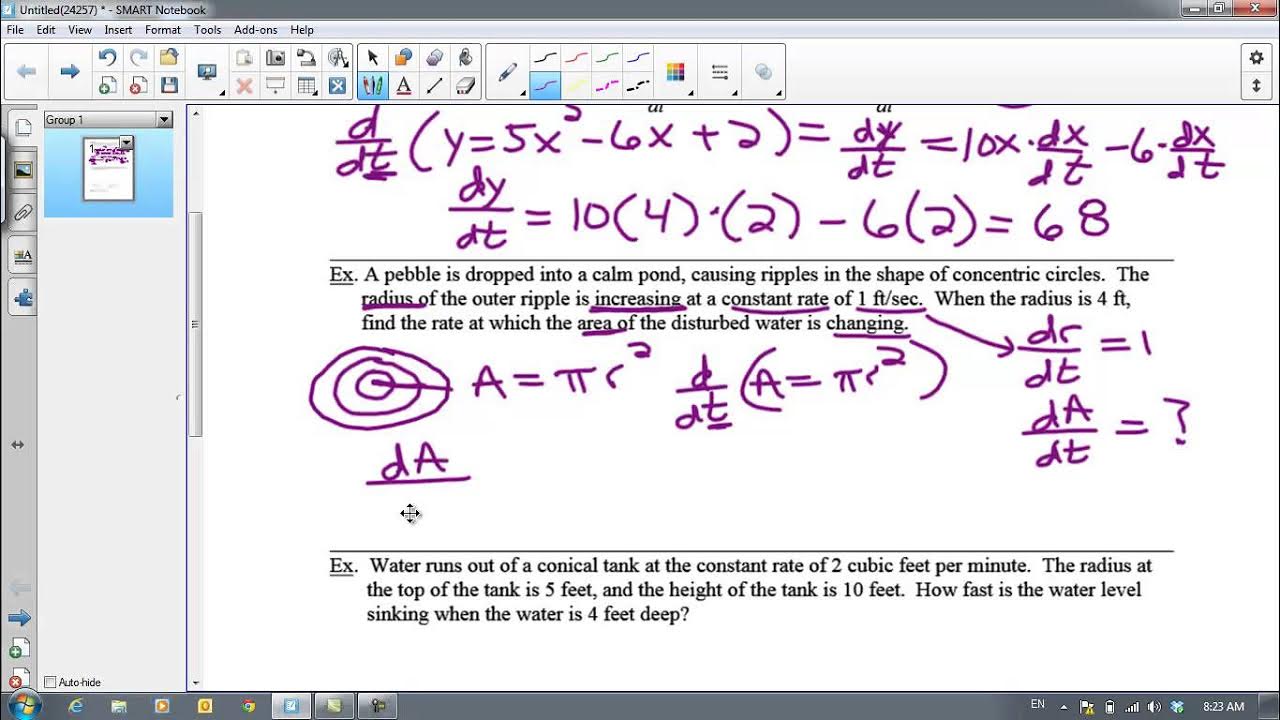
- 📐 An example used in the script is a right triangle where the base (b) and height (h) change over time, affecting the hypotenuse (c), demonstrating related rates.
- 🚓 A practical application discussed is a police car chasing a speeding car, where the related rates represent the speeds of both vehicles and their changing distance from each other.
- 🛫 Another example involves an airplane flying horizontally towards an observer, with the related rates representing the airplane's speed, the changing horizontal distance, and the constant altitude.
- 🧮 The derivative of a constant is zero, which simplifies the related rates equation when dealing with constants, such as the constant altitude of the airplane.
- 🔄 The process of solving related rates problems involves setting up an equation with variables, taking their derivatives with respect to time, and then analyzing how these rates of change are related.
- 📝 The script emphasizes the importance of understanding the relationships between variables and their rates of change, even without a visual representation.
- 🚀 The lesson aims to build an understanding of setting up related rates problems, with the intention of solving them in future lessons with actual numerical values.
Q & A
What is the main topic of this lesson?
-The main topic of this lesson is an introduction to related rates, which involves understanding how variables relate to each other in terms of their rates of change.
How does Mr. Bean introduce the concept of related rates?
-Mr. Bean introduces the concept of related rates by using the example of a right triangle and its changing dimensions, illustrating how the rates of change for each side are related to each other, similar to how the variables in the Pythagorean theorem are related.
What is the significance of the Pythagorean theorem in this lesson?
-The Pythagorean theorem is used as a basis to explain related rates. It shows the relationship between the sides of a right triangle, and this relationship is used to demonstrate how the rates of change of these sides can be related even when the triangle's dimensions are changing dynamically.
How does the lesson connect the concept of related rates to real-life scenarios?
-The lesson connects related rates to real-life scenarios by presenting a situation involving a police car chasing a speeding car and an airplane flying towards an observer. These examples help to illustrate how related rates can be applied to understand and solve problems involving motion and change over time.
What is the role of derivatives in related rates problems?
-Derivatives play a crucial role in related rates problems as they represent the rate of change of a function with respect to time. By taking the derivative of an equation that relates variables, we can find the rates at which these variables are changing and understand how these rates are related to each other.
How does the lesson handle the concept of constants in related rates?
-The lesson explains that the derivative of a constant is zero. This is demonstrated in the example of the airplane flying at a constant altitude, where the rate of change of the altitude (y) with respect to time is zero, simplifying the related rates equation.
What is the purpose of the practice problems mentioned in the lesson?
-The purpose of the practice problems is to help students apply the concepts learned in the lesson to various scenarios, enhancing their understanding of related rates and how to set up and solve problems involving rates of change.
How does the lesson emphasize the importance of understanding related rates?
-The lesson emphasizes the importance of understanding related rates by showing how it can be used to analyze and solve problems involving dynamic changes, such as the motion of vehicles and the approach of an airplane towards an observer.
What is the main takeaway from this lesson?
-The main takeaway from this lesson is the understanding of how to set up equations to relate variables in a dynamic situation, take their derivatives with respect to time, and analyze how these rates of change are interconnected, which is the essence of related rates problems.
What advice does Mr. Bean give for students who may struggle with the concepts?
-Mr. Bean advises students who may struggle with the concepts to practice and, if needed, move on to corrective assignments on the website to get more practice and solidify their understanding of related rates.
Outlines
📚 Introduction to Related Rates
This paragraph introduces the concept of related rates, a topic in calculus that deals with the relationship between varying quantities. Mr. Bean explains that understanding related rates involves recognizing how different variables are interconnected, often through familiar relationships such as the Pythagorean theorem. He uses the example of a triangle whose sides are changing dynamically to illustrate the concept of rates of change. Mr. Bean emphasizes that related rates are the derivatives of these variables with respect to time, and they maintain a relationship as defined by the Pythagorean theorem. The paragraph sets the stage for further exploration of related rates through examples and practice problems.
🚓 Applying Related Rates to a Police Chase
In this paragraph, the application of related rates is demonstrated through a hypothetical police chase scenario. The speaker labels the horizontal distance as 'x', the vertical distance as 'y', and the hypotenuse (direct line of sight) as 'z'. By applying the Pythagorean theorem (x^2 + y^2 = z^2) and taking derivatives with respect to time, the speaker establishes a relationship between the rates of change of these distances. The derivatives dx/dt, dy/dt, and dz/dt represent the rates of change for the escaping car, the police car, and the distance between the two cars, respectively. The paragraph concludes by emphasizing the importance of understanding these relationships and applying them to real-world problems without relying on visual aids.
Mindmap
Keywords
💡Related Rates
💡Pythagorean Theorem
💡Derivative
💡Implicit Differentiation
💡Chain Rule
💡Rate of Change
💡Dynamic
💡Constants
💡Horizontal and Vertical
💡Algebraic Setup
Highlights
Introduction to related rates in calculus, a concept that explores how variables relate to each other through rates of change.
Using the Pythagorean theorem as a basis for understanding relationships between variables in a dynamic context.
The concept of a triangle's sides changing dynamically, with one side's length remaining constant while the base extends.
Explaining related rates as the distinct rates of change assigned to different dimensions that are interconnected.
Derivatives and their role in calculating rates of change with respect to time, emphasizing the chain rule.
Illustration of how the rate of change of the base and hypotenuse in a triangle are related rates.
Setting up an equation using the Pythagorean theorem to relate the changing sides of a triangle with respect to time.
Application of related rates in a practical scenario involving a police car chasing a speeding car.
Deriving the related rates equation for the police car and speeding car scenario using the Pythagorean theorem.
Explanation of how the rate of change can indicate whether the police car is getting closer to or further away from the speeding car.
Introduction of a second example involving an airplane approaching an observer on the ground.
Use of the Pythagorean theorem to establish the relationship between the horizontal and vertical distances and the straight-line distance to the observer.
Accounting for the constant altitude of the airplane and its impact on the related rates equation.
Deriving the relationship between the airplane's speed and the rate at which it is approaching the observer.
Emphasizing the importance of understanding related rates without relying on visual diagrams.
The lesson's focus on setting up the variables in a relationship and taking their derivatives to understand how their rates interrelate.
Encouragement for further practice and the availability of corrective assignments for additional understanding.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: