Fall 2023 MNC: Motion "wellness" - velocity, speed, acceleration, distance, position

The video begins with Curtis Brown welcoming viewers to another session of Monday Night Calculus. He introduces his guests, Steve Kakasa and Tom Dick, and expresses excitement about their expertise. The topic for the evening is motion, and Curtis encourages teachers and students to participate actively by posting comments and questions in the chat. He also mentions that he will share his email for professional development hours and post the answer document after the session. Curtis apologizes for not posting questions beforehand and attributes the delay to issues with Texas Instruments. The session starts with a question posed by Steve about the problems they will be discussing, highlighting the importance of homework and participation.

In this segment, Tom uses technology to explore the motion of a particle with a given velocity function. He explains the process of graphing the velocity function and interpreting its graph to find when the particle is moving to the left. Tom demonstrates how to use a calculator to find the intervals when the velocity is negative, indicating backward motion. He also discusses calculating the acceleration by taking the derivative of the velocity function and uses the calculator to find the acceleration at a specific time. The focus is on using technology to solve problems related to motion, which is a common type of question in exams.

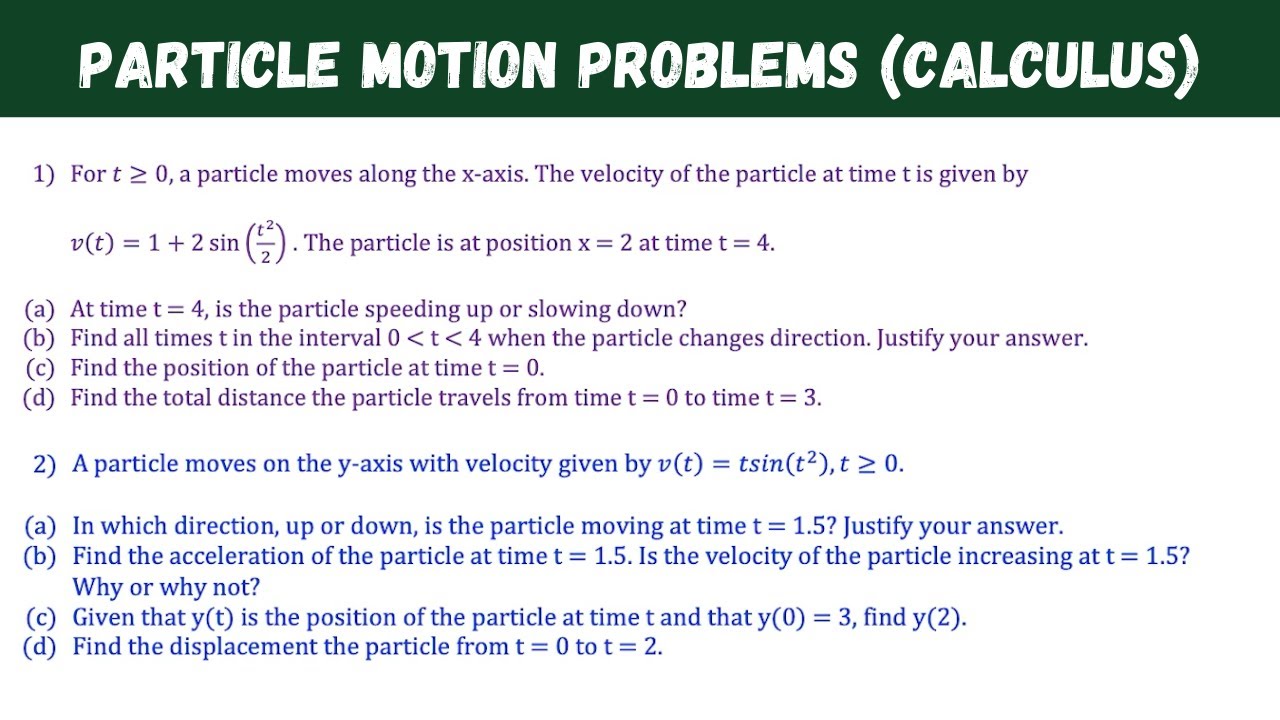
Steve takes over to analyze the particle motion more analytically. He revisits the velocity function and its zeros, using mathematical techniques to find the intervals when the particle moves to the left. Steve creates a sign chart to understand the velocity's behavior throughout the given time interval. He then moves on to find the position of the particle at specific times using integration, demonstrating the fundamental theorem of calculus. The segment emphasizes the importance of understanding both the graphical and analytical approaches to solving calculus problems related to motion.

This paragraph focuses on understanding the difference between distance traveled and displacement. The discussion revolves around a specific problem where the distance between the particle's positions at two different times is to be found. Curtis and Steve explore the concepts of absolute value in the context of displacement and distance traveled. They highlight the significance of recognizing when to use absolute values in various types of problems. The segment also touches on the importance of understanding the physical interpretation of mathematical expressions in motion problems.

The discussion shifts to a real-world problem involving a hot air balloon ride at a fair. The balloon's velocity is given by a piecewise linear function, and the task is to analyze its motion over time. The participants determine when the balloon changes direction by examining the velocity graph. They calculate the height of the balloon at specific times by interpreting the area under the velocity curve as the change in position. The segment also addresses finding the total distance traveled by the balloon and determining whether the balloon is speeding up or slowing down at a particular time. The problem encapsulates the application of calculus concepts to a practical scenario.

The session concludes with a series of challenging overtime problems that test the participants' calculus knowledge. These problems involve complex integrals and the application of substitution methods. The participants demonstrate clever use of calculus techniques to solve these problems, showcasing their deep understanding of the subject. The session ends with Curtis thanking Steve and Tom for their contributions and encouraging teachers and students to join future sessions. He also mentions the upcoming topics and reminds viewers to reach out for professional development certificates.