Proof of fundamental theorem of calculus | AP Calculus AB | Khan Academy
TLDRThe video script presents a detailed explanation of the fundamental theorem of calculus, demonstrating the connection between continuous functions and their antiderivatives. It illustrates the concept through the visualization of a function f(t) on an interval and introduces the function F(x) as the definite integral from 'a' to 'x' of f(t) dt. The script then rigorously proves that the derivative of F(x) is equal to f(x), highlighting the mean value theorem of definite integrals and the squeeze theorem in the process. This proof underscores the existence of an antiderivative for any continuous function, thereby unifying differential and integral calculus.
Takeaways
- 📈 The concept of a function being continuous on an interval is introduced, specifically from 'a' to 'b'.
- 🎓 The visualization of a continuous function is explained using the graph of y = f(t).
- 📊 A new function F(x) is defined as the definite integral from 'a' to 'x' of f(t) dt, representing the area under the curve up to point 'x'.
- 🌟 The importance of understanding the definite integral as an area, not just as a concept related to differentiation and antiderivatives, is emphasized.
- 🔄 The process of differentiating the function f(x) using the definition of derivatives is outlined.
- 🤔 The connection between the derivative of F(x) and the mean value theorem of definite integrals is explored.
- 🏆 The mean value theorem states that there exists a 'c' in the interval [x, x + delta x] such that f(c) equals the mean height of the curve over that interval.
- 📌 The proof that the derivative of F(x) is equal to f(x) itself is presented, showing the link between the integral and the derivative.
- 🌐 The fundamental theorem of calculus is highlighted as it connects the ideas of differential and integral calculus, showing that every continuous function has an antiderivative.
- 💡 The significance of the fundamental theorem of calculus is discussed, noting its role in unifying the concepts of calculus and providing a powerful tool for further mathematical analysis.
Q & A
What is the main concept discussed in the script?
-The main concept discussed in the script is the fundamental theorem of calculus, which connects the ideas of differentiation and integration, and establishes that every continuous function has an antiderivative.
How does the script visualize the function f(t)?
-The script visualizes the function f(t) as a graph on the t-axis, with the interval from 'a' to 'b' representing the domain of continuity for the function.
What is the function F(x) defined as in the script?
-The function F(x) is defined as the definite integral from 'a' to 'x' of f(t) dt, where 'x' is within the interval from 'a' to 'b'.
What does the area under the curve of f(t) represent?
-The area under the curve of f(t) represents the value of the function F(x), which is the definite integral from 'a' to 'x'.
How is the derivative of F(x) related to the function f(t)?
-The derivative of F(x), denoted as F'(x), is equal to f(x). This is a key result from the fundamental theorem of calculus, showing the direct relationship between the derivative of an antiderivative and the original function.
What is the mean value theorem of definite integrals mentioned in the script?
-The mean value theorem of definite integrals states that there exists a 'c' in the interval from 'x' to 'x + delta x' such that the function evaluated at 'c' times the interval length (delta x) is equal to the definite integral from 'x' to 'x + delta x' of f(t) dt.
How does the script use the mean value theorem to derive the relationship between F'(x) and f(x)?
-The script uses the mean value theorem to show that the derivative of F(x), F'(x), is equal to the function evaluated at some 'c' in the interval from 'x' to 'x + delta x'. As delta x approaches 0, 'c' also approaches 'x', and thus F'(x) is equal to f(x).
What is the significance of the fundamental theorem of calculus in the field of calculus?
-The fundamental theorem of calculus is significant because it establishes the connection between differential calculus (study of derivatives) and integral calculus (study of integrals). It shows that every continuous function has an antiderivative, which is a powerful unifying concept in calculus.
How does the script explain the concept of an antiderivative?
-The script explains the concept of an antiderivative by defining a function F(x) as the area under the curve of f(t) from 'a' to 'x'. It then proves that the derivative of this function F(x) is equal to the original function f(t), thereby showing that F(x) is an antiderivative of f(t).
What is the role of the squeeze theorem in the script's explanation?
-The squeeze theorem is used to rigorously prove that as delta x approaches 0, the value of 'c' (which comes from the mean value theorem) also approaches 'x'. This helps to confirm that the limit of F'(x) as delta x approaches 0 is indeed f(x), solidifying the relationship between the derivative and the antiderivative.
How does the script demonstrate the transition from viewing integrals solely as areas to understanding their connection to antiderivatives?
-The script begins by discussing integrals as areas under the curve and then through the process of defining a function F(x) and taking its derivative, it shows that the derivative of F(x) is the original function f(t). This transition demonstrates that integrals are not just about calculating areas but are also connected to the process of finding antiderivatives.
Outlines
📈 Introduction to the Concept of Continuity and the Function f
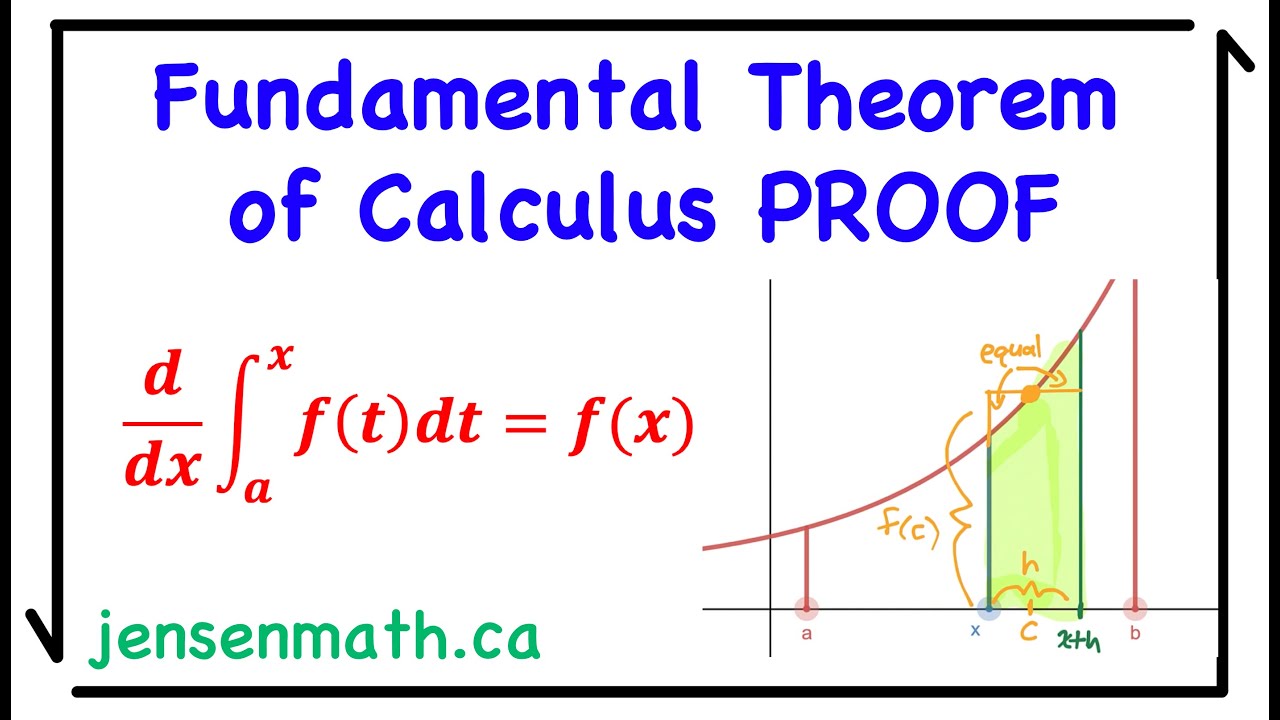
The paragraph introduces a continuous function, f, defined on the interval a to b. It discusses visualizing this function on a graph with the y-axis relabeled as the t-axis. The concept of the function F(x) is introduced as the definite integral from a to x of f(t) dt, representing the area under the curve of f from a to x. The paragraph sets the stage for exploring the relationship between this integral and derivatives, despite the fact that the connection is not yet known. It also begins the process of differentiating the function f using the definition of derivatives, leading to an expression involving the difference in the area under the curve as x changes.
📊 Derivation of the Mean Value Theorem and its Implications
This paragraph delves into the process of differentiating the function F(x) and how it relates to the Mean Value Theorem of definite integrals. It explains the expression for the derivative of F(x) as a limit involving the difference in areas under the curve. The Mean Value Theorem is introduced, stating that there exists a c in the interval (x, x+delta x) such that the function evaluated at c is equal to the mean height of the curve over that interval. This theorem is key to understanding the relationship between the integral and the derivative of a continuous function.
🎓 Proof of the Fundamental Theorem of Calculus and its Significance
The final paragraph provides a rigorous proof that the derivative of F(x) is equal to the original function f(x), using the concept of limits and the Squeeze Theorem. It establishes that as delta x approaches 0, the value of c, which represents a point in the interval (x, x+delta x), also approaches x. This leads to the conclusion that the derivative of F(x) is indeed f(x), affirming the Fundamental Theorem of Calculus. The theorem's importance is emphasized as it connects the concepts of differential calculus (derivatives) with integral calculus (antiderivatives), showing that every continuous function has an antiderivative and highlighting the power of this connection in the field of calculus.
Mindmap
Keywords
💡continuous function
💡interval
💡derivative
💡antiderivative
💡area under the curve
💡mean value theorem
💡limit
💡squeeze theorem
💡fundamental theorem of calculus
💡integration
💡mean height
Highlights
The discussion begins with a continuous function f on the interval a to b, providing a foundation for the exploration of visualization and mathematical concepts.
The introduction of the t-axis as a means to represent the variable in the context of continuity is a key step in setting up the problem.
The graph of y equals f of t is described as continuous on the interval from a to b, emphasizing the importance of the function's behavior across this range.
The function F of x is defined using a definite integral from a to x of f of t dt, which is a significant step in connecting integral calculus to the concept of antiderivatives.
The area under the curve f between a and x is equated to F of x, providing a geometric interpretation of the integral.
The attempt to prove a connection between the definite integral and antiderivatives is highlighted, setting the stage for the main theorem's exploration.
The derivative of f is taken using the definition of derivatives, which is a critical step in applying calculus concepts to the problem.
The expression for the derivative f prime of x is developed, leading to a deeper understanding of the relationship between the function and its derivative.
The mean value theorem of definite integrals is introduced as a tool to connect the function evaluated at c to the area under the curve.
The existence of a c in the interval x to x plus delta x is established, which is crucial for the proof of the fundamental theorem of calculus.
The approach of c to x as delta x approaches 0 is discussed, which is a key insight in the proof.
The use of the squeeze theorem to rigorously show that c approaches x as delta x approaches 0 is an important methodological step.
The conclusion that the derivative of the function F of x is equal to the continuous function f of x is reached, marking the successful completion of the proof.
The fundamental theorem of calculus is introduced, highlighting its significance in tying together differential and integral calculus.
The proof demonstrates that any continuous function has an antiderivative, which is a powerful result with broad implications for calculus.
The integral is connected to the process of taking a derivative, which is a profound insight into the nature of calculus.
The discussion concludes with the recognition of the fundamental theorem of calculus as a keystone in the field, providing a deep and unifying principle.
Transcripts
Browse More Related Video

Fundamental theorem of calculus (Part 2) | AP Calculus AB | Khan Academy

The Fundamental Theorem of Calculus - Proof
1st Fundamental Theorem of Calculus PROOF | Calculus 1 | jensenmath.ca
Fundamental Theorem of Calculus II
Worked example: Finding derivative with fundamental theorem of calculus | Khan Academy
Applying the Second Fundamental Theorem | MIT 18.01SC Single Variable Calculus, Fall 2010
5.0 / 5 (0 votes)
Thanks for rating: