Introduction to rate-of-change problems
TLDRThe video script delves into the application of derivatives, specifically the chain rule, to solve real-world problems. It begins with a review of the chain rule, presented in a slightly unconventional format for clarity. The script then explores a scenario involving a cone filled with water, using the chain rule to determine the rate at which the water level rises when water is poured in at a constant rate. The problem is solved by differentiating the volume equation with respect to time, resulting in a fascinating insight into the rate of change of the water level. The explanation is engaging and encourages viewers to understand the power of calculus in solving practical problems.
Takeaways
- 📚 The script begins with a review of the chain rule, a fundamental concept in calculus.
- 🔄 The chain rule is presented in a slightly different way, emphasizing the rate of change of one function with respect to another.
- 🎨 The讲师 changes colors for emphasis and variety, but the core concept remains the same.
- 👌 Two equivalent ways of writing the chain rule are explained, providing a deeper understanding of the concept.
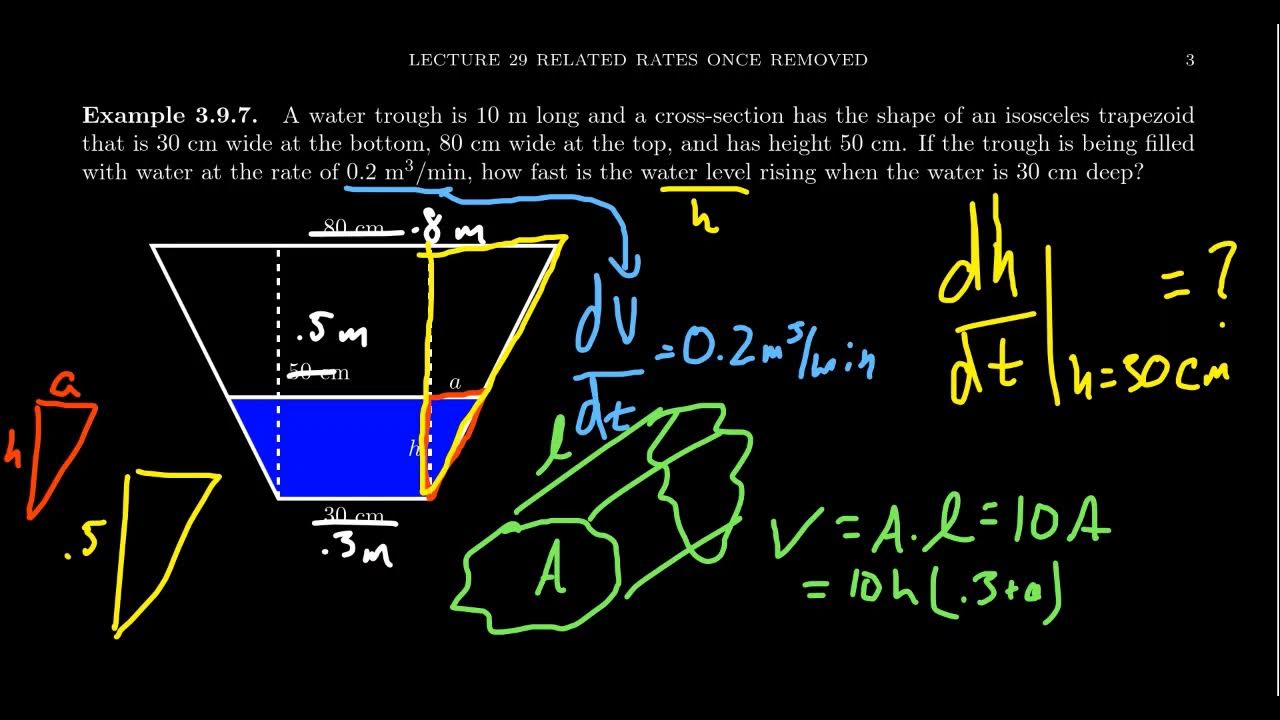
- 🏺 The example of a cone is used to demonstrate the application of the chain rule in solving real-world problems.
- 📏 The ratio of the radius to the height of the cone is constant, with the radius being 1/2 the height.
- 💧 The volume of a cone is given by the formula V = 1/3 * base area * height, where the base area is πr².
- 📈 The讲师 introduces a scenario of pouring water into a cone at a rate of 1 cubic centimeter per second and seeks to find the rate at which the water level rises.
- 🧠 The讲师 applies the chain rule to relate the rate of change of volume with respect to time (dv/dt) to the rate of change of height with respect to time (dh/dt).
- 🔢 By setting dv/dt to 1 and knowing the height (h = 2 cm), the讲师 calculates the rate of change of height with respect to time as 1/π cm/s.
- 🤔 The讲师 acknowledges the complexity of the problem and suggests that viewers may need to rewatch the video for better understanding.
Q & A
What is the chain rule in calculus?
-The chain rule is a fundamental rule in calculus that is used to find the derivative of a composite function. It states that the derivative of a function f(g(x)) is equal to the derivative of f with respect to g, multiplied by the derivative of g with respect to x.
How is the chain rule represented in the script?
-In the script, the chain rule is represented as df/dg * dg/dx, which means the rate at which f changes with respect to g times the rate at which g changes with respect to x.
What is the volume of a cone?
-The volume of a cone is given by the formula V = (1/3) * base area * height. The base area is pi times the radius squared (A = πr^2), so the volume formula can also be written as V = (1/3) * π * r^2 * h.
How does the ratio of the radius to the height change in the cone described in the script?
-In the cone described in the script, the ratio of the radius to the height remains constant. At any point, the radius is 1/2 of the height.
What is the relationship between the volume of water in the cone and the height in the given example?
-In the given example, the volume of water in the cone is (1/12) * π * h^3, where h is the height of the water.
How fast is the water level rising when h is equal to 2 centimeters?
-When h is equal to 2 centimeters, the rate at which the water level is rising, denoted as dh/dt, is 1/π centimeters per second.
What is the significance of the rate of change in this context?
-The rate of change in this context, dv/dt, represents how quickly the volume of water in the cone is increasing with respect to time. It is used to determine the speed at which the water level is rising.
What is the relationship between the rate of change of volume with respect to time and the rate of change of height with respect to time?
-The relationship is given by the equation (1/3) * π * h^2 * dv/dt = dv/dt. This equation shows that the rate of change of volume with respect to time is equal to the volume changing rate times the square of the height times the rate of change of height with respect to time.
How is the volume of water related to the height in the cone?
-The volume of water in the cone is directly proportional to the square of the height. This is evident from the volume formula V = (1/3) * π * (1/2h)^2 * h, which simplifies to V = (1/12) * π * h^3.
What is the rate of pouring water into the cup as described in the script?
-The water is being poured into the cup at a rate of 1 cubic centimeter per second, which is equivalent to 1 millimeter per second for the purposes of this problem.
How can the chain rule be applied to solve real-world problems like the one in the script?
-The chain rule can be applied to solve real-world problems involving rates of change, such as determining how fast a certain quantity is changing in relation to another quantity. In the script, it was used to find out the rate at which the water level in a cone is rising based on the volume of water poured in per second.
What is the final equation derived to find the rate at which the height of the water is changing with respect to time?
-The final equation derived to find the rate at which the height of the water is changing with respect to time is 1 = π * dh/dt, which simplifies to dh/dt = 1/π centimeters per second when h is 2 centimeters.
Outlines
📚 Introduction to Derivatives and Chain Rule
This paragraph begins with a review of the chain rule, a fundamental concept in calculus. The speaker introduces a new way of writing the chain rule, which might seem unfamiliar at first but is essentially the same as the traditional format. The explanation includes the derivative of a composite function, f(g(x)), and how it relates to the rates of change of the functions involved. The speaker also provides a practical example involving a cone and the volume of water it can hold, setting the stage for applying calculus to solve real-world problems.
💧 Calculating the Rate of Change in Volume and Height
In this paragraph, the speaker delves into the application of calculus to determine the rate at which the water level rises in a cone. The discussion involves the metric system and the conversion between different units of measurement. The main focus is on using the chain rule to relate the rate of change of volume with respect to time (dv/dt) to the rate of change of height with respect to time (dh/dt). The speaker solves for the rate of change of height when water is poured into the cone at a constant rate, providing a clear example of how calculus can be used to solve problems involving rates of change.
Mindmap
Keywords
💡Derivatives
💡Chain Rule
💡Rate of Change
💡Volume
💡Cone
💡Solid Geometry
💡Function
💡Integration
💡Height
💡Water Level
💡Pi (π)
Highlights
The introduction of using derivatives to solve practical problems.
A review of the chain rule presented in a new way.
Derivative of f(g(x)) expressed as the rate of change of f with respect to g, multiplied by the rate of change of g with respect to x.
The traditional chain rule format using f'(g(x)) and g'(x).
The mention of historical mathematicians, possibly Lagrange or Leibniz, related to the notation used.
The application of the chain rule to a geometric problem involving a cone.
The volume of a cone is given by 1/3 times the base area times the height.
The base area of the cone is π times the square of the radius, with the radius being 1/2 of the height.
The volume of water in the cone is calculated as 1/12π times the height cubed.
The problem of finding the rate at which the water level is rising in the cone.
The derivative of the volume with respect to time is taken to find the rate of change of the water level.
The rate of change of the volume with respect to height is expressed using the chain rule.
The height of the water in the cone is 2 centimeters, and the rate of change of volume with respect to time is 1 cubic centimeter per second.
The calculation of the rate at which the height of the water is changing with respect to time, which is 1 over π centimeters per second.
The acknowledgment that the problem might be confusing and the suggestion to review the material for better understanding.
The promise of more videos on rates of change problems to help with understanding.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: