Thinking outside the 10-dimensional box
TLDRThe video script explores the interplay between geometric and analytic reasoning in mathematics, highlighting the beauty of visualizing complex concepts in two and three dimensions. It discusses the challenge of extending this intuitive understanding to higher dimensions, where geometric insights become less accessible. The speaker introduces a 'slider' analogy to conceptualize higher-dimensional spaces, using the example of a four-dimensional sphere and a classic puzzle involving circles and higher-dimensional analogs to illustrate the counterintuitive nature of inner spheres' sizes in higher dimensions. The script emphasizes the value of visualizing mathematical concepts, even when they cannot be directly seen, and encourages a deeper engagement with high-dimensional geometry.
Takeaways
- 🌟 The beauty of math lies in the interplay between numbers and geometry, especially in two and three dimensions where our visual cortex can process spatial relationships more intuitively.
- 🔍 The usefulness of geometric reasoning is that it can clarify facts that seem opaque in purely analytic contexts, and vice versa.
- 💡 The connection between sums of squares and circles/spheres is central to many mathematical insights, including those involving pi, number theory, primes, and Pythagorean triples.
- 🌐 The challenge in higher dimensions is that our physical constraints limit our geometric intuition, making it difficult to visualize and reason about spaces with more than three dimensions.
- 🛫 Analogical reasoning using two and three dimensions can only take us so far; for higher dimensions, we must rely more on analytical methods, akin to a pilot using instruments rather than sight.
- 📈 The 'real estate' analogy helps in understanding the trade-offs between different variables in geometric configurations, where 'real estate' represents the squared values of coordinates.
- 📊 In higher dimensions, the concept of 'real estate' remains the same, but the global view that spatial visualization offers is lost, requiring a more abstract approach.
- 🔢 The script introduces a method for visualizing high-dimensional spaces using sliders to represent each coordinate, allowing for a more intuitive understanding of movements and relationships on spheres in higher dimensions.
- 🧠 The phenomenon of the inner sphere's radius increasing with higher dimensions is demonstrated, showing that in four dimensions, the inner sphere is the same size as the corner spheres, but in higher dimensions, the inner sphere becomes larger.
- 🕶️ The slider method and the concept of 'real estate' provide a more concrete way to think about high-dimensional shapes, despite the limitations of not being able to visualize these spaces fully.
- 🚀 The exploration of higher dimensions through this script encourages a shift in perspective, moving from skepticism about the existence of high-dimensional spaces to curiosity about their properties and characteristics.
Q & A
How does the concept of 'real estate' in the script relate to the geometric understanding of circles and spheres?
-The 'real estate' concept is used as an analogy to describe the distribution of values (squared) among the coordinates in a geometric space. It helps to visualize how the values of x, y, or more coordinates trade off against each other, similar to how different parties might share a piece of real estate. This analogy aids in understanding the geometric properties of shapes like circles and spheres in higher dimensions.
What is the significance of the slider representation in the script for understanding high-dimensional spaces?
-The slider representation provides a tangible way to conceptualize movements and relationships in high-dimensional spaces. Each slider represents a coordinate in a multi-dimensional space, and their collective values determine a point in that space. This method allows for a more intuitive grasp of high-dimensional geometry, despite the inherent challenge of visualizing such spaces.
How does the script explain the relationship between the radius of a circle and the coordinates in a two-dimensional space?
-The script explains that the radius of a circle in a two-dimensional space can be understood by considering the 'real estate' belonging to each coordinate (x and y). The total 'real estate' is one unit, and the values of x and y squared determine the position on the circle. This relationship is visualized as a trade-off between the values, where changes in one coordinate lead to compensatory changes in the other to maintain the circle's property.
What is the role of the Pythagorean theorem in the script's explanation of higher-dimensional geometry?
-The Pythagorean theorem is used to demonstrate how distances are calculated in higher-dimensional spaces. It shows that the distance from a point to the origin in a d-dimensional space is the square root of the sum of the squares of each coordinate. This principle is applied to find the radius of the inner sphere in various dimensional boxes, highlighting the counterintuitive nature of higher dimensions.
How does the script use the concept of 'real estate exchange' to explain the behavior of coordinates on a circle?
-The script describes the 'real estate exchange' as a way to visualize how the squared values of coordinates (x and y) trade off against each other on a circle. As one coordinate moves closer to zero, it 'gives up' its 'real estate,' allowing the other coordinate to 'gain' more, thus moving further away from zero. This exchange is what allows for movement along the circle and helps to understand the geometric properties in a more intuitive way.
What surprising result does the script reveal about the radius of the inner sphere in higher dimensions?
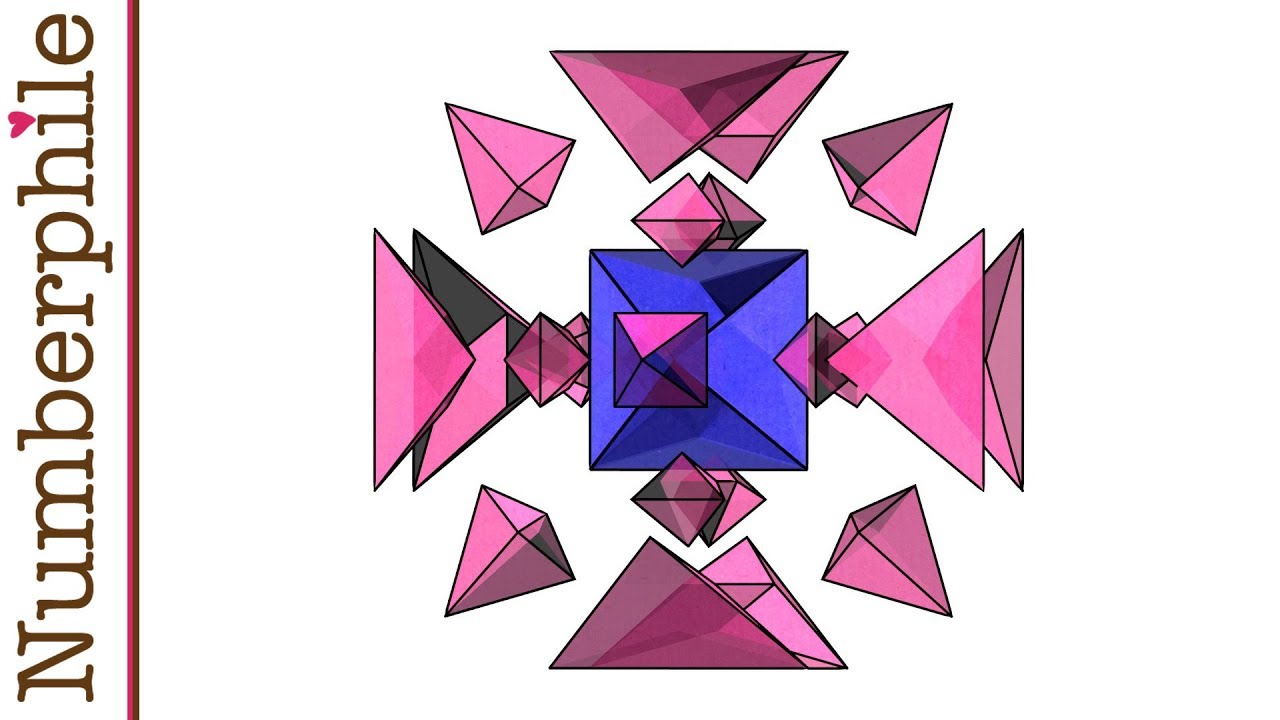
-The script reveals that as dimensions increase, the radius of the inner sphere also increases in a surprising way. In two and three dimensions, the inner sphere is smaller than the corner spheres, but in four dimensions, the inner sphere has the same radius as the corner spheres. As dimensions continue to increase, the inner sphere's radius grows without bound, even poking outside the bounding box in ten dimensions, which is a counterintuitive result.
How does the script connect the concept of 'real estate' to the Pythagorean theorem in higher dimensions?
-The script connects the concept of 'real estate' to the Pythagorean theorem by explaining that the total 'real estate' among all coordinates is equivalent to the square of the distance from the origin in a higher-dimensional space. This connection allows for a more intuitive understanding of how distances and radii are calculated in these spaces.
What is the significance of the 2x2 box and its inner circle in the script's discussion on higher dimensions?
-The 2x2 box and its inner circle serve as a practical example to illustrate the counterintuitive properties of higher dimensions. By examining the radius of the inner circle in relation to the corner circles, the script demonstrates how the behavior of these radii changes as dimensions increase, leading to surprising results in higher-dimensional analogs.
How does the script use the analogy of a bug on a sphere to describe the limitations of our understanding in high dimensions?
-The script uses the analogy of a bug on a sphere to convey the idea that just as a bug on a sphere's surface cannot see the entire sphere at once, we also struggle to visualize and understand high-dimensional spaces in their entirety. We can only grasp local movements and properties, which reflects the challenge of comprehending the global structure of high-dimensional objects.
What is the main goal of the slider method and 'real estate' analogy as presented in the script?
-The main goal of the slider method and 'real estate' analogy is to provide a more intuitive and accessible way to understand and think about high-dimensional geometry. By using these analogies, the script aims to make complex mathematical concepts more relatable and to help learners develop a better intuitive grasp of higher-dimensional spaces.
How does the script address the challenge of visualizing high-dimensional spaces?
-The script acknowledges the challenge of visualizing high-dimensional spaces and instead offers the slider method as a hybrid approach that combines geometric and analytic reasoning. This method allows for a more concrete understanding by representing each coordinate with a slider and using the 'real estate' analogy to visualize the relationships between coordinates in higher dimensions.
Outlines
📐 The Beauty of Geometric Reasoning in Math
This paragraph discusses the allure of mathematics through the lens of geometric reasoning. It highlights the intuitive nature of math when it involves the interplay between numbers and spatial concepts, such as understanding a circle in two and three dimensions. The paragraph emphasizes the channel's focus on connecting seemingly disparate ideas, particularly the relationship between sums of squares and circles/spheres, and how these connections are central to understanding higher mathematical concepts. It also touches on the limitations of our spatial intuition when dealing with higher dimensions, suggesting a need for a hybrid approach that combines geometric and analytic methods to enhance understanding.
🏠 Real Estate Analogy for Understanding Circles
The paragraph introduces a real estate analogy to conceptualize the relationship between x squared and y squared in the context of a circle. It explains how the value of x squared can be seen as the 'real estate' belonging to x, with y squared similarly belonging to y. The analogy is used to illustrate the exchange of real estate between x and y as one moves around the circle, with the piston-like dance motion of the sliders representing this exchange. The paragraph also discusses the addition of tick marks to visualize the trade-off in value between x squared and y squared, and how this relates to the distance from the origin in a unit sphere in three dimensions.
🔄 The Intuitive Slide to Higher Dimensions
This paragraph delves into the concept of extending the real estate analogy to higher dimensions, specifically focusing on the 4D sphere. It describes the process of visualizing a 4D unit sphere and the challenges associated with understanding higher-dimensional spaces. The paragraph introduces the idea of using vertical number lines with sliders to represent each dimension, with the goal of understanding how movements of these sliders correspond to movements on the sphere. It also touches on the concept of 'real estate exchange' in higher dimensions and how it mirrors the two-dimensional case, providing a foundational understanding for tackling high-dimensional puzzles.
🧩 The Counterintuitive Inner Circle Radius
The paragraph presents a classic example that highlights the counterintuitive nature of higher dimensions, focusing on the radius of an inner circle tangent to the corners of a 2x2 square in two dimensions and extending this to higher dimensions. It explains the process of finding the radius of the inner circle in two and three dimensions using the Pythagorean theorem and the concept of real estate exchange. The paragraph then introduces the idea of using sliders to analyze the two and three-dimensional cases, setting the stage for understanding the behavior of the inner circle radius in higher dimensions.
🌐 Exploring the Expansion of Inner Spheres in Higher Dimensions
This paragraph explores the surprising expansion of inner spheres as dimensions increase. It uses the analogy of a box and its corner spheres to illustrate how the radius of the inner sphere changes in higher dimensions. The paragraph describes how the inner sphere's radius increases from being smaller than the corner spheres in three dimensions to being the same size in four dimensions, and then exceeding the corner spheres' radii in five dimensions and beyond. The explanation includes the concept of 'real estate' shared among coordinates and how this affects the perceived size of the inner sphere. The paragraph concludes by emphasizing the importance of the slider method for teaching higher dimensions, as it provides a more concrete way of thinking about these abstract concepts.
Mindmap
Keywords
💡Geometry
💡Analytic Reasoning
💡Real Estate
💡Sliders
💡Higher Dimensions
💡Pythagorean Triples
💡Borsuk-Ulam Theorem
💡Intuition
💡Four-Dimensional Sphere
💡Counterintuitive
💡Visual Representation
Highlights
Mathematics uses the beauty of reasoning geometrically in two and three dimensions, creating a connection between numbers and spatial concepts.
The relationship between pairs or triplets of numbers and geometry helps clarify complex mathematical facts.
The usefulness of geometry in math is that it can make opaque facts clear through visual representation.
The connection between sums of squares and circles/spheres is central to many mathematical insights.
Mathematical intuition is limited when dealing with higher dimensions, as our physical space constrains our geometric understanding.
The challenge in higher dimensions is to conceptualize multiple numbers as points in a space, which is common in math, computer science, and physics.
A method for making analytic reasoning more visual is proposed, generalizing to high dimensions.
The focus is on higher dimensional spheres, such as a four-dimensional sphere with radius one centered at the origin.
The concept of 'real estate' in the context of geometry helps to understand the distribution of value between variables.
The analogy of 'real estate' allows for a useful visualization of the trade-off between variables in geometric terms.
The slider method provides a way to think about high-dimensional spaces by representing each coordinate with a slider.
The slider method helps to understand movements on a sphere by visualizing the exchange of 'real estate' between coordinates.
The example of a 2x2 box and tangent circles in two dimensions demonstrates the method for finding the radius of an inner circle.
In higher dimensions, the radius of the inner sphere increases unexpectedly, leading to counterintuitive results.
The slider method and real estate analogy provide a more intuitive understanding of high-dimensional mathematical phenomena.
In ten dimensions, the inner sphere's radius is more than twice as large as the corner spheres, a result of the distribution of real estate across ten coordinates.
The slider method and real estate analogy help demystify high-dimensional spaces and make them more accessible for mathematical exploration.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: