2021 AP Physics 1 Free Response #3 (First Administration)
TLDRThis video script discusses a physics problem from the 2021 AP Physics 1 exam involving a student pushing a disk on a frictionless surface with a constant force. The problem explores impulse, momentum, and the final velocities of both the disk and the student. It also includes scenarios where the disk collides with a block of different masses, estimating the velocity of the center of mass after the collision. The explanation uses conservation of momentum and impulse-momentum principles to derive equations and ratios, providing insights into the physics of the situation.
Takeaways
- 📚 The script is a walkthrough of a physics problem from the 2021 AP Physics 1 exam.
- 🧑🎓 A student with mass \( m_s \) uses a stick to apply a constant horizontal force \( F_h \) on a disk with mass \( m_d \) over time interval \( t_f \).
- 🏋️♂️ The student and the disk are on a smooth surface with negligible friction.
- 🔄 The impulse, which is the product of force and time, causes a change in momentum of the disk.
- 📉 The final velocity of the disk \( v_d \) is found by dividing the impulse by the mass of the disk \( m_d \).
- ⚖️ The ratio of the final velocities of the disk to the student \( v_d / v_s \) is derived to be \( m_s / m_d \).
- 📈 The script discusses how to sketch graphs of the speeds of both the student and the disk as functions of time.
- 💥 In a collision, if the disk's mass is much greater than the block's mass, the center of mass velocity \( v_{cm} \) is approximately equal to \( v_1 \), the initial velocity of the disk.
- 🚫 Conversely, if the disk's mass is much less than the block's mass, the center of mass velocity \( v_{cm} \) is approximately zero.
- 🔄 For a collision where neither object's mass is significantly greater, conservation of momentum is used to derive an equation for \( v_{cm} \).
- 🔄 The script also checks if the equations derived for \( v_{cm} \) in different scenarios are consistent with the physical reasoning provided.
Q & A
What is the scenario described in the third question of the 2021 AP Physics 1 exam?
-A student of mass ms is standing on a smooth surface and uses a stick to push a disk of mass md with a constant horizontal force fh over time from 0 to tf. The friction between the student's shoes and the surface is negligible.
What is the expression for the final speed of the disk relative to the surface?
-The final speed of the disk (vd) can be determined using the impulse-momentum theorem, which states that the impulse (force times time, fh*t) is equal to the change in momentum (md*vd). Therefore, vd = (fh*t) / md.
How is the impulse defined in the context of this problem?
-In this context, the impulse is defined as the product of the constant force (fh) and the time interval (t) over which the force is applied.
What is the relationship between the student's mass (ms) and the disk's mass (md) in determining the final speed of the student (vs)?
-The student's final speed (vs) is determined by the impulse experienced by the student, which is the same as the impulse on the disk but in the opposite direction. Thus, vs = (fh*t) / ms. The ratio of the disk's final speed to the student's final speed is given by vs/vd = ms/md.
What is the significance of the assumption of negligible friction in this problem?
-The assumption of negligible friction allows for the simplification of the problem by eliminating external forces that could affect the motion of the student and the disk, focusing solely on the force exerted by the student on the disk.
How can the ratio of the disk's final speed to the student's final speed be derived using conservation of momentum?
-By considering the system consisting of the student and the disk, the total momentum before and after the interaction must be conserved. Since the disk exerts an equal and opposite impulse on the student, the ratio of their final speeds can be found by setting the initial momentum of the disk equal to the final momentum of the combined system.
What does the script suggest about the physical interpretation of the ratio of the student's mass to the disk's mass?
-The script suggests that a larger student mass (ms) compared to the disk mass (md) would result in a smaller final speed for the student (vs), indicating that the student's motion would be less affected by the interaction.
How should the graph of the speeds of both the student and the disk as functions of time be sketched according to the script?
-The graph should show the speeds (not velocities, hence ignoring direction) of both the student and the disk as linear functions of time, with the disk's speed increasing until tf and then remaining constant, while the student's speed would be proportional to the disk's but scaled by the ratio of their masses.
What happens when the disk, moving at a constant speed, collides head-on with a block of mass mb that is at rest on the surface?
-The disk and the block collide and stick together, moving with a new velocity equal to the velocity of the center of mass of the combined system (vcm).
How is the velocity of the center of mass of the disk-block system estimated if the mass of the disk is much greater than that of the block?
-If the disk's mass is much greater than the block's mass, the velocity of the center of mass (vcm) is approximately equal to the initial velocity of the disk (v1), as the impulse from the block on the disk is negligible.
What is the equation for the velocity of the center of mass of the disk-block system if neither object's mass is much greater than the other?
-Using conservation of momentum, the equation for the velocity of the center of mass (vcm) after the collision is vcm = (md*v1 + mb*0) / (md + mb), where md is the mass of the disk, mb is the mass of the block, and v1 is the initial velocity of the disk.
Outlines
🚀 Impulse and Momentum in Physics Problem
The first paragraph discusses a physics problem from the 2021 AP Physics 1 exam involving a student exerting a constant horizontal force on a disk with negligible friction. The student's force over time is described as an impulse, which results in a change in the disk's momentum. The final speed of the disk is calculated using the impulse-momentum theorem, and the equation is derived as the impulse divided by the disk's mass. Additionally, the student's speed is derived using the same principles, and the ratio of the disk's speed to the student's speed is found to be the inverse of their masses. The paragraph concludes with a task to sketch graphs of the speeds of both the student and the disk as functions of time.
🎯 Collision Dynamics and Center of Mass Estimations
The second paragraph extends the physics scenario to include a collision between a moving disk and a stationary block. The discussion begins with the case where the disk's mass is significantly larger than the block's mass, leading to an estimate that the center of mass of the combined system will move at a speed close to the disk's initial speed due to the negligible impulse from the block. Conversely, if the disk's mass is much less than the block's, the center of mass velocity is estimated to be nearly zero. The paragraph then considers the case where neither mass is significantly larger, and a conservation of momentum equation is derived to calculate the velocity of the center of mass after the collision. The summary includes a comparison of the derived equation with the reasoning from the first scenario, confirming that the equations align with the physical expectations.
Mindmap
Keywords
💡Impulse
💡Momentum
💡Constant Force
💡Friction
💡Velocity
💡Ratio
💡Conservation of Momentum
💡Center of Mass
💡Collision
💡Estimate
Highlights
The problem involves a student using a stick to push a disk on a smooth surface with negligible friction.
A constant horizontal force is exerted on the disk over a time interval from 0 to tf.
The impulse exerted on the disk is equal to the force times the time (fh * t).
The final speed of the disk (vd) is determined by the impulse divided by the disk's mass (md).
The student's speed (vs) after applying the force is derived from the impulse divided by the student's mass (ms).
The ratio of the disk's final speed to the student's speed (vd/vs) is expressed in terms of their masses (ms/md).
Conservation of momentum or the concept of equal and opposite impulses can be used to derive the equation for vs.
The ratio vd/vs is inversely proportional to the ratio of the student's mass to the disk's mass.
The physical interpretation of the ratio is that a larger student mass results in a smaller speed change for the student.
The student and disk's speeds are to be sketched as functions of time, considering only positive values up to 2tf.
The disk's speed graph is expected to show a linear increase followed by a constant speed after tf.
The student's speed graph is influenced by the ratio of their masses and will be lower than the disk's.
The disk collides head-on with a block of mass mb, and the center of mass of the disk-block system moves with speed vcm.
If the disk's mass is much greater than the block's, the center of mass velocity is approximately equal to the disk's initial speed (v1).
If the disk's mass is much less than the block's, the center of mass velocity is approximately zero.
For a case where neither mass is significantly greater, conservation of momentum is used to derive the equation for vcm.
The derived equation for vcm in part c3 should agree with the reasoning for part c1 when md is much greater than mb.
Transcripts
Browse More Related Video
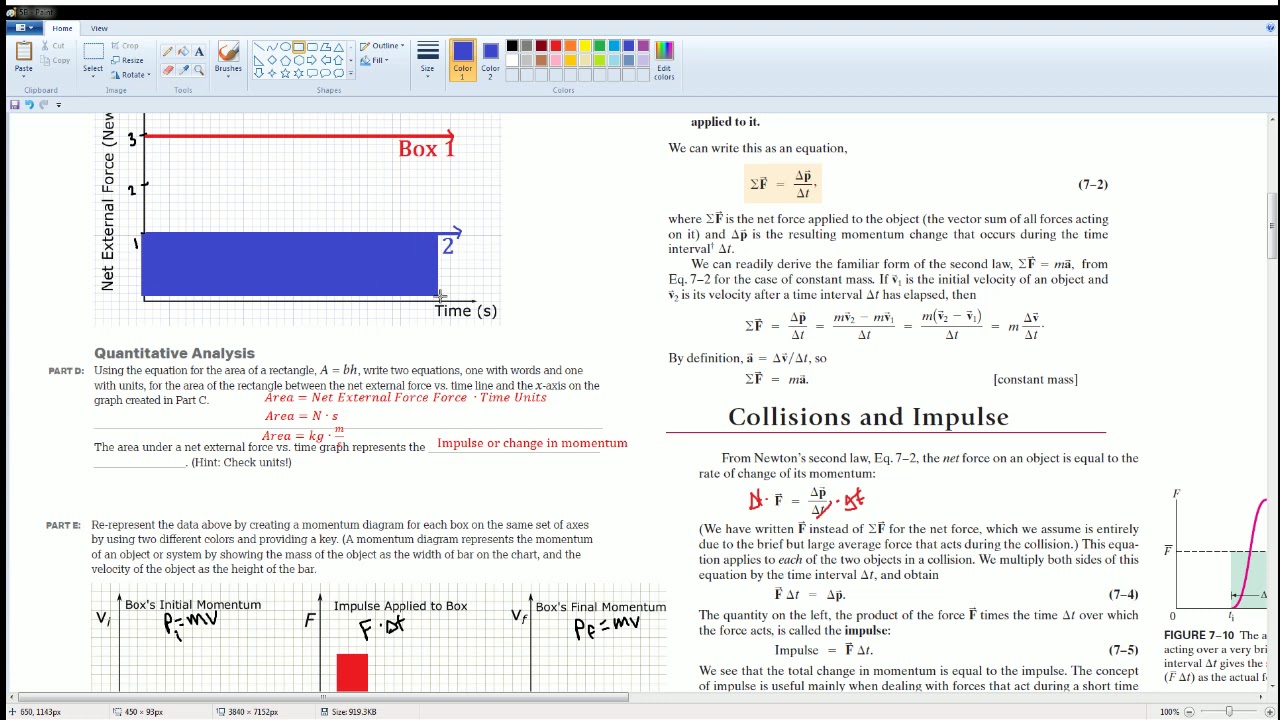
AP Physics Workbook 5.B Impulse
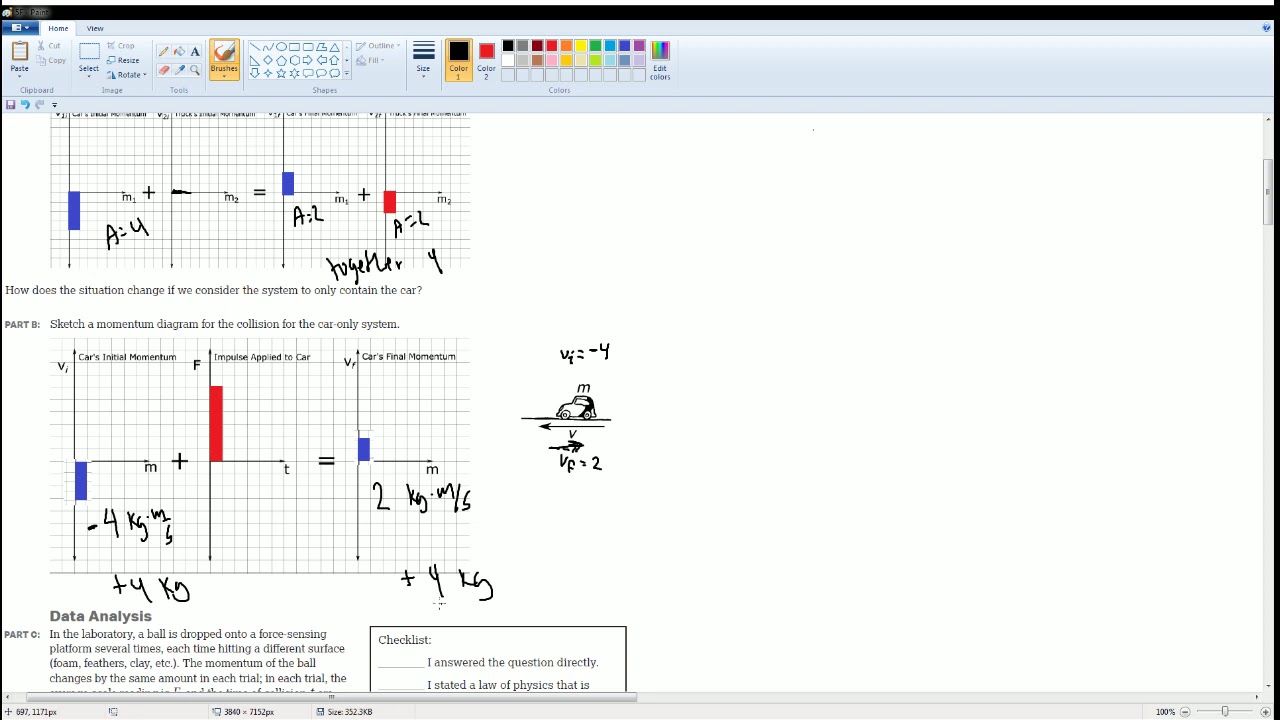
AP Physics Workbook 5.F Conservation of momentum in Elastic Collisions
2019 #1 Free Response Question - AP Physics 1 - Exam Solution

2022 Live Review 5 | AP Physics 1 | Understanding Impulse and Momentum

2023 AP Physics 1 Free Response #4

2023 AP Physics C Mechanics (Set 1)
5.0 / 5 (0 votes)
Thanks for rating: