Unit I: Lec 4 | MIT Calculus Revisited: Single Variable Calculus
TLDRThis MIT OpenCourseWare lecture delves into the foundational concept of limits in calculus, essential for understanding derivatives. The professor uses the analogy of a freely falling object to illustrate limits and their subtleties, highlighting the difference between average and instantaneous rates of change. He emphasizes the importance of rigorous definitions in calculus to avoid common pitfalls, such as division by zero, and provides a detailed explanation of the epsilon-delta definition of a limit, relating it back to the intuitive understanding of limits through graphical representation.
Takeaways
- 📚 The lecture focuses on the concept of limits, a fundamental aspect of calculus, and its subtlety in mathematical history.
- 💡 The professor uses the analogy of electricity and a light switch to illustrate the seemingly simple yet complex nature of limits.
- 🚀 The lecture introduces limits through the context of differential calculus, specifically with the example of a freely falling object.
- 📉 The distinction between average speed and instantaneous speed is highlighted, with the latter being the focus of the lecture.
- 🔍 The process of finding the instantaneous speed involves taking a limit as the time interval approaches zero, which is not as straightforward as it seems.
- 📈 The concept of average rate of change leads to the definition of the derivative, which is the instantaneous rate of change.
- 📚 The lecture emphasizes the importance of not allowing the variable 'h' to equal zero when finding limits, to avoid undefined expressions.
- 🤔 The intuitive approach to limits is contrasted with the rigorous epsilon-delta definition, which provides a precise mathematical framework.
- 📉 The graphical representation of limits is discussed, showing how the slope of the tangent line to a curve at a point represents the instantaneous rate of change.
- 🔢 The lecture provides a detailed example of finding a limit using the epsilon-delta definition, illustrating the process algebraically and geometrically.
- 🛠️ The importance of the function being one-to-one when dealing with limits is mentioned, as non-single valued functions can lead to ambiguity in the limit.
Q & A
What is the main topic of the lecture?
-The main topic of the lecture is the concept of limits in calculus, with a focus on derivatives and how they relate to instantaneous rates of change, such as the speed of a freely falling object.
Why is the concept of limits considered subtle in the history of mathematics?
-The concept of limits is considered subtle because, while it seems to give the right answer most of the time from a computational standpoint, there are instances, particularly in differential calculus, where the 1% of cases not covered by simple computation can lead to significant misunderstandings.
What is the anecdote about the professor and his wife related to?
-The anecdote is related to the simplicity and complexity of understanding electricity versus the subtlety of understanding the concept of limits in calculus.
What is the formula given for the distance a freely falling object falls in a vacuum?
-The formula given for the distance 's' a freely falling object falls in a vacuum is s = 16t^2, where 's' is the distance in feet and 't' is the time in seconds.
What is the difference between average speed and instantaneous speed?
-Average speed is calculated over a time interval and represents the total distance traveled divided by the time taken. Instantaneous speed, on the other hand, is the speed of an object at a specific moment in time, which can be found by taking the limit of the average speed as the time interval approaches zero.
How does the lecture illustrate the transition from average speed to instantaneous speed?
-The lecture illustrates this transition by progressively reducing the time interval over which the average speed is calculated, starting from an interval between t=1 and t=2, then to t=1 and t=1.1, and finally considering an infinitesimally small interval around t=1, represented as t=1+h.
What is the significance of the expression '32h + 16h^2' in the context of the lecture?
-The expression '32h + 16h^2' represents the average speed of the falling object as time 't' transitions from 1 to '1+h'. As 'h' approaches zero, this expression is used to approximate the instantaneous speed at t=1, which is suspected to be 32 feet per second.
Why is it incorrect to simply replace 'x' with 'a' when calculating the limit of a function as 'x' approaches 'a'?
-It is incorrect because the limit definition requires that 'x' never actually equals 'a'. Simply replacing 'x' with 'a' can lead to indeterminate forms, such as 0/0, which do not accurately represent the limit's value.
What is the epsilon-delta definition of a limit?
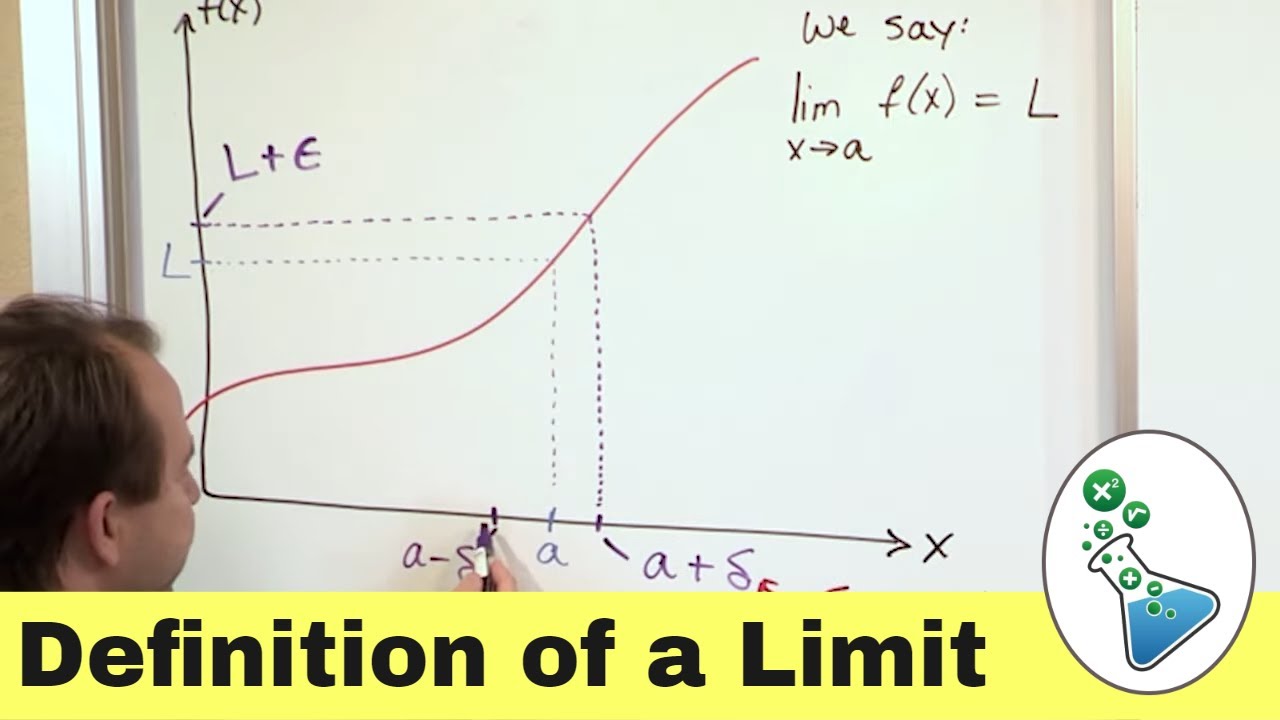
-The epsilon-delta definition states that the limit of 'f(x)' as 'x' approaches 'a' equals 'l' if, for any given epsilon > 0, there exists a delta > 0 such that if 0 < |x - a| < delta, then |f(x) - l| < epsilon.
How does the lecture use the concept of a tangent line to explain derivatives?
-The lecture explains that the derivative, or the instantaneous rate of change at a point, can be visualized as the slope of the tangent line to the curve of the function at that point. As 'delta x' approaches zero, the average rate of change becomes the slope of the tangent line, which is the derivative.
What is the purpose of the rigorous epsilon-delta definition of a limit?
-The purpose of the rigorous epsilon-delta definition is to provide an unambiguous and precise mathematical description of what a limit means, avoiding the pitfalls and misinterpretations that can arise from intuitive or informal definitions.
Can you provide an example from the script where the epsilon-delta definition is applied?
-Yes, the script applies the epsilon-delta definition to the limit of 'x^2 - 2x' as 'x' approaches three. It shows how to algebraically find a delta that ensures 'x^2 - 2x - 3' is within epsilon of zero when 'x' is within delta of three, without 'x' actually being equal to three.
What is the importance of the function being one-to-one in the context of limits?
-The importance of the function being one-to-one is that it ensures a unique output for each input, which is crucial for the limit definition to work properly. If the function is not one-to-one, there could be multiple points where the function has the same output, leading to ambiguity in determining the limit.
How does the script illustrate the geometric interpretation of the epsilon-delta definition?
-The script uses a graph of 'y = f(x)' to illustrate the geometric interpretation. It shows how for a given epsilon, horizontal lines at 'l + epsilon' and 'l - epsilon' can be projected onto the curve to find the corresponding range of 'x' values (the delta) that ensure 'f(x)' is within epsilon of 'l' when 'x' is close to 'a'.
Outlines
📚 Introduction to Calculus and Limits
The speaker introduces the concept of limits, a fundamental topic in calculus, and its subtlety in mathematical history. Using an anecdote about electricity, the professor illustrates the intuitive yet complex nature of limits. The lecture aims to explore derivatives and limits, starting with the example of a freely falling object and the formula for the distance it falls, 's = 16t^2'. The focus is on determining the instantaneous speed at a specific time, differentiating it from the average speed, which is a more familiar concept.
🔍 Exploring Instantaneous Speed and Average Speed
The paragraph delves into the difference between average and instantaneous speed, using the example of a ball falling in a vacuum. It explains how to calculate the average speed over different time intervals and suggests that a smaller interval might provide a better approximation of the instantaneous speed at a particular moment. The mathematical process involves computing the distance 's' at time 't' and time 't+h', and then finding the average speed over the interval 'h'. The concept is that as 'h' approaches zero, the average speed over the interval '1 to 1+h' should approximate the instantaneous speed at 't=1'.
📉 The Concept of Limits in Calculus
This section discusses the formal definition of limits in calculus. It explains the process of finding the average speed between 't=1' and 't=1+h' and then investigates what happens as 'h' approaches zero. The paragraph emphasizes the importance of not allowing 'h' to equal zero, as this would lead to division by zero, which is undefined. The goal is to understand the behavior of the function as 'h' gets arbitrarily small without actually reaching zero, which is central to the concept of a limit.
🔑 Generalizing the Concept of Instantaneous Speed
The speaker generalizes the concept of instantaneous speed by considering it not just at 't=1' but at any time 't'. The paragraph explains how the instantaneous speed can be found for any given time 't' by using the same method of taking limits as 'h' approaches zero. The result is that the instantaneous speed of a freely falling object is '32t', which is derived from the equation of motion 's=16t^2' by applying the limit process.
📈 The Role of Analytic Geometry in Calculus
The importance of analytic geometry in the study of calculus is highlighted, emphasizing how a graphical representation can clarify complex concepts. The paragraph discusses how the average rate of change of a function can be visualized as the slope of a line segment joining two points on a graph. It also points out the limitations of average values and the importance of considering the behavior of a function over smaller intervals to better approximate the instantaneous rate of change.
📘 Defining the Derivative and the Limit Process
This section focuses on defining the derivative as the instantaneous rate of change at a point and relating it to the limit of the average rate of change as the interval approaches zero. The paragraph explains the geometric interpretation of the derivative as the slope of the tangent line to a curve at a given point. It also discusses the potential pitfalls of using the intuitive approach to limits and the need for a more rigorous definition.
🤔 The Challenge of Understanding Limits
The paragraph addresses the challenges in understanding the concept of limits, particularly the indeterminate forms like 0/0 that often arise in calculus. It uses the example of the function 'f(x) = (x^2 - 9)/(x - 3)' to illustrate how the limit process can lead to such forms and the importance of not simply substituting the limit value into the function. The speaker emphasizes the need for a more objective criterion to define limits.
📚 The Mathematical Definition of Limit
The speaker provides the formal mathematical definition of a limit, explaining the epsilon-delta concept. It describes how for any given positive tolerance level (epsilon), a corresponding delta can be found such that if the distance between 'x' and 'a' is less than delta, then the difference between 'f(x)' and 'l' is less than epsilon. The paragraph aims to make this rigorous definition more understandable by relating it to the intuitive idea of getting arbitrarily close to a value.
📉 Graphical Interpretation of Limits
This section uses a graphical approach to illustrate the epsilon-delta definition of a limit. It explains how the limit of a function as 'x' approaches 'a' can be visualized on a graph, with horizontal lines representing the tolerance limits around the limit value 'l'. The paragraph discusses how the distance between 'x' and 'a' corresponds to the vertical distance between the function value and the limit value within the specified tolerance.
🔍 Analyzing the Limit of a Quadratic Function
The paragraph applies the concept of limits to the quadratic function 'f(x) = x^2 - 2x' as 'x' approaches three. It uses both graphical and algebraic methods to demonstrate that the limit is three. The speaker shows how to use the epsilon-delta definition to find the appropriate delta that ensures 'f(x)' is within epsilon of three when 'x' is close to but not equal to three.
📝 Conclusion and Future Topics
In conclusion, the speaker summarizes the importance of understanding the epsilon-delta definition of limits and its application in calculus. They acknowledge the difficulty of the topic and promise to develop more straightforward methods for solving limit problems in the next lecture. The speaker also emphasizes the practical applications of the epsilon-delta approach in making approximations and understanding tolerance limits.
Mindmap
Keywords
💡Limit
💡Creative Commons license
💡MIT OpenCourseWare
💡Derivatives
💡Average speed
💡Instantaneous speed
💡Freely falling object
💡Analytic Geometry
💡Tangent line
💡Epsilon-delta definition
💡Indeterminate form
Highlights
The lecture introduces the concept of limit, a fundamental aspect of Calculus, through the context of differential Calculus.
The professor uses the anecdote of a professor contemplating electricity to illustrate the subtlety of the limit concept.
The importance of distinguishing between average speed and instantaneous speed is discussed, with the latter being the focus of the lecture.
The freely falling object example is used to demonstrate the calculation of instantaneous speed at a specific time.
The concept of average rate of speed is used as a stepping stone to understand the more complex idea of instantaneous speed.
The lecture explains how to calculate the average speed of a ball during a time interval and how it relates to the concept of limits.
The process of narrowing the time interval to better approximate the instantaneous speed is discussed.
The transition from average speed to instantaneous speed is shown through the use of the variable 'h' representing an infinitesimally small change in time.
The mathematical expression for the average speed as h approaches zero is derived, leading to the concept of the derivative.
The significance of not dividing by zero when taking the limit is emphasized, highlighting the mathematical rigor required.
The lecture generalizes the concept of instantaneous speed to any time 't', not just at 't' equals one.
The graphical representation of the concept of limit and derivative is introduced, relating to the slope of the tangent line to a curve at a point.
The difference between the average rate of change and the instantaneous rate of change is clarified using geometric interpretations.
The lecture addresses the common mistake of assuming the limit can be found by simply substituting the limit value into the function.
The rigorous definition of a limit using epsilon and delta is introduced to provide a precise mathematical framework.
The importance of the function being one-to-one when working with limits is discussed to avoid ambiguity in the graphical interpretation.
The lecture concludes with an exercise to find the limit of a quadratic function as x approaches three, demonstrating the epsilon-delta definition in practice.
The graphical and algebraic methods are correlated to provide a comprehensive understanding of the limit concept.
The potential difficulties and the need for rigorous criteria in determining limits are highlighted through examples and exercises.
Transcripts
Browse More Related Video
Limits in Calculus: Definition & Meaning. What is a Limit?

Unit I: Lec 5 | MIT Calculus Revisited: Single Variable Calculus

Precise Definition of a Limit - Example 1 Linear Function

Epsilon-delta limit definition 2 | Limits | Differential Calculus | Khan Academy
Calculus Chapter 1 Lecture 7 Limits

Epsilon-delta definition of limits
5.0 / 5 (0 votes)
Thanks for rating: