Vertical distance of bouncing ball | Sequences, series and induction | Precalculus | Khan Academy

Khan Academy
8 Nov 201305:10
EducationalLearning
32 Likes 10 Comments

TLDRThis video explores the total vertical distance a ball travels when dropped from 10 meters and bounces with each peak height halving. It demonstrates how the ball's journey forms an infinite geometric series, calculating the sum to be 30 meters, highlighting the intriguing mathematical pattern in everyday phenomena.

Takeaways
  • 🏀 The scenario involves a ball dropped from a height of 10 meters that bounces back to half its previous height with each bounce.
  • 📉 The ball's height after each bounce follows a geometric progression, halving each time.
  • 🔽 The initial drop is 10 meters straight down, contributing to the total vertical distance traveled.
  • 🔼 The first bounce includes a 5-meter ascent and descent, totaling 10 meters of vertical travel.
  • 🔄 Each subsequent bounce involves both an ascent and descent, with the total vertical distance being twice the height reached by the bounce.
  • 📈 The total vertical distance traveled by the ball can be represented as an infinite geometric series.
  • 🔢 The formula for the sum of an infinite geometric series is a / (1 - r), where 'a' is the first term and 'r' is the common ratio.
  • 🌐 The series in the script is simplified to -10 + 20 * (1/2)^k, where k starts from 0 and goes to infinity.
  • 🧩 The negative sign in the series represents the initial drop, and the 20 represents the total vertical distance of each bounce (up and down).
  • 📊 Applying the geometric series formula, the sum is calculated as 20 / (1 - 1/2), which simplifies to 40.
  • 🎯 The total vertical distance the ball travels, accounting for all the bounces, is 30 meters, combining the initial drop and the series sum.
Q & A

  • What is the initial height from which the ball is dropped?

    -The ball is initially dropped from a height of 10 meters.

  • How does the ball's bounce height change with each bounce?

    -With each bounce, the ball reaches half the height of the previous bounce.

  • What is the peak height of the ball's second bounce?

    -The peak height of the ball's second bounce is 5 meters.

  • How high does the ball go on its third bounce?

    -On its third bounce, the ball goes 2.5 meters high.

  • What is the total vertical distance the ball travels in its first two bounces?

    -In its first two bounces, the ball travels a total vertical distance of 20 meters (10 meters down and 10 meters up).

  • How is the vertical distance the ball travels related to a geometric series?

    -The vertical distance the ball travels forms an infinite geometric series, where each term represents the distance traveled up and down during each bounce.

  • What is the formula for the sum of an infinite geometric series?

    -The sum of an infinite geometric series is given by \( a \over 1 - r \), where \( a \) is the first term and \( r \) is the common ratio.

  • What is the common ratio in the series representing the ball's vertical distance?

    -The common ratio in the series is 1/2, as the ball's bounce height decreases by half each time.

  • How is the initial drop of 10 meters represented in the geometric series?

    -The initial drop of 10 meters is represented as -10 in the geometric series, accounting for the downward motion.

  • What is the total vertical distance the ball travels according to the geometric series calculation?

    -The total vertical distance the ball travels is 30 meters, calculated as -10 + 40 (from the sum of the infinite geometric series).

  • Why is the initial drop considered as -10 in the geometric series calculation?

    -The initial drop is considered as -10 to account for the downward direction, making the series start from zero and simplify the calculation.

Outlines
00:00
🏀 Bouncing Ball's Infinite Geometric Series

This paragraph describes a scenario where a ball is dropped from a height of 10 meters and each subsequent bounce reaches half the height of the previous one. The script explains the concept of an infinite geometric series by calculating the total vertical distance the ball travels, including both ascents and descents. The process involves summing up the series of distances the ball travels upwards and downwards after each bounce, which is simplified into a geometric series formula. The common ratio is identified as 1/2, and the first term of the series is 10 meters. The sum of the series is then calculated using the formula for an infinite geometric series, resulting in a total vertical distance of 30 meters for the ball.

05:02
📏 Total Vertical Distance Calculation

The second paragraph reiterates the conclusion from the previous paragraph, confirming that the total vertical distance the ball travels is 30 meters. This serves as a brief recap and affirmation of the mathematical result obtained from the analysis of the bouncing ball scenario, emphasizing the final answer to the question posed in the video script.

Mindmap
Keywords
💡Ball
In the context of the video, 'ball' refers to the object that is dropped from a height and bounces multiple times. It is the central subject of the experiment being discussed, illustrating the concept of vertical distance traveled during its descent and ascent.
💡Height
The term 'height' is used to describe the initial distance from which the ball is dropped, which is 10 meters. It is a critical parameter in calculating the total vertical distance the ball travels, as each subsequent bounce reaches half the height of the previous one.
💡Bounce
A 'bounce' in the video refers to each time the ball reaches its peak height after being dropped and then falling back down. The concept of bounce is essential in understanding the pattern of the ball's movement and how it affects the total vertical distance traveled.
💡Peak Height
'Peak height' is the maximum vertical distance the ball reaches during each bounce. The script explains that each bounce reaches half the peak height of the previous one, starting from 10 meters and decreasing exponentially.
💡Vertical Distance
This term is central to the video's theme, as it describes the total distance the ball travels in a vertical direction. The script calculates this by adding the distances traveled during each descent and ascent, emphasizing the importance of considering both upward and downward movements.
💡Geometric Series
A 'geometric series' is a mathematical concept introduced in the video to describe the pattern of the ball's bounces. It is an infinite series where each term is a constant multiple of the previous term, which in this case is 1/2. The video uses this series to calculate the total vertical distance traveled by the ball.
💡Common Ratio
The 'common ratio' in the context of the video is the factor by which each term in the geometric series is multiplied to get the next term. Here, it is 1/2, indicating that each bounce reaches half the height of the previous one.
💡Infinite Series
An 'infinite series' is a series that continues indefinitely. The video uses the concept of an infinite geometric series to model the ball's continuous bouncing, where each bounce is half the height of the previous one, theoretically extending forever.
💡Sum
The 'sum' in the video refers to the total of all terms in the geometric series, which in this case represents the total vertical distance the ball travels. The script uses the formula for the sum of an infinite geometric series to find this total.
💡Formula
The 'formula' mentioned in the video is used to calculate the sum of an infinite geometric series, which is \( a \over 1 - r \). Here, \( a \) is the first term and \( r \) is the common ratio. The video applies this formula to find the total vertical distance the ball travels, which turns out to be 30 meters.
Highlights

The ball is dropped from a height of 10 meters and bounces to half the height of the previous bounce.

The ball's first peak height after bouncing is 5 meters.

The ball's subsequent bounces follow a pattern of decreasing height by half each time.

The total vertical distance the ball travels is the focus of the problem.

The ball first travels 10 meters straight down.

Each bounce involves the ball traveling up and down the same distance.

The total vertical distance for each bounce is calculated by considering both up and down movements.

The problem is approached by considering the ball's movement as an infinite geometric series.

The sum of an infinite geometric series is derived as a/(1-r), where a is the first term and r is the common ratio.

The series representing the ball's total vertical distance is simplified and rewritten for clarity.

The first term of the series is -10, representing the initial 10 meter drop.

The common ratio for the series is 1/2, as each bounce height is half of the previous one.

The sum of the series is calculated to find the total vertical distance traveled by the ball.

The sum is found to be 20 over (1 - 1/2), which simplifies to 40 meters.

The total vertical distance the ball travels, including the initial drop, is 30 meters.

The problem demonstrates the application of geometric series in real-world scenarios involving repeated bouncing.

The solution process involves breaking down the problem into a series of up and down movements, each represented as a term in the geometric series.

The final answer is obtained by summing the series and adding the initial drop distance.

Transcripts

Browse More Related Video

Repeating decimal as infinite geometric series | Precalculus | Khan Academy

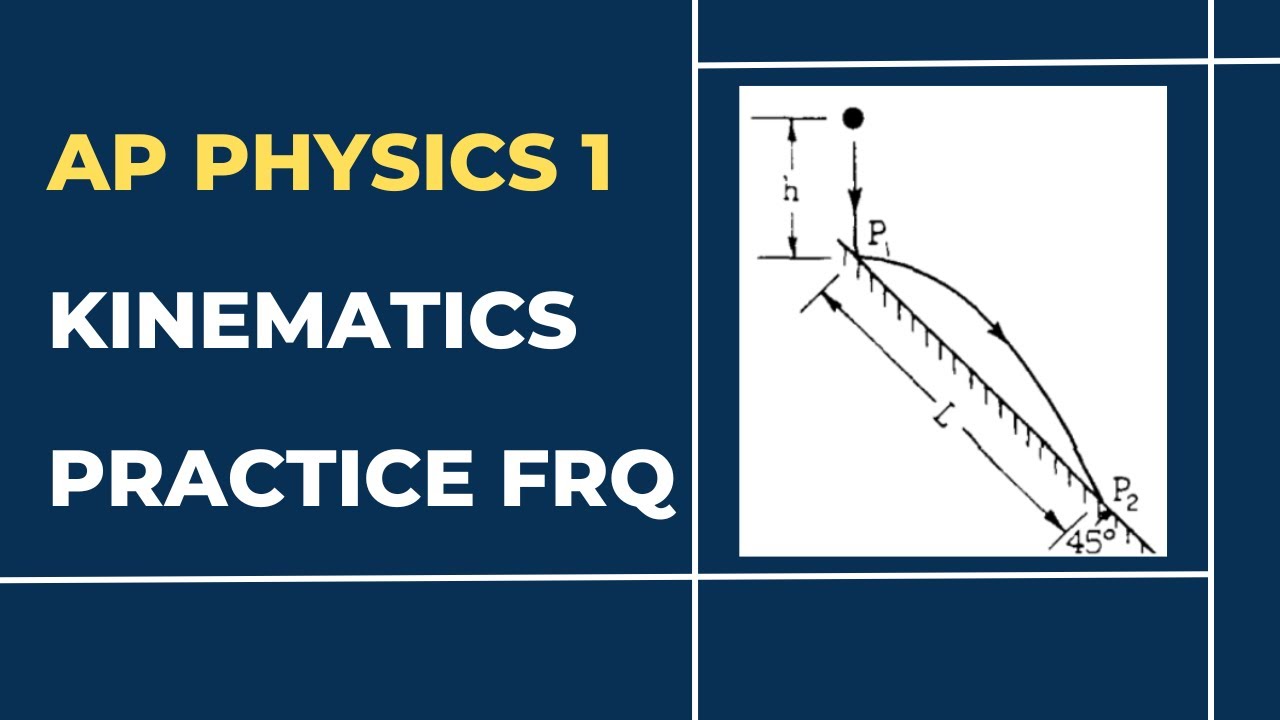
AP Physics 1 Kinematics Problem #5

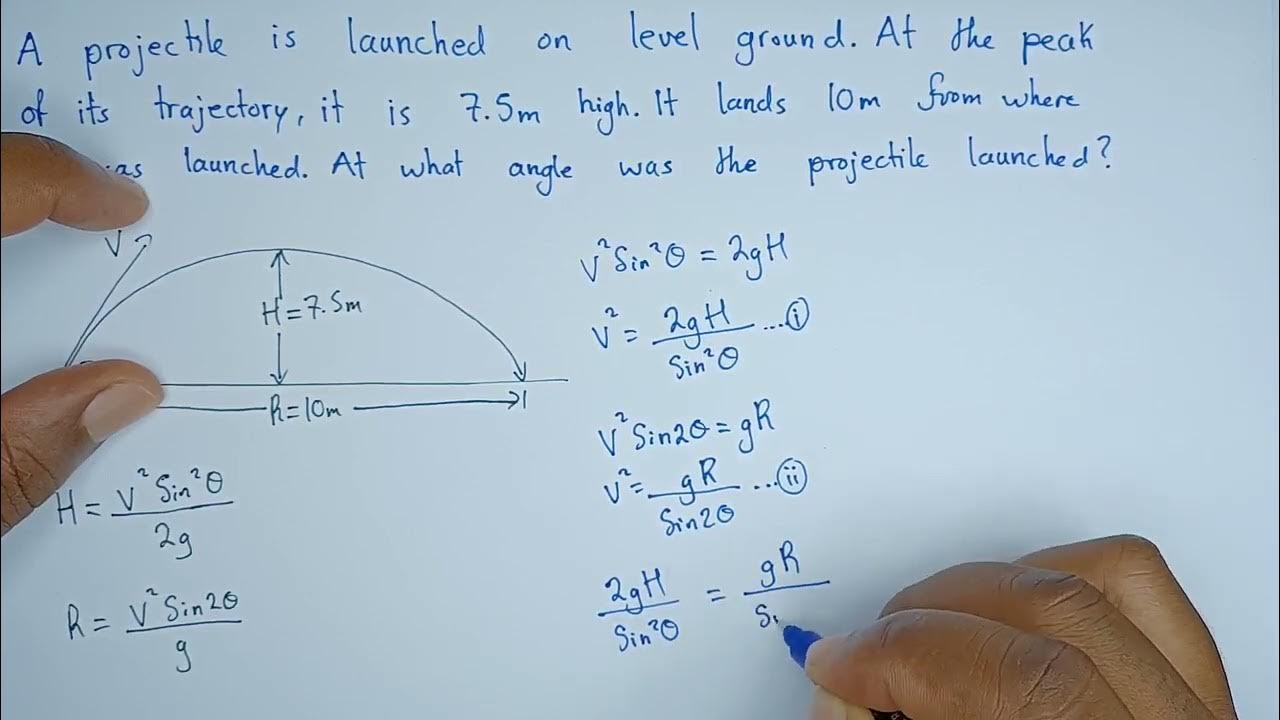
How to calculate the angle of projection - Projectile Motion

Series sum example | Sequences, series and induction | Precalculus | Khan Academy

Worked examples: finite geometric series | High School Math | Khan Academy

AP Physics B Kinematics Presentation General Problems #11

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Bouncing BallGeometric SeriesVertical DistancePhysics ProblemMathematicsEducational VideoInfinite SeriesBounce HeightMotion AnalysisLearning Material