Lec 21: Gradient fields and potential functions | MIT 18.02 Multivariable Calculus, Fall 2007

MIT OpenCourseWare

27 Jan 200950:11

EducationalLearning

32 Likes 10 Comments

TLDRThis lecture explores the concept of vector fields, particularly gradient fields, and their properties. It delves into the criteria for determining if a vector field is a gradient field by examining the equality of mixed partial derivatives. The lecture introduces the notion of path independence and conservation of energy, highlighting their equivalence to being a gradient field. It also covers methods for finding potential functions and discusses the curl, a measure of rotation in a field, with applications in physics such as fluid dynamics and torque in force fields.

Takeaways

📚 The script discusses the concept of vector fields, particularly focusing on gradient fields and their properties.
🔍 A gradient field is identified by the property that the line integral of the field is path-independent, meaning the work done depends only on the endpoints of the path.
🔄 The script explains that a vector field is conservative if it satisfies the condition that the line integral over a closed curve is zero, indicating no net work is done.
📉 The criterion for determining if a vector field is a gradient field is presented, which involves checking if the mixed partial derivatives are equal (i.e., M_y = N_x).
📈 The importance of the vector field being defined and differentiable everywhere in the plane is highlighted, as this is a prerequisite for the field to be a gradient field.
🔑 The script introduces the concept of 'curl' as a measure of the rotation or twisting in a vector field, which is crucial for understanding conservative fields.
🌀 The curl is defined as the difference between the partial derivatives of the vector field components (N_x - M_y), and a zero curl indicates a conservative field.
🛠 Two methods for finding the potential function of a gradient field are presented: computing line integrals and using anti-derivatives.
🔍 The method of computing line integrals involves integrating along a path from a starting point to an endpoint and is related to the change in potential.
📝 The anti-derivatives method involves solving for the potential function by integrating one component of the field with respect to one variable and then differentiating with respect to the other.
🌐 The script also touches on the implications of the vector field being defined in simply connected regions and the challenges it poses for the potential function being well-defined.

Q & A

What is a gradient field in the context of vector calculus?
-A gradient field is a vector field that is the gradient of a scalar potential function. It has the property that the line integral of the field over a path depends only on the endpoints of the path, not the path itself, making it path-independent.
What does it mean for a line integral to be path independent?
-A line integral is path independent if the work done by the vector field along a curve from one point to another is the same regardless of the actual path taken between those points.
Why is a conservative vector field important in physics?
-A conservative vector field is important in physics because it implies the conservation of energy. If you move an object in a closed loop within such a field, the net work done is zero, indicating no net energy is gained or lost.
What is the criterion for determining if a vector field is a gradient field?
-The criterion for a vector field to be a gradient field is that the mixed partial derivatives of the potential function are equal, i.e., ∂M/∂y = ∂N/∂x, where M and N are the components of the vector field.
What is the physical interpretation of the curl of a vector field?
-The curl of a vector field measures the rotation or vorticity of the field at a given point. It indicates the tendency of the field to induce circular motion or rotation.
How does the curl relate to the conservative property of a vector field?
-The curl of a vector field being zero is a necessary and sufficient condition for the field to be conservative. If the curl is non-zero, the field cannot be conservative.
What is the significance of the term 'simply connected region' in the context of vector fields?
-A simply connected region is a region in the plane that does not have any 'holes'. If a vector field is defined in a simply connected region and satisfies the condition ∂M/∂y = ∂N/∂x, then the field is guaranteed to be a gradient field.
Can a vector field be a gradient field if it is only defined in part of the plane?
-No, a vector field can only be a gradient field if it is defined and differentiable everywhere in the plane or in a simply connected region. If it is undefined at some points, it may not be a gradient field even if it satisfies the condition ∂M/∂y = ∂N/∂x in the defined region.
What is the method for finding the potential function of a given gradient field?
-One method involves computing line integrals along specific paths and setting the potential at the end point equal to the line integral plus a constant. Another method involves solving the system of partial differential equations given by the components of the vector field, integrating with respect to one variable and then differentiating with respect to the other to find the potential function.
How does the concept of curl relate to torque in the context of force fields?
-The curl of a force field measures the torque exerted on a test object placed at a point in the field. It is a measure of how the force field tends to cause rotation or angular acceleration of the object.
What is the fundamental theorem of line integrals mentioned in the script?
-The fundamental theorem of line integrals states that the line integral of a gradient field from one point to another is equal to the change in the potential function between those two points, which is a direct consequence of the path independence of gradient fields.

Outlines

00:00

📚 Introduction to Gradient Fields and Conservative Vector Fields

The script begins with an introduction to MIT OpenCourseWare and its educational resources, followed by a discussion on vector fields, specifically gradient fields. It explains that if a vector field is a gradient field, the line integral of the field is path-independent, meaning the work done depends solely on the endpoints of the path. This property is linked to the field being conservative, implying no net work is done when traversing a closed loop. The session aims to present a criterion to determine if a vector field is a gradient field and how to find the potential function if it is.

05:06

🔍 Criterion for Gradient Fields and Partial Derivatives

This paragraph delves into the criterion for identifying a gradient field, which involves checking if the mixed partial derivatives are equal, i.e., M_y equals N_x. It emphasizes the importance of this property for a vector field to be considered a gradient field. The script also introduces the concept of a converse condition, which will be explored in more detail later, and stresses the need for the vector field to be well-defined everywhere in the plane.

10:08

🌀 Exploring Non-Conservative Vector Fields with Examples

The script provides an example of a non-conservative vector field, demonstrating that it fails the gradient field test due to unequal mixed partial derivatives. It also discusses the implications of having the property M_y equals N_x only in certain regions of the plane and the potential issues that arise when the vector field is not defined everywhere, particularly in regions with holes.

15:16

📉 Determining Potential Functions for Gradient Fields

The paragraph discusses methods for finding potential functions of gradient fields. It explains that once a field has been confirmed as a gradient field, one can attempt to find the potential function, either by guessing or using systematic methods. The script mentions that there are two main methods for finding the potential, which will be detailed in subsequent lectures.

20:20

🛣️ Computing Line Integrals to Find Potential Functions

This section introduces the first method for finding potential functions by computing line integrals. It describes a process where the line integral along a path is equated to the change in potential, allowing the potential function to be determined up to an arbitrary constant. The script provides a step-by-step example of how to compute this for a given vector field, emphasizing the simplicity of choosing certain paths for the computation.

25:21

🔄 Evaluating Line Integrals Along a Broken Line Path

The script continues with the computation of line integrals, specifically along a broken line path consisting of two segments: one along the x-axis and the other along the y-axis. It simplifies the process by evaluating the integrals separately for each segment due to the constancy of one variable in each case, leading to a potential function formula that includes an integration constant.

30:26

📝 Method of Anti-derivatives for Finding Potential Functions

The paragraph introduces an alternative method for finding potential functions using anti-derivatives. It outlines a step-by-step procedure to integrate with respect to one variable and then differentiate with respect to the other to match the original vector field components. The script emphasizes the importance of careful matching and the potential pitfalls of this method.

35:38

🔧 Recap and Introduction to Curl for Vector Fields

The script recaps the concepts of gradient fields and conservative vector fields, and introduces a new concept: curl. It explains that curl, defined as N_x - M_y, measures the rotation or 'twist' in a vector field. The paragraph also discusses the physical interpretation of curl in terms of vorticity in fluid dynamics and torque in force fields.

40:42

🌪️ Physical Interpretation of Curl in Velocity and Force Fields

This section provides a deeper understanding of curl in the context of physical fields. It explains how curl measures the angular velocity of rotation in a velocity field and the torque exerted on a test object in a force field. The script also touches on the implications of curl in weather phenomena like hurricanes and tornadoes, and how the sign of curl indicates the direction of rotation.

Mindmap

Keywords

💡Vector Field

A vector field assigns a vector to each point in space, representing a direction and magnitude at that point. In the context of the video, vector fields are discussed in terms of their properties and operations, such as gradients, line integrals, and conservative fields. The script mentions that if a vector field is a gradient field, it has special properties like path independence for line integrals.

💡Line Integral

A line integral is a mathematical operation that measures the accumulated effect of a vector field along a curve. In the script, the concept is used to discuss how the work done by a force field can be computed by taking the line integral along a path, and how this integral can be path-independent if the field is conservative.

💡Gradient Field

A gradient field is a vector field that is the gradient of a scalar potential function. The script explains that if a vector field is a gradient field, the line integral of the field along any path between two points depends only on the endpoints of the path, not the path itself.

💡Path Independence

Path independence refers to the property of a line integral where the value is the same for all paths connecting two points if the vector field is conservative. The script uses this concept to describe the behavior of gradient fields, where the work done is the same regardless of the chosen path.

💡Conservative Field

A conservative field is a vector field where the line integral around any closed loop is zero. This concept is tied to the conservation of energy in physics. The script discusses how conservative fields have the property that the line integral is path independent and equals zero for closed curves.

💡Potential Function

A potential function is a scalar function whose gradient gives the vector field. In the script, the potential function is discussed as a way to find a scalar field that, when differentiated, yields the original vector field, especially when the vector field is conservative.

💡Partial Derivatives

Partial derivatives are derivatives of a function with respect to one variable, while the other variables are held constant. The script mentions that for a vector field to be a gradient field, the mixed partial derivatives must be equal, i.e., the partial derivative of the y-component with respect to x must equal the partial derivative of the x-component with respect to y.

💡Curl

Curl is a measure of the rotation or 'twist' of a vector field at a particular point. The script introduces curl as a way to determine if a vector field is conservative; if the curl is zero at every point, the field is conservative. It also explains how curl measures the intensity of rotation in a velocity field.

💡Simply Connected Region

A simply connected region is a region in the plane that does not enclose any holes. The script hints at the importance of this concept when discussing the conditions under which a vector field can be determined to be a gradient field, suggesting that if a field is defined in a simply connected region and satisfies the condition of equal mixed partial derivatives, it is a gradient field.

💡Anti-derivatives

Anti-derivatives, or indefinite integrals, are the reverse process of differentiation. The script discusses using anti-derivatives as a method to find the potential function of a vector field by integrating the components of the field with respect to the appropriate variable.

💡Integration Constant

An integration constant arises when finding anti-derivatives and represents the undetermined constant of integration. The script mentions that when finding a potential function, the integration constant can be chosen arbitrarily because it does not affect the gradient of the function.

Highlights

MIT OpenCourseWare offers high-quality educational resources for free with support from donations.

The concept of vector fields as gradient fields allows for the computation of line integrals based on potential changes between endpoints rather than path specifics.

Line integrals in gradient fields are path-independent, meaning the work done depends solely on the endpoints.

Conservative vector fields are characterized by zero work done over a closed curve, indicating energy conservation.

A criterion to determine if a vector field is a gradient field is introduced, involving the equality of mixed partial derivatives.

The importance of a vector field being well-defined everywhere for it to be a gradient field is emphasized.

A counterexample is presented where a vector field satisfies the gradient condition except at an undefined point, affecting its classification.

The notion of simply connected regions is hinted at as important for understanding the properties of vector fields.

An example demonstrates that a rotating vector field (-yi, xj) is not a gradient field due to non-matching partial derivatives.

A method to find the potential function of a gradient field by computing line integrals along specific paths is explained.

The role of integration constants in defining potential functions and their equivalence despite different constant values is discussed.

A step-by-step example illustrates how to compute the potential function for a given vector field using line integrals.

An alternative method for finding potential functions using anti-derivatives is presented as a systematic approach.

The potential function found is confirmed by taking its gradient and matching it with the original vector field.

The curl of a vector field is introduced as a measure of its rotation or vorticity, with a zero curl indicating potential conservativeness.

Physical interpretations of curl are given, relating it to the rotation in velocity fields and torque in force fields.

The significance of curl in weather phenomena like hurricanes and tornadoes is mentioned, where high curl values indicate intense rotation.

Green's theorem is previewed as a future topic that will provide more insights into curl and non-conservative properties of vector fields.

Transcripts

Browse More Related Video

Conservative Vector Fields & Potential Functions

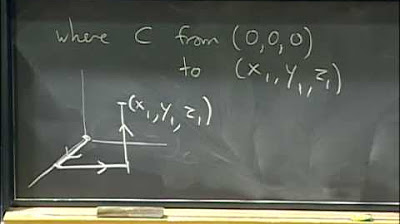
Lec 30: Line integrals in space, curl, exactness... | MIT 18.02 Multivariable Calculus, Fall 2007

Lec 20: Path independence and conservative fields | MIT 18.02 Multivariable Calculus, Fall 2007

Calculus 3: Lecture 15.1 Vector Fields

Vector Fields, Divergence, and Curl

Calculus 3: Vector Fields (Video #27) | Math with Professor V

Related Tags

Vector Fields Gradient Fields Line Integrals Potential Functions Path Independence Conservative Fields Curl Definition Rotation Measurement Educational Script Physics Analogy