Divergence theorem example 1 | Divergence theorem | Multivariable Calculus | Khan Academy

Khan Academy

26 Jun 201210:44

EducationalLearning

32 Likes 10 Comments

TLDRThe script discusses the application of the divergence theorem to simplify the calculation of the flux of a complex vector field across a solid region defined by the bounds of x, z, and y. The region's upper and lower bounds are described, and the vector field's components are detailed. The divergence of the vector field simplifies to 2x, leading to a triple integral that is calculated in a specific order of integration. After a series of integration steps, the flux is found to conveniently evaluate to zero, showcasing the power of the divergence theorem in simplifying complex surface integrals.

Takeaways

📚 The script discusses the application of the divergence theorem to simplify the calculation of the flux of a vector field across the boundary of a solid region.
📐 The region of interest is defined by x ranging from -1 to 1, with z being a function of x and bounded by 0 and an arch shape, and y bounded by 0 and a plane described by y = 2 - z.
🔍 The vector field in question is complex, involving natural logs and tangents, which makes the direct calculation of the surface integral challenging.
🌀 The divergence theorem is introduced as a method to transform the surface integral into a triple integral over the volume, involving the divergence of the vector field F.
🧩 The divergence of F is calculated to be 2x, which significantly simplifies the problem by reducing the triple integral to an integral of 2x over the volume.
📉 The integration is ordered as y first, then z, and finally x, based on the bounds of each variable within the region.
∫ The integral with respect to y is simplified by recognizing that 2x is a constant, resulting in an expression of 2x(2 - z).
📈 The subsequent integration with respect to z involves evaluating the antiderivative of the expression obtained from the y-integration, with z ranging from 0 to 1 - x^2.
📊 The final integration with respect to x is performed on the simplified expression obtained from the z-integration, with x ranging from -1 to 1.
🔢 The antiderivative of the expression with respect to x is calculated, and the limits of integration are applied to find the final result.
🎯 The surprising result of the integral is zero, indicating a special case where the flux of the vector field across the boundary of the region sums to zero.

Q & A

What is the Divergence Theorem and how does it relate to the flux across the boundary of a region?
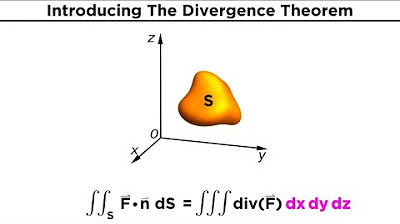
-The Divergence Theorem states that the flux of a vector field across the boundary of a region is equal to the triple integral of the divergence of the vector field over the volume of the region. It simplifies the calculation of surface integrals by converting them into volume integrals.
What is the region described in the script, and what are its boundaries in terms of x, y, and z?
-The region is a simple solid bounded by x ranging from -1 to 1, z ranging from 0 to a function of x (which forms an arch), and y ranging from 0 to 2 minus z (bounded by a plane).
What is the vector field described in the script and why is it considered 'crazy'?
-The vector field is not explicitly described in the script, but it is referred to as 'crazy' likely due to the presence of complex functions like natural logarithms and tangents, which make the surface integrals difficult to evaluate directly.
How does the script suggest simplifying the flux calculation using the Divergence Theorem?
-The script suggests calculating the divergence of the vector field first, which simplifies the flux calculation to a triple integral of the divergence over the volume of the region.
What is the calculated divergence of the vector field F in the script?
-The divergence of the vector field F is simplified to 2x after taking partial derivatives with respect to x, y, and z.
What is the order of integration suggested in the script for evaluating the triple integral?
-The suggested order of integration is y first, then z, and finally x, which corresponds to integrating over the volume of the region in the order of increasing complexity.
How does the script simplify the expression for the triple integral after calculating the divergence?
-The script simplifies the expression by recognizing that 2x is a constant with respect to y and z, and then proceeds to integrate over y, z, and x in the suggested order.
What is the final result of the triple integral after evaluating it in the script?
-After a series of integrations and simplifications, the final result of the triple integral is found to be 0.
Why does the final result of the triple integral being 0 indicate a neat simplification?
-A result of 0 suggests that the net flux of the vector field across the boundary of the region is zero, indicating a balance or cancellation of the flux in and out of the region.
What insight does the script provide about the process of evaluating surface integrals using the Divergence Theorem?
-The script demonstrates that the Divergence Theorem can significantly simplify the evaluation of surface integrals by converting them into volume integrals, especially when dealing with complex vector fields.

Outlines

00:00

📚 Introduction to Divergence Theorem Application

The video script begins with an introduction to the Divergence Theorem and its potential use in simplifying the calculation of surface integrals. The region of interest is a solid bounded by x from -1 to 1, z from 0 to a function of x, and y from 0 to 2 minus z. A complex vector field involving natural logs and tangents is given, and the task is to evaluate the flux across the boundary of this region. The Divergence Theorem is suggested as a method to simplify this task, equating the flux to a triple integral of the divergence of the vector field over the volume of the region. The divergence of the vector field is calculated, simplifying the problem to a triple integral of 2x over the specified bounds.

05:04

🔍 Detailed Calculation of the Flux Using Divergence Theorem

This paragraph delves into the specifics of calculating the flux using the Divergence Theorem. The integration is ordered by first integrating with respect to y, then z, and finally x. The bounds for each variable are clearly defined, with y ranging from 0 to 2 minus z, z from 0 to 1 minus x squared, and x from -1 to 1. The integration process involves simplifying the expression for the flux to 2x times (2 minus z), then integrating with respect to z and x. The antiderivative with respect to z is calculated, and the expression is further simplified to an integral with respect to x of a polynomial function of x, which is then integrated to find the final result.

10:07

🎯 Conclusion of Flux Calculation and Simplification to Zero

The final paragraph concludes the flux calculation, revealing that after all the integration steps and simplifications, the result of the flux integral is zero. This outcome is a neat simplification that highlights the power of the Divergence Theorem in evaluating complex surface integrals. The script confirms the correctness of the steps and the final result, emphasizing the successful application of the theorem to obtain a straightforward answer.

Mindmap

Keywords

💡Divergence Theorem

The Divergence Theorem, also known as Gauss's Theorem, is a fundamental result in vector calculus that relates the flux of a vector field through a surface to the divergence of the field in the volume enclosed by that surface. In the video, the theorem is used to simplify the calculation of the flux of a complex vector field across the boundary of a solid region by transforming it into a triple integral over the volume of the region. The script mentions the theorem as a potential method to simplify the surface integrals, which are typically complex to evaluate.

💡Vector Field

A vector field is a mathematical field that assigns a vector to each point in space. In the context of the video, the vector field is described as 'crazy' with components involving natural logarithms and tangents. The vector field is central to the problem as its flux across the boundary of the region is what needs to be evaluated using the Divergence Theorem.

💡Flux

Flux in physics and mathematics refers to the quantity of a substance or a physical property (such as heat, light, or fluid) passing through a given surface. In the video, the flux of the vector field is the quantity of interest, and it is calculated using the Divergence Theorem to transform the surface integral into a volume integral, simplifying the process.

💡Surface Integral

A surface integral is an integral taken over a surface, rather than along a curve or an area. In the script, the complexity of surface integrals is highlighted, especially when dealing with a complex vector field. The Divergence Theorem is then used to convert the surface integral into a triple integral over the volume, which is easier to handle.

💡Divergence

Divergence is a measure of how a vector field spreads out or diverges from a point. It is a scalar function that gives the magnitude of the rate at which the vector field is changing at each point. In the video, the divergence of the vector field is calculated as the first step in applying the Divergence Theorem, simplifying to 2x, which is a key step in the process.

💡Partial Derivative

A partial derivative is the derivative of a function of multiple variables with respect to one of those variables, while the others are held constant. In the script, partial derivatives are taken with respect to x, y, and z to calculate the divergence of the vector field, which is a critical step in the application of the Divergence Theorem.

💡Triple Integral

A triple integral is an integral that extends over a three-dimensional region and is used to calculate volumes, mass, or other quantities that are functions of three variables. In the video, the triple integral is used to represent the volume integral over the region, which is part of the Divergence Theorem's application to find the flux.

💡Integration Order

Integration order refers to the sequence in which the variables are integrated over in a multiple integral. In the script, the order is carefully chosen to simplify the calculation, starting with integrating over y, then z, and finally x, which is determined by the bounds of the region and the nature of the problem.

💡Antiderivative

An antiderivative, also known as an indefinite integral or primitive, is a function that represents the reverse process of differentiation. In the video, antiderivatives are found for the integrand during the process of evaluating the triple integral, which is essential for solving the problem.

💡Simplification

Simplification in mathematics refers to the process of making complex expressions or calculations more straightforward. Throughout the script, simplification is a key theme, as the Divergence Theorem is used to simplify the complex surface integral into a more manageable volume integral, and further simplifications are made in the integration process to reach the final result.

💡Infinitesimal Volume

An infinitesimal volume is an infinitesimally small volume used in the concept of integration, especially in triple integrals, to approximate the actual volume by summing up these infinitesimals. In the video, the divergence theorem involves multiplying the divergence by an infinitesimal volume (dv) to represent the contribution of each small volume element to the total flux.

Highlights

Introduction of using divergence theorem to simplify surface integrals with complex vector fields.

Description of the 3D region with bounds on x, z, and y defined by functions.

Vector field F with natural logs and tangents is given.

Flux across the boundary is equated to triple integral of divergence of F using divergence theorem.

Calculation of divergence of F simplified to 2x.

Restatement of flux as a triple integral of 2x over the region.

Integration order decided as y, then z, then x for simplification.

Integration with respect to y first, treating 2x as a constant.

Integration with respect to z next, evaluating antiderivative from 0 to 1-x^2.

Final integration with respect to x from -1 to 1.

Simplification of the integral expression to 3x - 2x^3 - x^5.

Taking the antiderivative with respect to x and evaluating from -1 to 1.

Surprising result that the integral evaluates to 0 after all simplifications.

Engaging narrative explaining the step-by-step process of applying the divergence theorem.

Demonstration of the power of divergence theorem in converting surface integrals to volume integrals.

Illustration of the methodical approach to solving complex integrals in multivariable calculus.

Highlighting the importance of correct integration order for simplification.

Emphasis on algebraic manipulation and simplification in integral calculus.

The practical application of calculus concepts in evaluating vector fields and their flux.

Educational insight into the process of setting up and solving triple integrals.

Clarification on the importance of correct variable bounds in integral setup.

Detailed walkthrough of evaluating each part of the triple integral separately.

Final simplification leading to the integral evaluating to 0, showcasing the beauty of mathematical simplification.

Transcripts

Browse More Related Video

Divergence theorem proof (part 1) | Divergence theorem | Multivariable Calculus | Khan Academy

Lec 28: Divergence theorem | MIT 18.02 Multivariable Calculus, Fall 2007

Green's Theorem (Circulation & Flux Forms with Examples)

The Divergence Theorem

Divergence example

3D divergence theorem intuition | Divergence theorem | Multivariable Calculus | Khan Academy

Divergence theorem example 1 | Divergence theorem | Multivariable Calculus | Khan Academy

Takeaways

Q & A

What is the Divergence Theorem and how does it relate to the flux across the boundary of a region?

What is the region described in the script, and what are its boundaries in terms of x, y, and z?

What is the vector field described in the script and why is it considered 'crazy'?

How does the script suggest simplifying the flux calculation using the Divergence Theorem?

What is the calculated divergence of the vector field F in the script?

What is the order of integration suggested in the script for evaluating the triple integral?

How does the script simplify the expression for the triple integral after calculating the divergence?

What is the final result of the triple integral after evaluating it in the script?

Why does the final result of the triple integral being 0 indicate a neat simplification?

What insight does the script provide about the process of evaluating surface integrals using the Divergence Theorem?