Properties of Sigma Notation and Summation Formulas | Pre-Calculus

Prof D
18 May 202111:19
EducationalLearning
32 Likes 10 Comments

TLDRThis educational video introduces viewers to the properties of sigma notation and demonstrates how to evaluate it. The instructor explains the constant multiplier property, the distribution property, and the summation at a new index. Through three examples, the video shows how to simplify sums involving sigma notation, including the summation of linear and quadratic terms. The examples cover summing constants, linear expressions, and quadratic terms, providing a clear understanding of summation properties and their applications in simplifying series.

Takeaways
  • πŸ“š The video is a tutorial on how to evaluate sigma notation using its properties.
  • πŸ”’ It introduces the constant multiplier property where 'c' times the summation of 'f(i)' from 'i=m' to 'i=n' simplifies to 'c' times the summation of 'f(i)'.
  • πŸ“ The script explains the parallelogram property, which is not detailed but implies distributing sigma notation over addition or subtraction.
  • πŸ“‰ The video demonstrates how to evaluate summation at a new index by adjusting the bounds and multiplying by the constant 'c'.
  • ⏲ It covers the summation of consecutive integers property, stating that the sum from 1 to 'n' is 'n*(n+1)/2'.
  • πŸ“ The script also explains the summation of consecutive squares property, which is 'n*(n+1)*(2n+1)/6'.
  • πŸ“ Example 1 shows the process of expanding and simplifying the summation of '4i - 5' from 1 to 30, resulting in 1710.
  • πŸ“‘ Example 2 illustrates simplifying the expression '2i - 2 + 2' from 1 to 20, which simplifies to 420.
  • πŸ“˜ Example 3 involves the summation of '3i^2 + 5' from 1 to 10, and the video shows the steps to reach the sum of 1205.
  • πŸ‘¨β€πŸ« The presenter, Prof D, encourages viewers to ask questions or seek clarifications in the comments section.
  • πŸ‘‹ The video concludes with a sign-off from Prof D, indicating the end of the tutorial.
Q & A

  • What is sigma notation?

    -Sigma notation is a way to write a sum of many terms, in a concise form using the Greek letter sigma (Ξ£).

  • What property is used to simplify the summation of c times f(i) from i = m to n?

    -The property used is that the summation of c times f(i) from i = m to n equals c times the summation of f(i) from i = m to n.

  • How do you handle a constant term in sigma notation?

    -When dealing with a constant term in sigma notation, it can be factored out of the summation.

  • What is the formula for the summation of i from 1 to n?

    -The formula for the summation of i from 1 to n is n(n + 1) / 2.

  • How is the summation of i squared from 1 to n evaluated?

    -The summation of i squared from 1 to n is evaluated using the formula n(n + 1)(2n + 1) / 6.

  • What is the result of summing 4i - 5 from 1 to 30?

    -The summation of 4i - 5 from 1 to 30 equals 1710.

  • How do you simplify the summation of 2 times (i - 1) + 2 from 1 to 20?

    -First, distribute the terms inside the summation, then use the properties of sigma notation to simplify. The result is 420.

  • What is the result of summing 3i squared + 5 from 1 to 10?

    -The summation of 3i squared + 5 from 1 to 10 equals 1205.

  • How do you handle the summation of a binomial expression?

    -Each term in the binomial expression can be summed separately using the properties of sigma notation.

  • What should you do if you have questions or clarifications about the video content?

    -If you have questions or clarifications, you can put them in the comments section below the video.

Outlines
00:00
πŸ“š Introduction to Sigma Notation and Its Properties

This paragraph introduces the video's focus on evaluating sigma notation using its properties. The instructor begins by explaining the summation notation of c times f(i) from i=m to i=n, emphasizing that 'c' is a constant. The properties discussed include the handling of constants within the summation and the distribution of sigma notation over addition and subtraction. A brief mention of evaluating summation at a new term and the constant factor property is also made, setting the stage for the examples that will follow.

05:03
πŸ” Detailed Examples of Sigma Notation Evaluation

The instructor proceeds with three detailed examples to demonstrate the application of sigma notation properties. The first example involves expanding and simplifying the summation of 4i minus 5 from i=1 to i=30, using the properties of summation to simplify the expression to 1710. The second example shows the summation of 2 times (i - 1 + 2) from i=1 to i=20, simplifying to 420 by applying the summation properties. The third example tackles the summation of 3i^2 plus 5 from i=1 to i=10, which is simplified using the formula for the summation of i^2, resulting in a final answer of 1205. Each example is carefully explained, highlighting the process of simplification and the use of sigma properties.

10:03
πŸŽ‰ Conclusion and Invitation for Feedback

The video concludes with a summary of the lessons covered on evaluating sigma notation and an invitation for viewers to ask questions or seek clarifications in the comments section. The instructor, referred to as 'Prof D', thanks the viewers for watching and signs off with a friendly 'catch you on the flip side, bye', indicating the end of the educational content and encouraging viewer interaction.

Mindmap
Keywords
πŸ’‘Sigma Notation
Sigma notation, represented by the Greek letter Ξ£, is a mathematical notation used to represent the sum of a sequence of terms. In the video, sigma notation is the central theme, as the host demonstrates how to evaluate and simplify summations using various properties. For example, the script mentions 'summation notation of c times f, of i from i equals m to n', illustrating the basic structure of sigma notation where 'c' is a constant and 'f(i)' is a function of 'i' that sums over a range from 'm' to 'n'.
πŸ’‘Constant
In mathematics, a constant is a value that does not change. In the context of the video, constants are coefficients that multiply the function within the sigma notation. The script explains that when a term is constant, it can be factored out of the summation, simplifying the expression. For instance, 'c times the summation of f of i, from i equals m to n' shows how a constant 'c' can be factored out and then multiplied by the sum of 'f(i)' over the range.
πŸ’‘Summation Properties
Summation properties refer to the rules and patterns that can be applied to simplify expressions within sigma notation. The video discusses several properties, such as the constant multiple property and the distributive property, which allow for the simplification of summations. For example, the script states 'if the term nothing is composed of two or more terms, to guide it over a whether nothing is a, distribute you're adding sigma notation', indicating the use of properties to break down complex summations into simpler components.
πŸ’‘Parallelogram Property
The parallelogram property is a specific summation property that allows for the manipulation of summations that are composed of multiple terms. The video mentions this property when discussing how to handle summations with more than one term, suggesting that it can be used to distribute the summation over each term individually. However, the script does not provide a detailed example of this property, so it may refer to a more general approach to handling complex summations.
πŸ’‘Evaluation
Evaluation in the context of summations means finding the numerical value of the sum represented by sigma notation. The video provides several examples of how to evaluate summations by applying different properties. For instance, the script shows the evaluation of 'summation of 4i, minus 5 from 1 to 30' by first applying the constant multiple property and then using the formula for the sum of the first 'n' natural numbers to find the result.
πŸ’‘Distributive Property
The distributive property is a fundamental property of arithmetic that allows for the distribution of multiplication over addition or subtraction. In the video, this property is applied to sigma notation to simplify expressions where a constant is multiplied by a sum of terms. The script mentions 'distribute you're adding sigma notation', which refers to the process of distributing the summation over each term within the summation.
πŸ’‘Example Problems
Example problems are used in educational content to illustrate how to apply concepts and solve for specific cases. The video includes several example problems that demonstrate the application of summation properties to evaluate sigma notation. For example, 'example number one expand the following sums and simplify if possible number one the summation of 4i, minus 5 from 1 to 30' shows how to apply the properties to find the sum of a series of terms.
πŸ’‘Simplification
Simplification in mathematics refers to the process of making an expression easier to understand or calculate. In the context of the video, simplification involves using summation properties to reduce complex summations to a more manageable form. The script demonstrates this with expressions like '4 times 465, minus 150', which simplifies to '1710', showing the process of simplifying a summation to its final numerical value.
πŸ’‘Summation Formulas
Summation formulas are mathematical formulas used to calculate the sum of a series of numbers or terms within a specific range. The video mentions several formulas, such as the sum of the first 'n' natural numbers and the sum of the squares of the first 'n' natural numbers. For example, the script uses 'summation of i, from 1 to n which is equals to n times n plus one over 2' to calculate the sum of the first 'n' natural numbers, which is a common formula in summation problems.
πŸ’‘Educational Content
Educational content is material designed to teach or inform an audience about a particular subject. The video script is an example of educational content, as it aims to instruct viewers on how to evaluate sigma notation using its properties. The host, 'prof d', provides explanations and examples to help viewers understand the concepts being taught, which is a characteristic of educational content.
Highlights

Introduction to evaluating sigma notation using its properties.

Explanation of the constant multiplier property in summation notation.

Demonstration of the parallelogram property for summation notation.

Guidance on distributing sigma notation when terms are composed of multiple parts.

Illustration of summation properties for evaluating at a new variable and constant.

Application of summation properties to simplify expressions involving constants.

Example of summation of i from 1 to n and its formulaic representation.

Example of summation of i squared from 1 to n and its formulaic representation.

Step-by-step walkthrough of example one: summation of 4i minus 5 from 1 to 30.

Simplification of the summation in example one to arrive at the final answer.

Presentation of example two: summation of 2 times (i - 1 + 2) from 1 to 20.

Explanation of how to simplify the summation in example two to find the result.

Introduction of example three: summation of 3i squared plus 5 from 1 to 10.

Detailed breakdown of the summation in example three using sigma properties.

Final calculation and conclusion of the summation in example three.

Summary of the video's content and the ability to evaluate sigma notation using its properties.

Invitation for viewers to ask questions or seek clarifications in the comments section.

Closing remarks and sign-off from Prof D.

Transcripts

Browse More Related Video

PROPERTIES OF SIGMA NOTATION || PRE-CALCULUS

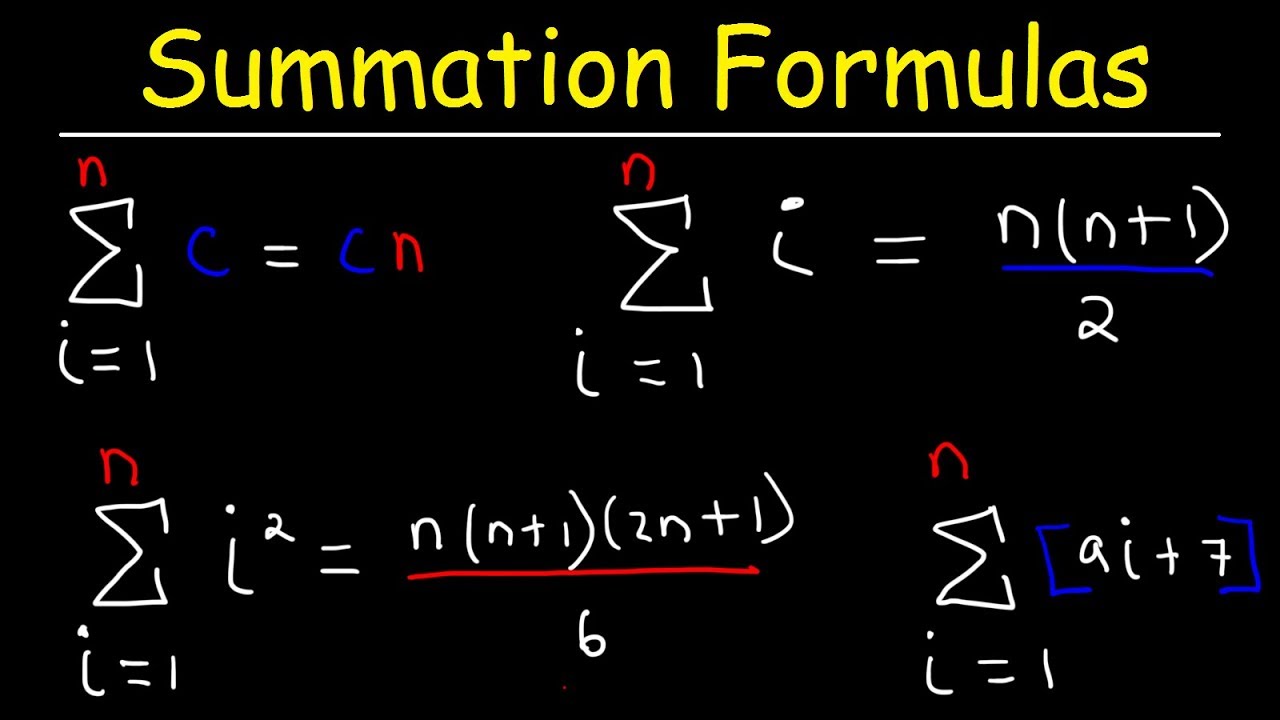
Summation Formulas and Sigma Notation - Calculus

Calculus 1 Lecture 4.3: Area Under a Curve, Limit Approach, Riemann Sums

Writing Summations in Sigma Notation | Series and Sigma Notation | Pre-Calculus

Sigma notation for sums | Sequences, series and induction | Precalculus | Khan Academy

AP Calculus BC Lesson 10.1

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Sigma NotationMath TutorialEducational VideoSummation PropertiesCalculus TechniquesMathematicsEducation ChannelProblem SolvingSeries ExpansionNumerical Methods