Motion | Distance and Displacement | Physics | Infinity Learn

Infinity Learn NEET
4 May 201703:56
EducationalLearning
32 Likes 10 Comments

TLDRThe video script explores the concepts of distance and displacement, two fundamental measures in physics. Distance, measured in meters (or kilometers), is the total length of a path traveled, regardless of direction, making it a scalar quantity. In contrast, displacement is the straight-line distance from the starting point to the final position, including direction, thus it is a vector quantity. The script illustrates these concepts through everyday examples, such as taking a detour due to road works, which increases the distance traveled but not the displacement. It also humorously points out that taxis charge based on distance, not displacement, even if the passenger ends up at the starting point after a round trip. The summary effectively communicates the key differences between distance and displacement, using relatable scenarios to engage viewers and enhance their understanding of these physical concepts.

Takeaways
  • 📏 Distance is the total length of the path traveled, irrespective of direction.
  • ↗️ The shortest path between two points is a straight line, known as the best route.
  • 🚧 Detours increase the distance traveled due to obstacles or road works.
  • 📏 Distance is measured in meters ('m') and can also be expressed in kilometers ('km').
  • 🔢 A detour can significantly increase the distance from the direct path.
  • 📍 Displacement is the vector quantity that includes both the distance and direction traveled.
  • 🔄 Even if a person travels a long distance in a loop, the displacement is zero if they return to the starting point.
  • 🧭 Displacement specifies direction, making it different from distance.
  • 🚖 Taxis charge based on the distance traveled, not the displacement.
  • 🔄 The distance covered in everyday activities is often greater than the displacement due to the nature of back-and-forth movements.
  • 💭 Understanding the difference between distance and displacement is crucial for grasping fundamental concepts in physics.
Q & A

  • What is the definition of distance?

    -Distance is the measure of the total length covered when moving from one place to another.

  • Why did the travelers have to take a detour in the given example?

    -The travelers had to take a detour due to road works along the best route, which was straight up north.

  • How is distance typically measured?

    -Distance is typically measured in meters and is denoted by the lower case 'm'.

  • What is the relationship between kilometers and meters?

    -A kilometer is equivalent to one thousand meters.

  • Why is distance considered a scalar quantity?

    -Distance is a scalar quantity because it does not specify direction, only the magnitude of how much ground has been covered.

  • What is displacement and how does it differ from distance?

    -Displacement is 'distance with direction'. It differs from distance in that it includes the direction of travel, making it a vector quantity.

  • How can one cover a large distance with zero displacement?

    -One can cover a large distance with zero displacement by returning to the starting point after traveling, such as running back and forth and ending up in the same spot.

  • In what scenario would the magnitude of distance and displacement be the same?

    -The magnitude of distance and displacement would be the same if the traveled path is a straight line, as the displacement would then include both the distance and the direction.

  • Why is the distance covered often greater than the displacement in everyday life?

    -The distance covered is often greater than the displacement because people frequently travel back and forth between places, accumulating more distance without increasing the displacement from the starting point.

  • How are taxi fares typically calculated?

    -Taxis charge passengers based on the distance traveled, not on the displacement.

  • What would be the taxi fare if a passenger goes to a neighboring town and returns in the same taxi?

    -The taxi fare would be calculated based on the entire round trip distance, regardless of the passenger's displacement being zero.

  • Why is it important to understand the difference between distance and displacement?

    -Understanding the difference between distance and displacement is important for grasping fundamental concepts in physics, and it helps in comprehending real-world applications such as travel, navigation, and understanding the nature of motion.

Outlines
00:00
📏 Understanding Distance and Displacement

This paragraph explains the concept of distance as the total length traveled between two points, which can be affected by factors such as road works, leading to a detour and increased distance covered. Distance is measured in meters ('m') and can also be expressed in kilometers (1 km = 1000 m). The example given is a journey between two towns, initially 20,000 meters apart, but due to a detour, the distance traveled becomes 50,000 meters or 50 kilometers. The paragraph distinguishes distance as a scalar quantity because it lacks direction. It then introduces displacement as 'distance with direction,' emphasizing that displacement is a vector quantity because it includes direction and results in a specific location. The concept is further illustrated with an example of a child running back and forth but returning to the starting point, thus covering a lot of distance but having zero displacement. The paragraph concludes by noting that in everyday life, the distance traveled is usually greater than the displacement, and it poses a question about how taxis charge passengers, which is based on the distance covered, not displacement.

Mindmap
Keywords
💡Distance
Distance is the total length of the path traveled between two points, regardless of the direction. It is a scalar quantity, meaning it only has magnitude and does not include direction. In the video, the concept is illustrated by the example of traveling to a town, where a detour due to road works increases the total length traveled from 20,000 meters to 50,000 meters, or from 20 kilometers to 50 kilometers.
💡Detour
A detour is an alternate route taken when the usual path is obstructed or blocked, such as road works. The video uses the concept of a detour to demonstrate how the actual distance traveled can be greater than the direct distance between two points, thus affecting the total distance covered.
💡Scalar Quantity
A scalar quantity is a physical quantity that has magnitude but no direction. In the context of the video, distance is referred to as a scalar because it does not specify the path taken or the direction of travel. The video emphasizes this by pointing out that knowing someone traveled 20 kilometers does not indicate their final position or direction.
💡Displacement
Displacement is the straight-line distance between the starting and ending points of a journey, including the direction of travel. It is a vector quantity, which means it has both magnitude and direction. The video explains that while the distance covered in two separate trips to a town might differ, the displacement remains the same if the starting and ending points are identical, illustrating that displacement is direction-sensitive.
💡Vector Quantity
A vector quantity has both magnitude and direction, unlike a scalar quantity. Displacement is introduced in the video as a vector quantity because it specifies the direction of travel in addition to the distance covered. The video uses the example of traveling 20 kilometers north to a town to illustrate that the direction 'north' is a critical component of displacement.
💡Kilometer
A kilometer is a unit of length equal to one thousand meters. The video uses kilometers to simplify the discussion of large distances. It explains that instead of saying 20,000 meters, one can say 20 kilometers, making it easier to understand and communicate the distance covered.
💡Direction
Direction refers to the course along which someone or something moves or is aimed to move. In the video, direction is a key factor in distinguishing between distance and displacement. The inclusion of direction in displacement makes it a vector quantity, as opposed to distance, which lacks direction and is therefore a scalar.
💡Meters
Meters are the base unit of length in the International System of Units (SI). The video uses meters as the primary unit to measure distance and displacement, with the example given that the distance between two towns is about twenty thousand meters.
💡Taxi Fare
Taxi fare is the charge for a journey taken in a taxi, which is typically calculated based on the distance traveled. The video uses the example of a taxi fare to highlight that in everyday life, charges are based on the total distance of the trip, not the displacement, even if the starting and ending points are the same.
💡Round Trip
A round trip is a journey that returns to the starting point. The video discusses a scenario where a passenger takes a taxi for a round trip to a neighboring town and is charged for the entire distance of the trip. This serves to emphasize that in practical terms, distance is the relevant measure for calculating fares, not displacement.
💡Resultant Change
Resultant change refers to the final outcome or effect after all actions are considered. In the context of the video, the child in the example has zero displacement because they returned to the original spot despite covering a significant distance by running back and forth. This illustrates the difference between the total distance covered and the displacement from the starting point.
Highlights

Distance is the measure of the total length covered when moving from one place to another.

Distance is measured in meters and denoted as 'm'.

A kilometer is equal to one thousand meters.

Distance is a scalar quantity as it does not specify direction.

Displacement is defined as 'distance with direction'.

Displacement is a vector quantity because it includes direction.

In the given example, the straight path and detour have different distances but the same displacement.

The child in the example covered lots of distance but had zero displacement since he returned to the starting point.

If the distance traveled is a straight line, the magnitude of displacement will be the same as the distance, with direction included.

In most everyday situations, the distance covered is greater than the displacement.

Taxis charge passengers based on the distance traveled, not displacement.

Even if a passenger returns to their starting point, they are still charged for the entire round trip distance.

Distance and displacement are fundamental concepts in physics with practical implications in everyday scenarios like travel.

Understanding the difference between distance and displacement is crucial for grasping basic physics concepts.

The example of the child running back and forth illustrates the concept of distance versus displacement in a relatable way.

The concept of displacement is introduced through the practical example of traveling to a town and taking a detour.

The transcript uses clear, everyday examples to explain the abstract concepts of distance and displacement.

The importance of specifying direction when defining displacement is emphasized.

The transcript concludes with a thought-provoking question about taxi fares and the concepts of distance and displacement.

Transcripts

Browse More Related Video

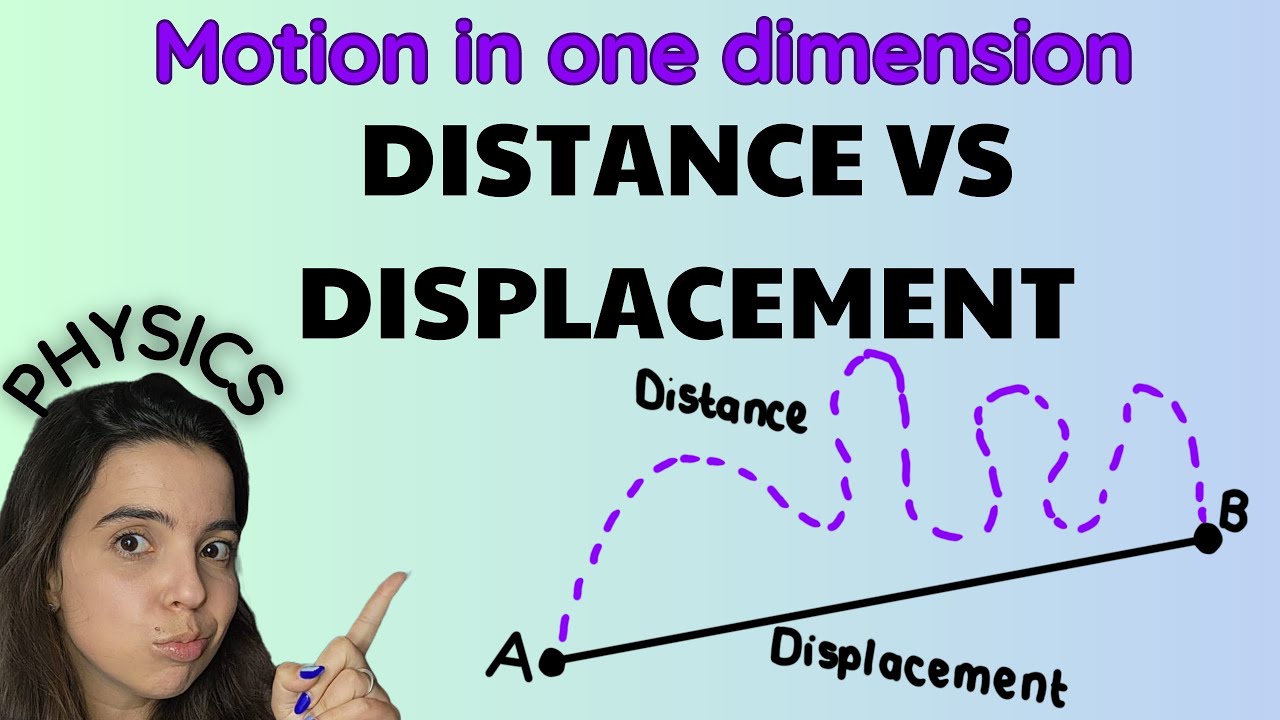
Displacement and Distance: Motion in One Dimension

Displacement and Velocity - How is it different from Distance and Speed? | Physics

Distance vs. Displacement & Speed vs. Velocity | Kinematics Explained

DISTANCE & DISPLACEMENT | Physics Animation

What Are Distance and Displacement? | Physics in Motion

Distance and Displacement: what are they and what's the difference

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
PhysicsDistanceDisplacementDirectionScalarVectorTravelMetersKilometersRoadworksDetourTaxisCharge