Conservation of Angular Momentum | Physics with Professor Matt Anderson | M12-18

Physics with Professor Matt Anderson
1 Nov 202105:34
EducationalLearning
32 Likes 10 Comments

TLDRIn this educational transcript, Professor Anderson delves into the concept of angular momentum and its conservation. He explains that, much like linear momentum, angular momentum is conserved in the absence of external torques. Using the classic example of a ball on a string, he illustrates how the speed of the ball increases as the string's length is reduced. The principle is that the initial and final angular momenta must be equal, leading to the conclusion that when the radius is halved, the speed of the ball doubles. This demonstration effectively conveys the foundational principle of angular momentum conservation and its practical implications, offering a clear and engaging explanation that is both informative and accessible.

Takeaways
  • 📐 **Conservation of Angular Momentum**: Angular momentum (L) is conserved in a system when there are no external torques acting on it.
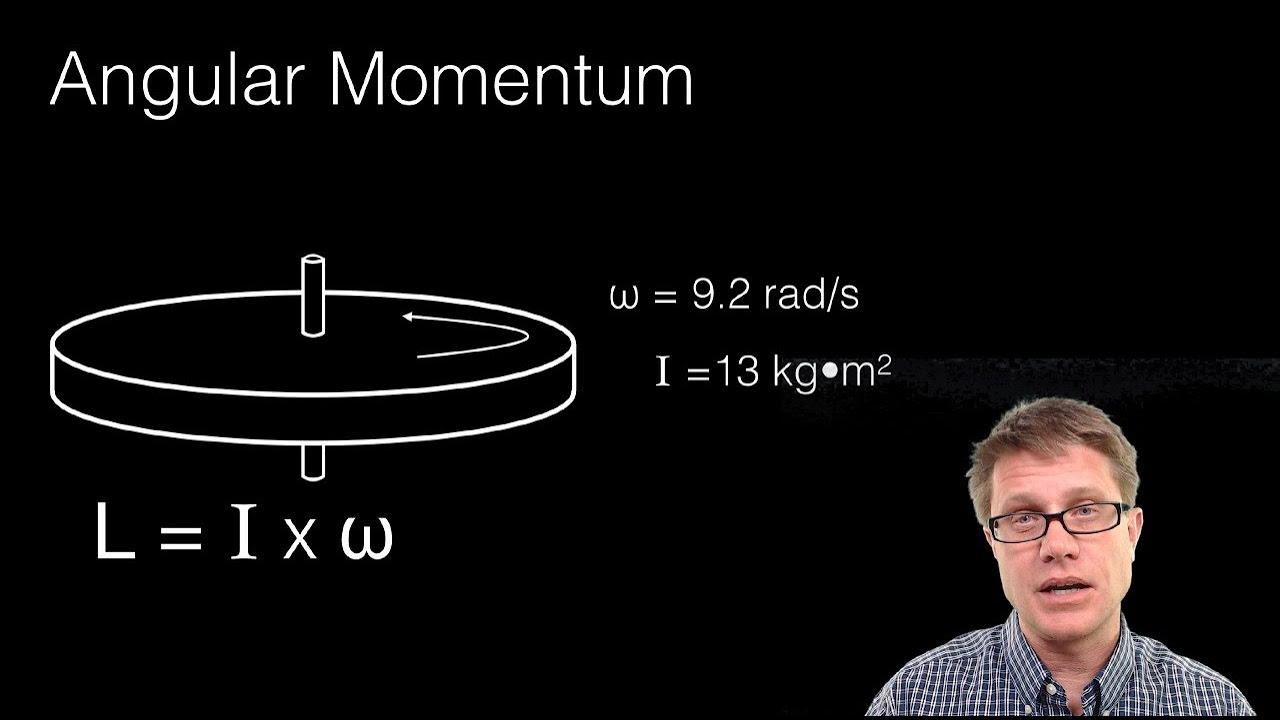
  • 🔄 **Definition of Angular Momentum**: Angular momentum is a vector quantity, represented by L, and is the rotational equivalent of linear momentum.
  • ⚫ **System Consideration**: The initial and final states of the entire system must be considered to apply the conservation of angular momentum.
  • 🧵 **Ball on a String Example**: The example of a ball on a string is used to illustrate the principles of angular momentum.
  • 🏃 **Effect of Changing Radius**: When the radius (r) of the circular path of the ball is reduced, the speed (v) of the ball increases, following the conservation of angular momentum.
  • 🔢 **Mathematical Relationship**: The final speed (v_final) is twice the initial speed (v_initial) when the radius is halved, calculated as v_final = (r_initial / r_final) * v_initial.
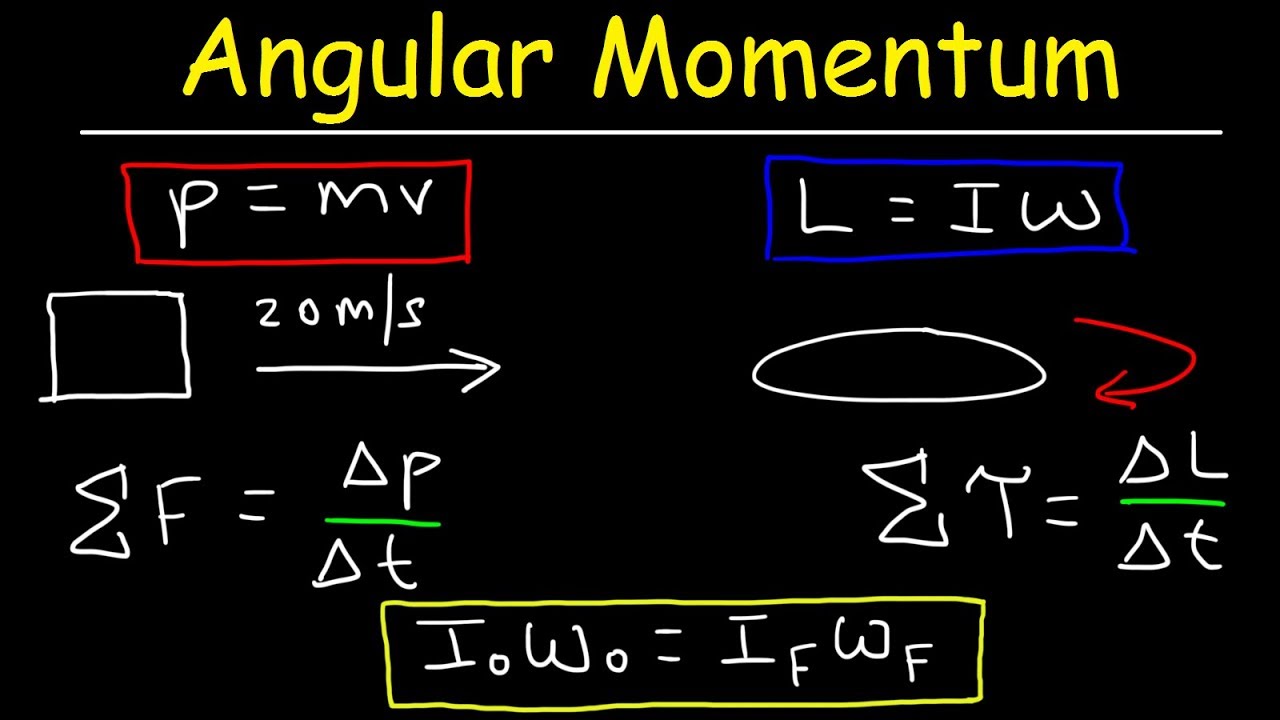
  • ⚖️ **Conservation Equation**: The initial angular momentum (L_initial) must equal the final angular momentum (L_final), L_initial = L_final.
  • 🚀 **Increasing Speed with Decreasing Radius**: As the radius decreases, the object's speed increases, following the conservation of angular momentum.
  • ∞ **Extreme Case**: In the limit, as the radius approaches zero, the speed of the object would theoretically approach infinity, similar to the effect seen with black holes.
  • 🤔 **Practical Implications**: The concept is important for understanding phenomena such as the increasing spin of a figure skater as they pull their arms in closer to their body.
  • 📚 **Further Inquiry**: Professor Anderson encourages students to approach them for clarification if the concept is not clear, emphasizing the importance of understanding the principles discussed.
Q & A

  • What is the fundamental principle discussed in the script?

    -The fundamental principle discussed in the script is the conservation of angular momentum.

  • Under which condition is angular momentum conserved?

    -Angular momentum is conserved when there are no external torques acting on the system.

  • What does the conservation of angular momentum imply for a system?

    -The conservation of angular momentum implies that the initial angular momentum (L_initial) must equal the final angular momentum (L_final) for the system.

  • What is the formula for angular momentum in the context of a ball on a string?

    -In the context of a ball on a string, the formula for angular momentum is mvr, where m is the mass, v is the velocity, and r is the radius of the circular path.

  • What happens to the speed of the ball when the length of the string is halved, according to the conservation of angular momentum?

    -When the length of the string is halved, the speed of the ball doubles, as long as there are no external torques acting on the system.

  • What is the implication of the angular momentum conservation principle in the example of the ball on a string?

    -The implication is that as the radius (r) decreases, the velocity (v) of the ball must increase to maintain the same angular momentum, leading to a faster spinning ball.

  • How does the mass of the ball affect the final velocity when the string is shortened?

    -The mass of the ball (m) does not affect the final velocity in this scenario because it remains constant and cancels out in the equation mv_initial * r_initial = mv_final * r_final.

  • What would happen to the speed of the ball if the string is continuously shortened?

    -If the string is continuously shortened, the speed of the ball would increase indefinitely, following the principle that v_final = (r_initial / r_final) * v_initial.

  • What is the analogy used in the script to describe what happens when an object is pulled into a black hole?

    -The analogy used is that as an object gets sucked into a black hole, it starts spinning faster and faster, similar to how the ball's speed increases as the string is shortened.

  • What would be the final speed of the ball if the string's length approaches zero?

    -In the limit, as the string's length approaches zero, the final speed of the ball would theoretically approach infinity, indicating an infinitely fast spinning ball.

  • Why is it important to consider the entire system when applying the conservation of angular momentum?

    -It is important to consider the entire system to account for all the contributing factors to the angular momentum. This ensures that the principle of conservation is accurately applied and the calculation of initial and final angular momentum is correct.

  • Can the conservation of angular momentum be applied to systems where there are external torques?

    -No, the conservation of angular momentum principle strictly applies to systems where there are no external torques. If external torques are present, the angular momentum of the system will change.

Outlines
00:00
📚 Introduction to Angular Momentum Conservation

Professor Anderson introduces the concept of angular momentum and its conservation. He explains that, similar to linear momentum, angular momentum is conserved in the absence of external torques. The professor uses the example of a ball on a string to illustrate how angular momentum is maintained even when the radius of the spinning circle changes. When the string's length is halved, the speed of the ball doubles, demonstrating the conservation of angular momentum.

05:03
🔄 Effect of Radius on Angular Velocity

This paragraph delves into the relationship between the radius of rotation and the velocity of the spinning object. As the radius decreases, the angular velocity increases. The professor illustrates this by showing that if the radius is reduced to a third, the velocity would triple. He takes the concept to the extreme, suggesting that as the radius approaches zero, the velocity would theoretically become infinite, drawing a parallel to the increasing spin of matter as it falls into a black hole.

Mindmap
Keywords
💡Angular Momentum
Angular momentum is a vector quantity that represents the rotational equivalent of linear momentum in physics. It is defined as the product of the moment of inertia and the angular velocity of an object. In the context of the video, angular momentum is a key principle that is conserved, meaning that if there are no external torques acting on a system, its angular momentum remains constant. This is illustrated by the ball on a string example, where the angular momentum is conserved even when the string's length changes.
💡Conservation of Angular Momentum
The conservation of angular momentum is a fundamental principle in physics that states that the total angular momentum of a closed system remains constant if no external torques are applied. This principle is central to the video's discussion, as it is used to explain the behavior of the ball on a string when the string's length is altered. The conservation law is demonstrated through the formula \( L_i = L_f \), where \( L \) represents angular momentum.
💡Torque
Torque is the measure of the force that can cause an object to rotate around an axis. It is a vector quantity represented as the cross product of the radius vector from the axis of rotation to the point of force application and the force vector. In the video, torque is mentioned in the context of it being a factor that, if absent, allows for the conservation of angular momentum. The absence of external torques is what allows the ball on a string to maintain its angular momentum as it changes speed when the string's length is altered.
💡Linear Momentum
Linear momentum is the product of an object's mass and its velocity and is a vector quantity that describes the motion of an object in a straight line. Although the video focuses on angular momentum, linear momentum is mentioned as a foundational principle that is also conserved in collisions. The concept helps to contrast with angular momentum, emphasizing the difference between linear and rotational motion.
💡Elastic and Inelastic Collisions
Elastic and inelastic collisions refer to two types of collisions in physics. In an elastic collision, both momentum and kinetic energy are conserved, while in an inelastic collision, only momentum is conserved, and kinetic energy is not. The video briefly mentions these types of collisions to highlight the conservation of momentum, which is a prerequisite for discussing angular momentum.
💡Vector
A vector is a quantity that has both magnitude and direction. In the context of the video, both linear momentum and angular momentum are described as vectors. The vector nature of angular momentum is important because it includes the direction of rotation, which is crucial for understanding how angular momentum is conserved when the string's length changes in the ball on a string example.
💡Moment of Inertia
The moment of inertia is a measure of an object's resistance to changes in its rotation. It is a scalar quantity that depends on the mass of the object and the distribution of that mass. In the video, the moment of inertia is implicitly involved in the calculation of angular momentum for the ball on a string, as it is part of the formula \( L = mvr \), where \( m \) is mass, \( v \) is velocity, and \( r \) is the radius of rotation.
💡Ball on a String
The ball on a string is a classic physics example used to illustrate concepts of circular motion and conservation of angular momentum. In the video, this example is used to demonstrate what happens to the speed of the ball when the string's length is reduced. The example shows that as the radius \( r \) decreases, the speed \( v \) of the ball increases to conserve angular momentum.
💡Black Hole
A black hole is mentioned in the video as an analogy to describe what happens when an object gets closer to a point of rotation, like the ball on a string when the string's length is reduced. As the object gets closer to the axis of rotation, it spins faster, similar to how matter accelerates as it approaches a black hole. This analogy helps to visualize the principle of conservation of angular momentum in an extreme scenario.
💡External Forces
External forces are forces that act on a system from the outside. In the context of the video, the absence of external forces (or torques, in the case of angular momentum) is a condition for the conservation of angular momentum. The video emphasizes that if no external torques act on a spinning system, its angular momentum remains constant.
💡System
In the video, a system refers to the set of objects or particles being studied in the context of physics. The conservation of angular momentum is discussed in relation to a system, such as the ball on a string. The system's initial and final states are compared to determine if angular momentum is conserved, which is crucial for understanding the behavior of the system under study.
Highlights

Angular momentum is a foundational principle in physics, similar to the conservation of momentum.

Conservation of angular momentum applies to both elastic and inelastic collisions.

Angular momentum is conserved when there are no external torques acting on a system.

The conservation of angular momentum is represented by the equation L_initial = L_final.

The entire system must be accounted for in the calculation of angular momentum.

A simple example involving a ball on a string is used to illustrate the concept.

When the length of the string is halved, the speed of the ball doubles.

The angular momentum of a ball on a string is given by the formula mvr.

The mass (m) remains constant and cancels out in the final calculation.

The final speed (v_final) is calculated as v_initial multiplied by the ratio of the initial radius to the final radius.

In the example, as the radius is reduced to half, the speed increases by a factor of two.

If the radius continues to decrease, the speed increases proportionally.

In the limit, as the radius approaches zero, the speed would theoretically become infinite.

The phenomenon is likened to the increasing spin of matter as it falls into a black hole.

The example provides a clear understanding of the conservation of angular momentum in a practical scenario.

The lecture encourages students to seek clarification if they have any doubts about the concept.

The analogy of a ball on a string is a relatable and easy-to-understand model for explaining complex physics principles.

The lecture emphasizes the importance of considering the entire system when applying the conservation laws.

Transcripts

Browse More Related Video

Rotational Energy | Physics with Professor Matt Anderson | M12-17

Conservation of Angular Momentum

What Is Angular Momentum?

Angular Momentum - Basic Introduction, Torque, Inertia, Conservation of Angular Momentum

What IS Angular Momentum?

Angular Momentum of a Spinning Wheel | Physics with Professor Matt Anderson | M12-16

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Angular MomentumConservation LawsPhysics EducationBall on StringScientific PrincipleMomentum DynamicsEducational ContentPhysics ConceptsLecture SeriesProfessor AndersonPhysical Phenomena