Spinning Ice Skater | Physics with Professor Matt Anderson | M12-02

Physics with Professor Matt Anderson
1 Nov 202104:42
EducationalLearning
32 Likes 10 Comments

TLDRIn the video script, the presenter explores a physics problem related to an ice skater's spin, using a top view illustration of Brian Boitano as an example. The skater is spinning at a rate of 100 revolutions per minute (rpm), and the task is to calculate the speed of the skater's hands. To do this, the presenter uses the formula v = ω × r, where v is the speed, ω is the angular velocity, and r is the radius of the spin (in this case, the length of the skater's arm). The arm's length is approximated as 70 centimeters. Converting 100 rpm to radians per second, the presenter calculates the angular velocity, then multiplies it by the arm's length in meters to find the hand's speed. The final calculation results in a hand speed of approximately 7.3 meters per second, which is roughly equivalent to 15 miles per hour, providing a relatable and engaging explanation of the physics involved in a spinning skater's motion.

Takeaways
  • 🎓 The problem involves calculating the speed of an ice skater's hands while spinning.
  • 🧍 The skater is represented by Brian Boitano in the example, with a humorous note about his hands.
  • 🌀 The skater's spinning speed is given as 100 revolutions per minute (rpm).
  • 🔢 To find the speed, a relationship between velocity (v), angular velocity (omega), and radius (r) is used: v = omega × r.
  • ⏱️ The script converts rpm to radians per second, which is necessary for the calculation.
  • 📏 The length of the skater's arm is approximated as 70 centimeters for the calculation.
  • 📐 One revolution is equivalent to 2π radians, which is used to convert rpm to radians per second.
  • 🧮 The calculated angular velocity in radians per second is 100 × 2π / 60.
  • 📐 The speed of the hand is then found by multiplying the angular velocity by the length of the arm in meters (0.7 meters).
  • 🧮 The approximate speed of the hand is calculated to be around 7.3 meters per second.
  • 🚀 This speed is roughly equivalent to 15 miles per hour, which is presented as a reasonable figure.
  • 📝 The process involves unit conversion and the application of physics formulas to solve a real-world problem.
Q & A

  • What is the problem being discussed in the script?

    -The problem being discussed is calculating the speed of an ice skater's hands while spinning, using Brian Boitano as an example.

  • What is the initial spinning speed given for the ice skater?

    -The initial spinning speed given is 100 revolutions per minute (rpm).

  • How is the relationship between speed (v), angular velocity (omega), and radius (r) expressed?

    -The relationship is expressed as v = omega * r, where v is the speed, omega is the angular velocity, and r is the radius.

  • What is the unit conversion needed to calculate the angular velocity in radians per second?

    -To convert from rpm to radians per second, you multiply by 2π and divide by 60 seconds.

  • What is the length of the arm used in the approximation?

    -The length of the arm used in the approximation is 70 centimeters.

  • How is the length of the arm converted for the calculation?

    -The length of the arm is converted from centimeters to meters by dividing by 100, resulting in 0.7 meters.

  • What is the final calculated speed of Brian Boitano's hands in meters per second?

    -The final calculated speed of the hands is approximately 7.3 meters per second.

  • How is the final speed converted to miles per hour?

    -The final speed is converted to miles per hour by recognizing that 7.3 meters per second is roughly equivalent to 15 miles per hour.

  • What is the significance of using SI units in the calculation?

    -Using SI units (Système International d'unités) ensures that the units are consistent and standardized, which is crucial for accurate scientific calculations.

  • Why is it important to cancel out units during the calculation?

    -Canceling out units is important to simplify the expression and to ensure that the final answer is in the correct units for the physical quantity being calculated.

  • What is the role of pi (π) in the calculation of angular velocity?

    -Pi (π) is used to convert revolutions to radians since one full revolution is equal to 2π radians.

  • How does the script demonstrate the application of physics to a real-world scenario?

    -The script demonstrates the application of physics by using the principles of circular motion to calculate the speed of a moving object (the ice skater's hands) during spinning.

  • What is the relevance of this problem to a physics class?

    -This problem is relevant to a physics class as it involves the application of concepts like angular velocity, linear speed, and unit conversion, which are fundamental to understanding circular motion.

Outlines
00:00
🧊 Calculating the Speed of a Spinning Ice Skater's Hands

The paragraph introduces a physics problem related to an ice skater, specifically focusing on the speed of the skater's hands as they spin. The skater, humorously referred to as having 'ridiculously large hands', is spinning at a rate of 100 revolutions per minute (rpm). To determine the speed of the hands, the length of the skater's arm is approximated to be 70 centimeters. Using the relationship between velocity (v), angular velocity (omega), and radius (r), where v = omega * r, the angular velocity is first converted from rpm to radians per second. The calculation involves multiplying 100 by 2 pi and dividing by 60 to get the angular velocity in radians per second. The speed of the hand is then found by multiplying this angular velocity by the length of the arm in meters (0.7 m). After a quick calculation, the speed is approximated to be around 7.3 meters per second, which is roughly equivalent to 15 miles per hour.

Mindmap
Keywords
💡Ice Skater
An ice skater is an individual who performs on ice using ice skates. In the context of the video, the ice skater, Brian Boitano, is used as an example to illustrate the physics of spinning motion. The skater's spinning is central to the problem being discussed, which involves calculating the speed of the skater's hands as they spin.
💡Spinning
Spinning refers to the rotational motion of an object around an axis. In the video, the spinning motion is applied to the ice skater's body as they rotate on the ice. The concept is crucial to understanding the physics problem, which involves calculating the speed of the skater's hands during the spin.
💡Top View
A top view is a perspective from above, looking down on an object or scene. The video uses the term to describe the visual representation of the ice skater spinning, which helps to set the scene for the physics problem and to visualize the motion being analyzed.
💡Revolutions per Minute (RPM)
Revolutions per minute (RPM) is a unit of measurement that indicates how many complete rotations an object makes in one minute. In the video, the skater's spinning speed is given as 100 RPM, which is a key piece of information used to calculate the speed of the skater's hands.
💡Speed
Speed is a measure of how fast an object is moving, typically expressed in terms of distance over time. The video's main objective is to calculate the speed of Brian Boitano's hands as he spins, making speed a fundamental concept in the problem-solving process.
💡Radius (r)
The radius (r) is the distance from the center of a circle to any point on the circumference. In the context of the video, the radius is used to represent the length of the skater's arm, which is crucial for determining the speed of the hands during the spin.
💡Angular Velocity (omega)
Angular velocity (omega) is a measure of the rate of rotation, expressed in radians per second. The video demonstrates how to convert the skater's spinning speed from RPM to radians per second, which is necessary to calculate the linear speed of the skater's hands.
💡Radian
A radian is a unit of angular measure where one radian is the angle subtended at the center of a circle when the length of the arc is equal to the radius of the circle. The video uses radians to express angular velocity, which is essential for the mathematical calculations involved in the problem.
💡SI Units
SI units, or International System of Units, is the modern form of the metric system and is based on the meter, kilogram, and second. The video emphasizes the importance of using SI units, specifically meters and seconds, for the calculations to maintain consistency and accuracy in the physics problem.
💡Linear Speed
Linear speed is the rate at which an object moves along a straight path, as opposed to angular speed which is rotational. The video's problem involves calculating the linear speed of the skater's hands using the formula v = omega * r, where v is the linear speed, omega is the angular velocity, and r is the radius (length of the arm).
💡Miles per Hour (MPH)
Miles per hour (MPH) is a unit of speed expressing the number of miles an object can travel in one hour. The video concludes by converting the calculated speed of the skater's hands from meters per second to miles per hour to provide a more intuitive understanding of the speed involved.
Highlights

The problem involves calculating the speed of an ice skater's hands as he spins

The skater is spinning at 100 revolutions per minute (rpm)

The length of the skater's arm is approximated as 70 centimeters

The relationship between speed (v), angular velocity (omega), and radius (r) is v = omega * r

First, convert 100 rpm to radians per second

Multiply by (1 minute/60 seconds) to get rid of the minutes

Multiply by (2 pi radians/1 revolution) to convert revolutions to radians

So omega = (100 * 2 pi) / (60 seconds) = 100 * 2 pi / 60 radians per second

Then the speed of the hand is v = omega * r = (100 * 2 pi / 60) * 0.7 meters

Simplify to get v ≈ 7.2 meters per second

7.2 meters per second is equivalent to roughly 15 miles per hour

The calculation provides a reasonable estimate of the skater's hand speed during a spin

The problem demonstrates the application of physics concepts to a real-world scenario

It shows the relationship between angular velocity and linear speed

Converting units from rpm to radians per second is a key step in the calculation

The skater's arm length is a crucial parameter in determining the hand's speed

The calculation involves basic arithmetic operations and unit conversions

The final answer provides an intuitive understanding of the skater's motion

The problem engages students by using a relevant and interesting example

It helps students appreciate the relevance of physics in everyday life

Transcripts

Browse More Related Video

Angular Momentum of a Spinning Wheel | Physics with Professor Matt Anderson | M12-16

Linear Speed and Angular Velocity

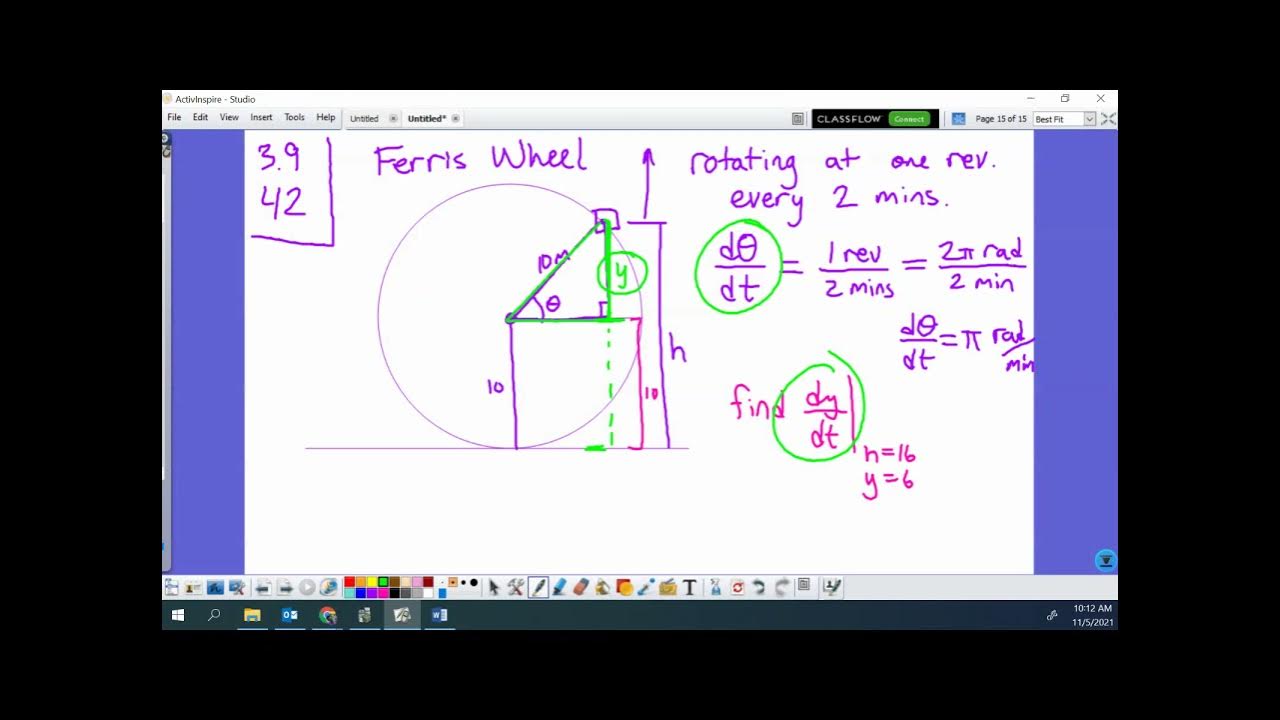
3.9 #42 Related Rates Ferris Wheel Angular Velocity and Right Triangle Trig

Angular Acceleration | Physics

Speed Distance Time | Forces & Motion | Physics | FuseSchool

Rotational Motion - Merry Go Round Slowing Down | Physics with Professor Matt Anderson | M12-03

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
PhysicsIce SkatingSpeed CalculationBrian BoitanoSpinning MotionRPM ConversionMathematicsVelocitySI UnitsHumorous ApproachEducational Content