Spooky Action at a Distance (Bell's Inequality) - Sixty Symbols
TLDRThe video script delves into the intricacies of Bell's inequality, a pivotal concept in quantum mechanics that has profound implications for our understanding of the universe. Introduced by John Bell in 1964 to address the Einstein-Podolsky-Rosen paradox, Bell's inequality challenges the notion of 'spooky action at a distance' and the existence of hidden variables that could explain quantum entanglement without resorting to instantaneous communication between particles. The script explores the thought experiment proposed by David Mermin, which simplifies the complex mathematics behind Bell's theorem, making it more accessible. It discusses the Stern-Gerlach experiment, the concept of entangled states, and the measurement of particle spins. The video also touches on the philosophical and practical implications of quantum mechanics, including the random nature of quantum events and the potential applications in quantum computing and cryptography. It concludes by contemplating the transition from quantum to classical states through decoherence and the potential impact of entangled particles on a cosmic scale.
Takeaways
- 📜 Bell's inequality is a significant concept in quantum mechanics that challenges our understanding of the universe, originally developed by John Bell in 1964 to address the Einstein-Podolski-Rosen paradox.
- 🧲 The Stern-Gerlach experiment from the 1920s demonstrated that particles have discrete properties, such as 'spin up' or 'spin down,' which is a fundamental aspect of quantum mechanics.
- 🤝 Quantum entanglement is a phenomenon where two particles become linked, and the state of one instantly influences the state of the other, regardless of the distance between them.
- 🔄 In an entangled state, measuring one particle's spin in a certain direction forces the other particle into a corresponding state, even when they are far apart.
- 🚫 Einstein's discomfort with quantum mechanics, particularly the idea of 'spooky action at a distance' and his belief in hidden variables that would explain the underlying determinism of quantum phenomena.
- ⚖️ Bell's theorem and the subsequent experiments showed that if local hidden variables existed, they would lead to predictions that contradict the observed results from quantum mechanics.
- 🚀 Quantum mechanics predicts that when entangled particles are measured with different orientations, the outcomes will be different 25% of the time, which is less than the 33% threshold that would be expected if hidden variables were at play.
- ⛓ The concept of decoherence in quantum mechanics explains how entangled states can be disrupted, which is crucial for understanding how quantum systems transition to classical states.
- 🔒 Quantum entanglement is a key principle in modern applications like quantum computing and cryptography, where it can be used to create secure communication channels.
- ⏱️ The instantaneous nature of entanglement does not allow for faster-than-light communication, as the outcomes are random and cannot be used to transmit meaningful information.
- ❗️ Bell's inequality has profound implications for our understanding of reality, suggesting that the universe may be fundamentally probabilistic and not deterministic, despite Einstein's preference for a universe governed by hidden laws.
Q & A
What is Bell's inequality and why is it significant in quantum mechanics?
-Bell's inequality is a theoretical concept in quantum mechanics that was introduced by John Bell in 1964. It is significant because it provides a way to test the local hidden variable theories, which are deterministic alternatives to quantum mechanics. The inequality shows that if local hidden variables were responsible for the properties of quantum particles, certain statistical correlations between distant particles would not be possible. However, experiments have shown that these correlations do occur, suggesting that quantum mechanics is fundamentally non-deterministic and non-local.
Who was John Bell and what is his contribution to quantum mechanics?
-John Bell was a physicist from Northern Ireland who made significant contributions to the foundations of quantum mechanics. His most notable work is the formulation of Bell's inequality, which challenged the concept of local hidden variables proposed by Einstein, Podolsky, and Rosen (EPR). Bell's work has had a profound impact on our understanding of quantum entanglement and non-locality, and it continues to influence discussions about the nature of reality at the quantum level.
What is the EPR Paradox and how does it relate to Bell's inequality?
-The EPR Paradox, named after Einstein, Podolsky, and Rosen, is a thought experiment that questions the completeness of quantum mechanics. It suggests that 'spooky action at a distance' (instantaneous correlation between distant particles) implies that quantum mechanics must be incomplete, as it seems to allow for faster-than-light communication, which violates relativity. Bell's inequality was formulated to test the EPR Paradox's assumptions. The experimental violation of Bell's inequality supports the non-locality of quantum mechanics and suggests that the EPR Paradox's underlying assumptions about local hidden variables are incorrect.
What is the Stern-Gerlach experiment and how does it relate to quantum measurement?
-The Stern-Gerlach experiment, conducted in the 1920s, is a classic experiment that demonstrated the quantization of angular momentum. In this experiment, particles (like silver atoms or electrons) are passed through a non-uniform magnetic field, which causes them to split into discrete paths based on their spin orientation. This experiment is significant because it showed that quantum measurements yield discrete results (e.g., spin up or down) rather than a continuous spectrum, which is a fundamental aspect of quantum mechanics.
What is quantum entanglement and how does it play a role in Bell's inequality?
-Quantum entanglement is a phenomenon where two or more particles become linked in such a way that the state of one particle is immediately connected to the state of the other, no matter the distance between them. This connection is such that the measurement of one particle's property instantaneously affects the state of the other, even when separated by large distances. In the context of Bell's inequality, entanglement is crucial because it allows for the testing of the correlations between particles that the inequality predicts. The experimental confirmation of the violation of Bell's inequality provides strong evidence for the reality of quantum entanglement.
How does David Mermin's work contribute to the understanding of Bell's inequality?
-David Mermin, a renowned physicist, has contributed to the understanding of Bell's inequality by providing more accessible explanations and frameworks for the theorem. He simplified the complex mathematics involved in Bell's work, making it more understandable to a broader audience. Mermin's work has helped to elucidate the principles underlying quantum entanglement and the implications of Bell's inequality for our understanding of quantum mechanics.
What is the concept of 'spooky action at a distance' as referred to by Einstein?
-The term 'spooky action at a distance' was used by Einstein to describe the phenomenon of quantum entanglement, where two particles, regardless of the distance separating them, appear to instantaneously affect each other's states upon measurement. Einstein was uncomfortable with this concept as it seemed to challenge the local realism inherent in classical physics and the principle that nothing can travel faster than the speed of light, as stated in his theory of relativity.
What are hidden variables and why did Einstein believe in their existence?
-Hidden variables are hypothetical, unobserved quantities that, according to some interpretations, could explain the probabilistic nature of quantum mechanics and restore determinism to the theory. Einstein believed in the existence of hidden variables because he was uncomfortable with the idea that quantum mechanics could be inherently probabilistic. He thought that there must be some underlying, yet undiscovered, variables that determine the outcomes of quantum events, thus avoiding the need for 'spooky action at a distance'.
How do the results of experiments on Bell's inequality challenge the concept of hidden variables?
-The results of experiments testing Bell's inequality have consistently shown correlations between entangled particles that are not possible if local hidden variables were the sole determinant of quantum behavior. These findings challenge the concept of hidden variables by demonstrating that the predictions of quantum mechanics, which do not assume the existence of hidden variables, are accurate. This suggests that the universe may indeed operate on fundamentally probabilistic principles rather than deterministic ones.
What is the implication of Bell's inequality for the concept of 'decoherence' in quantum mechanics?
-Bell's inequality and the experiments that have tested it imply that entangled states, which are central to the concept of quantum decoherence, are very delicate and can be easily disrupted. Decoherence is the process by which a quantum system loses its quantum properties as it interacts with its environment, effectively 'collapsing' into a classical state. The sensitivity of entangled particles to their environment, as suggested by the violation of Bell's inequality, underscores the importance of isolating quantum systems to maintain coherence for applications like quantum computing and cryptography.
How does the concept of quantum entanglement relate to modern technologies like quantum computing and cryptography?
-Quantum entanglement is a fundamental principle that underlies many modern technologies. In quantum computing, entanglement allows quantum bits (qubits) to be correlated in ways that enable a quantum computer to perform many calculations simultaneously, offering the potential for significant speedups over classical computers. Similarly, in quantum cryptography, entanglement can be used to create secure communication channels. The security of these channels relies on the fact that any attempt to eavesdrop on the communication would disturb the entangled particles, thus revealing the presence of the eavesdropper.
Outlines
📜 Introduction to Bell's Inequality
The first paragraph introduces Bell's inequality as a significant concept in quantum mechanics, which was developed by John Bell in 1964 to challenge Einstein's views on quantum entanglement. The paper by Bell is acknowledged as complex and challenging, but David Mermin's work helps to make the topic more accessible. The paragraph also discusses the historical context of quantum mechanics experiments, specifically the Stern-Gerlach experiment, which demonstrated the quantized nature of particle spin.
🌀 Quantum Entanglement and Measurement
The second paragraph delves into quantum entanglement, where pairs of particles are created in a state where their spins are correlated. It explains how measurements of the spin of these entangled particles can result in either an 'up' or 'down' state with no in-between, and how changing the orientation of the measurement apparatus affects the outcome. The paragraph also introduces Mermin's thought experiment, which uses a box with a switch and lights to illustrate the principles of entanglement.
🚨 Einstein's Paradox and Hidden Variables
The third paragraph discusses Einstein's discomfort with quantum mechanics, particularly the concept of 'spooky action at a distance' where entangled particles seem to affect each other instantaneously over any distance. It explores the idea of hidden variables that Einstein believed could explain the behavior of quantum particles without resorting to randomness. The paragraph outlines a hypothetical scenario where particles agree on their behavior before being separated, which is tested against the actual results of quantum experiments.
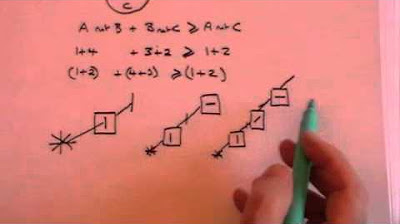
🧩 Bell's Inequality and Quantum Predictions
The fourth paragraph focuses on Bell's inequality, which is used to test the validity of hidden variables theories against the predictions of quantum mechanics. It explains that if particles had predetermined states (hidden variables), the outcome of measurements would be different from what quantum mechanics predicts. The actual experimental results align with quantum mechanics, indicating that the universe operates on probabilities rather than hidden determinism.
🔑 Implications of Quantum Entanglement
The fifth and final paragraph considers the broader implications of quantum entanglement, touching on topics like quantum computing and cryptography. It emphasizes the instantaneous nature of entanglement and its no-go for faster-than-light communication. The paragraph also contemplates the transition from quantum to classical mechanics through decoherence and the potential impact of entangled particles across the universe on each other.
Mindmap
Keywords
💡Bell's inequality
💡Quantum mechanics
💡Entanglement
💡Stern-Gerlach experiment
💡David Mermin
💡Einstein-Podolsky-Rosen paradox
💡Hidden variables
💡Spooky action at a distance
💡Decoherence
💡Quantum computing
💡Geostationary satellite
Highlights
Bell's inequality is a crucial concept in quantum mechanics that has profound implications for our understanding of the universe.
John Bell's 1964 paper challenged Einstein's views and introduced a fundamental principle in quantum mechanics.
David Mermin's work provided a more accessible explanation of Bell's theorem, making it more understandable to a broader audience.
The Stern-Gerlach experiment from the 1920s was pivotal in observing the quantized nature of particle spin.
Quantum entanglement is a phenomenon where particle states are interconnected such that the state of one instantaneously affects the state of another, regardless of distance.
Bell's inequality predicts that if local hidden variables exist, the correlation between entangled particles would be greater than what quantum mechanics predicts, which has been experimentally disproven.
Einstein's concept of 'spooky action at a distance' refers to the instantaneous communication between entangled particles, which he found unsettling.
The idea of hidden variables suggests there is underlying physics that particles carry with them, which is not accounted for in quantum mechanics.
Bell's theorem implies that if particles had predetermined states, the experimental results would contradict observed phenomena, indicating the probabilistic nature of quantum mechanics.
Quantum entanglement is a key principle in the development of quantum computing and modern cryptography.
The concept of decoherence in quantum mechanics addresses how entangled states are maintained or lost when particles interact with their environment.
Entangled particles can be affected by events in distant parts of the universe, but this does not allow for faster-than-light communication or the transmission of usable information.
The randomness and probabilistic nature of quantum mechanics, as demonstrated by Bell's inequality, are fundamental aspects of the universe that cannot be explained away by hidden variables.
The experimental verification of Bell's inequality supports the quantum mechanical prediction that the correlation between entangled particles is less than what would be expected if hidden variables were real.
The implications of Bell's inequality challenge the classical understanding of cause and effect, suggesting a more complex and fundamentally probabilistic universe.
Understanding quantum entanglement has practical implications for technology and communications, as well as philosophical implications for our understanding of reality.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: