Lecture 5 | Topics in String Theory
TLDRThe transcript appears to be a lecture discussing the theoretical aspects of black holes, their properties, and the paradoxes associated with them. It covers the concept of black hole temperature, the ionization of atoms near the event horizon, and the application of the Heisenberg uncertainty principle to scenarios involving black holes. The lecturer uses thought experiments to illustrate the challenges in measuring the properties of particles near a black hole, emphasizing the limitations imposed by quantum mechanics and general relativity. The discussion delves into the increase in temperature as one approaches the horizon, the concept of time dilation, and the idea that an observer falling into a black hole would not experience any dramatic event at the horizon, contrary to an external observer's perspective. The lecture aims to provide a deeper understanding of black holes through a combination of mathematical reasoning and physical intuition, highlighting the current boundaries of scientific knowledge on the subject.
Takeaways
- 📚 The concept of a black hole with a temperature of 3° Kelvin was discussed, which would be in equilibrium with its surroundings due to the cosmic background radiation.
- 🌌 The wavelength of light corresponding to 3° Kelvin is in the microwave range, specifically around 1 cm to 10 m, which is associated with the cosmic microwave background.
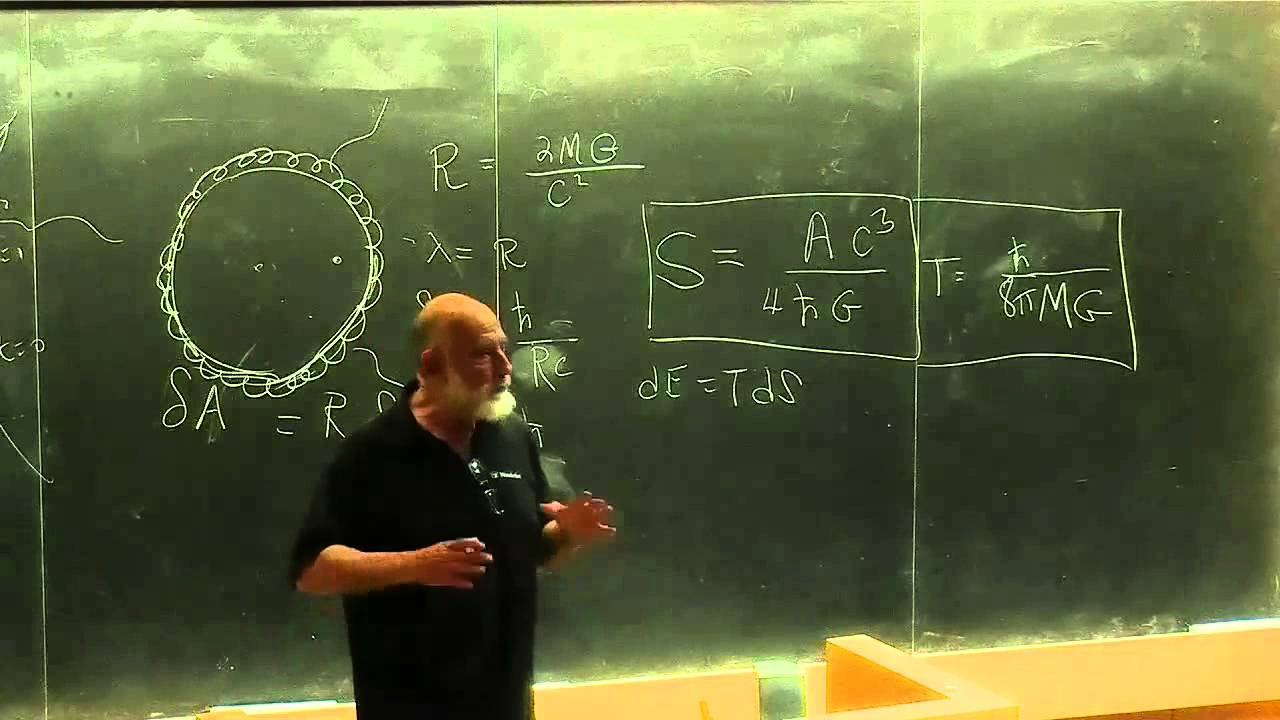
- 🔍 The radius of a black hole that emits light with a wavelength of 1 cm was estimated to be around 1 cm, which is the Schwarzschild radius.
- ⚖️ The mass of a black hole with a 1 cm radius (and thus a temperature of 3° Kelvin) was approximated to be roughly the mass of the Earth.
- 📈 The Penrose diagrams for flat space and a Schwarzschild black hole were used to visualize the spacetime structure without and with a black hole.
- 🤔 The idea of creating a black hole by collapsing a shell of light (or radiation) was explored, which would theoretically lead to the formation of a black hole if the shell's energy were sufficiently concentrated.
- ⚫️ Birkhoff's theorem was introduced, stating that outside a spherically symmetric mass distribution, the spacetime geometry is the same as if all the mass were concentrated at the center.
- ♾️ The black hole formation process was described, where an incoming shell of light creates a region of spacetime that is described by the Schwarzschild metric outside and flat spacetime inside, up until the shell's arrival at the center.
- 🔥 The temperature of a black hole, known as the Hawking temperature, increases as one approaches the event horizon, leading to a very hot environment near the horizon.
- ⏳ Time dilation effects mean that an object falling into a black hole will experience its own time normally, but to an outside observer, the object will appear to slow down and take an infinite amount of time to reach the event horizon.
- ❓ The paradox of an object falling into a black hole experiencing extreme temperatures while an outside observer sees it frozen at the event horizon is a complex issue that intertwines gravity, quantum mechanics, and the nature of information.
Q & A
What is the significance of a black hole having a temperature of 3° Kelvin?
-A black hole at 3° Kelvin is significant because it would be in equilibrium with its surroundings due to the cosmic background radiation. However, this equilibrium is unstable; any fluctuation in temperature would cause the black hole to either heat up or cool down rapidly.
What is the relationship between the wavelength of light emitted by a black hole and its size?
-The wavelength of light emitted by a black hole is approximately the same size as the black hole's radius. This relationship allows us to estimate the radius of a black hole based on the temperature or wavelength of the light it emits.
How does the mass of a black hole relate to its radius?
-The mass and radius of a black hole are proportional to each other. If a black hole has a radius of 1 cm, its mass would be roughly the mass of the Earth, according to the script.
What is the Penrose diagram, and how is it used in the context of black holes?
-A Penrose diagram is a graphical representation of the causal structure of spacetime using a conformal transformation to compactify infinity. It is used to visualize the geometry of spacetime, including the behavior near black holes, by 'smushing' or compactifying space into a finite region.
What is Birkoff's theorem, and how does it apply to the formation of a black hole?
-Birkoff's theorem states that the spacetime geometry inside a spherically symmetric mass distribution is flat, and outside the shell, the geometry is the same as if all the mass were concentrated at the center. This theorem is used to describe the formation of a black hole by imagining a shell of light (or mass) collapsing inwards, creating a region of flat spacetime inside the shell and a black hole geometry outside.
What is the definition of the event horizon of a black hole?
-The event horizon is the boundary in spacetime beyond which events cannot affect an outside observer. It is the surface that separates the region from which light can escape from the region where it cannot, effectively marking the 'point of no return' for anything falling into the black hole.
How does the concept of thermal equilibrium relate to the temperature of a black hole?
-Thermal equilibrium refers to a state where the temperature is constant throughout a system and entropy is maximized. For a black hole, the concept is complex due to the presence of gravity. The temperature of a black hole, as perceived by a distant observer, is given by the Hawking temperature, which is cold. However, as one approaches the black hole, the effective temperature increases, becoming extremely high near the event horizon.
What is the Hawking temperature, and how does it relate to the size of a black hole?
-The Hawking temperature is the temperature of a black hole as perceived by a distant observer. It is inversely proportional to the size of the black hole's event horizon. Larger black holes have a lower Hawking temperature and are 'colder,' while smaller black holes have a higher Hawking temperature and are 'hotter.'
What is the implication of the Heisenberg uncertainty principle in the context of measuring the properties of particles near a black hole?
-The Heisenberg uncertainty principle implies that it is impossible to simultaneously measure the position and momentum of a particle with arbitrary precision. This becomes particularly relevant near a black hole, where the intense gravitational field and high temperatures make it impossible to measure a particle's properties without altering them, such as when trying to determine if a particle is ionized by the high temperature near the event horizon.
How does the concept of a black hole's entropy being proportional to its event horizon area relate to the information paradox?
-The concept that a black hole's entropy is proportional to its event horizon area suggests that information about what falls into a black hole may be somehow 'stored' on the horizon. This ties into the information paradox, which questions what happens to the information about particles that fall into a black hole, as traditional theories suggest that this information is lost, contradicting the principles of quantum mechanics.
What is the role of quantum mechanics in understanding the behavior of particles near the event horizon of a black hole?
-Quantum mechanics plays a crucial role in understanding the behavior of particles near the event horizon of a black hole. It introduces phenomena such as quantum fluctuations and the Hawking radiation process, which predicts that black holes emit particles due to quantum effects near the event horizon. Quantum mechanics also raises questions about the nature of information and its conservation in the context of black holes.
Outlines
😀 Estimating the Size of a 3 Kelvin Black Hole
The paragraph discusses the theoretical exercise of calculating the size of a black hole with a temperature of 3 Kelvin, which is the same as the cosmic background radiation. It highlights the relationship between the wavelength of light emitted by a black hole and its size, concluding that a black hole with a 1 cm wavelength would have a Schwarzschild radius of about a centimeter. The mass of such a black hole is estimated to be roughly the mass of the Earth.
📐 Penrose Diagrams and Black Hole Geometry
This section delves into the Penrose diagrams for flat space and a Schwarzschild black hole. It emphasizes the importance of visual representation to understand complex mathematical concepts without delving too deep into calculations. The description of the Penrose diagram for flat space is provided, including the behavior of light rays and the concept of time and radial distance in the diagram.
🌌 Creating a Black Hole and Its Penrose Diagram
The paragraph explores the process of creating a black hole from a mathematical perspective, using Penrose diagrams. It describes the structure of space-time for a black hole and how it transitions from flat space far away from the black hole to the Schwarzschild metric near the event horizon. The concept of a singularity is introduced, and the behavior of light rays in relation to the black hole is discussed.
🌟 Birkoff's Theorem and Its Implications for Black Holes
This part of the script introduces Birkoff's theorem, which is a generalization of Newton's theorem for spherically symmetric mass distributions. It explains that the gravitational field inside a spherical shell of mass is zero, and outside it, the field is as if all the mass were concentrated at the center. The theorem is applied to the idea of creating a black hole by compressing the Earth's mass into a sphere, resulting in a black hole with a radius of about a centimeter.
💥 The Formation and Properties of a Black Hole Horizon
The paragraph discusses the concept of a black hole's horizon and its formation. It explains that the horizon is the boundary separating the regions from which escape to infinity is possible and impossible. The text uses the analogy of a drain hole in a lake to describe the point of no return. The behavior of light rays and the concept of the horizon growing in size as more mass falls into the black hole are also covered.
🌡️ Black Hole Temperature and Quantum Phenomena
This section addresses the temperature of black holes, introducing the concept of Hawking temperature. It explains that black holes have a temperature and entropy proportional to the horizon area. The text also touches on the quantum phenomena involved in the increase of a black hole's area with the addition of information, and the theoretical aspects of a black hole's evaporation due to quantum effects.
🔬 The Heisenberg Uncertainty Principle and Black Hole Experiments
The final paragraph explores the application of the Heisenberg uncertainty principle to the problem of measuring the properties of particles near a black hole's horizon. It discusses the challenges of conducting experiments to determine the state of particles falling into a black hole, highlighting the inherent contradictions and limitations imposed by quantum mechanics. The text concludes with the idea that certain questions about black holes may be unanswerable through experimentation due to these quantum uncertainties.
Mindmap
Keywords
💡Black Hole
💡Hawking Temperature
💡Penrose Diagram
💡Schwarzschild Radius
💡Cosmic Microwave Background Radiation
💡Birkhoff's Theorem
💡Event Horizon
💡Singularity
💡Entropy
💡Heisenberg Uncertainty Principle
💡Quantum Mechanics
Highlights
The concept of building a black hole as an academic exercise was introduced, emphasizing the theoretical and mathematical approach rather than physical construction.
The importance of the cosmic microwave background radiation temperature of 3° Kelvin in relation to black hole equilibrium was discussed.
The wavelength of light corresponding to a 3° black hole was identified as being in the microwave range, specifically around a centimeter.
The relationship between the wavelength of light emitted by a black hole and its size was explored, with the radius of the black hole being roughly equivalent to the wavelength of light.
The calculation of the Schwarzschild radius for a black hole with the mass of the Earth was performed, resulting in an approximate size of a centimeter.
The Penrose diagrams for flat space and a Schwarzschild black hole were used to visualize the space-time geometry surrounding a black hole.
Birkhoff's theorem was introduced, explaining the gravitational field outside a spherically symmetric mass distribution is the same as if all the mass were concentrated at the center.
The formation of a black hole from an incoming light shell was described, illustrating the concept of the event horizon and its growth over time.
The theoretical experiment of lowering an object towards a black hole to measure the temperature increase near the horizon was proposed.
The paradox of a black hole's temperature as perceived by a distant observer versus an observer falling into the black hole was discussed.
The limitations of measuring temperature near a black hole due to the need for high-energy photons to obtain information were highlighted.
The Heisenberg uncertainty principle was related to the challenges of conducting experiments near a black hole, where the act of measurement affects the outcome.
The idea that the environment near a black hole's horizon gets hotter as objects fall in was explored, with implications for the object's interaction with this environment.
The concept of a black hole's temperature being related to the kinetic energy of particles was discussed, with a focus on the practical challenges of measuring this temperature.
The philosophical and scientific implications of the information paradox and the concept of an event horizon were touched upon.
The conclusion that it is impossible to experimentally confirm the lack of high temperature experienced by an atom falling into a black hole was reached.
Transcripts
Browse More Related Video

Lecture 4 | Topics in String Theory

Deriving Hawking's most famous equation: What is the temperature of a black hole?

Lecture 2 | Topics in String Theory

How Does Gravity Escape A Black Hole?

Black Hole Bonanza: StarTalk Live! With Neil deGrasse Tyson, Janna Levin, & Jenny Greene
Inside Black Holes | Leonard Susskind
5.0 / 5 (0 votes)
Thanks for rating: