Lecture 4 | New Revolutions in Particle Physics: Standard Model
TLDRThe video script is an in-depth exploration of quantum chromodynamics (QCD), a fundamental theory in particle physics that describes the interactions between quarks and gluons, collectively known as partons. The lecturer delves into the concept of color charge, analogous to electric charge but within the context of the strong nuclear force. They explain how the theory ensures that only color-neutral (singlet) states, such as baryons (three quarks) and mesons (quark-antiquark pairs), can exist as free particles, while individual quarks and gluons are confined within these composite particles. The script also touches on the mathematical framework of QCD, including group theory and the representations of the SU(3) group, which underpins the theory's structure. The lecturer discusses the properties of gluons, which are unique in that they carry color charge and can interact with other gluons, leading to complex dynamics and the formation of 'flux tubes' that confine quarks. The summary concludes with the implications of these interactions for the observable particles in nature and hints at the broader structure of the standard model of particle physics, which includes additional symmetries and the Higgs field.
Takeaways
- 📚 The speaker discusses the concept of group theory and its significance in theoretical physics, particularly in the context of quantum mechanics and particle physics.
- 🧲 Group theory is used to describe the symmetries of physical systems, and rotations in space are a key example, where the rotation group is represented by matrices that act on physical quantities.
- 🌀 In quantum mechanics, states are represented by vectors in a linear space, and the size of these vectors depends on the number of mutually orthogonal states of the system.
- 🎭 The speaker uses the example of Alice and Bob to illustrate the abstract nature of group theory, with Alice learning about the rotation of a spin in quantum mechanics.
- 🔍 The concept of unitary matrices is introduced, which are matrices that preserve the inner product in a vector space and are crucial in quantum mechanics for representing state changes.
- 🤔 The determinant of a unitary matrix is a complex number with a magnitude of one, which is a key property used to define special unitary groups.
- 🤓 The speaker explores the idea of representations of groups, explaining that the same abstract group element can have different matrix representations of varying dimensions.
- 👥 The importance of generators of a group is highlighted, noting that they are related to infinitesimal elements of the group and are conserved quantities that play a role in conservation laws.
- 🤖 The script touches on the application of group theory to quantum chromodynamics (QCD), discussing the behavior of quarks and gluons under the SU(3) symmetry group.
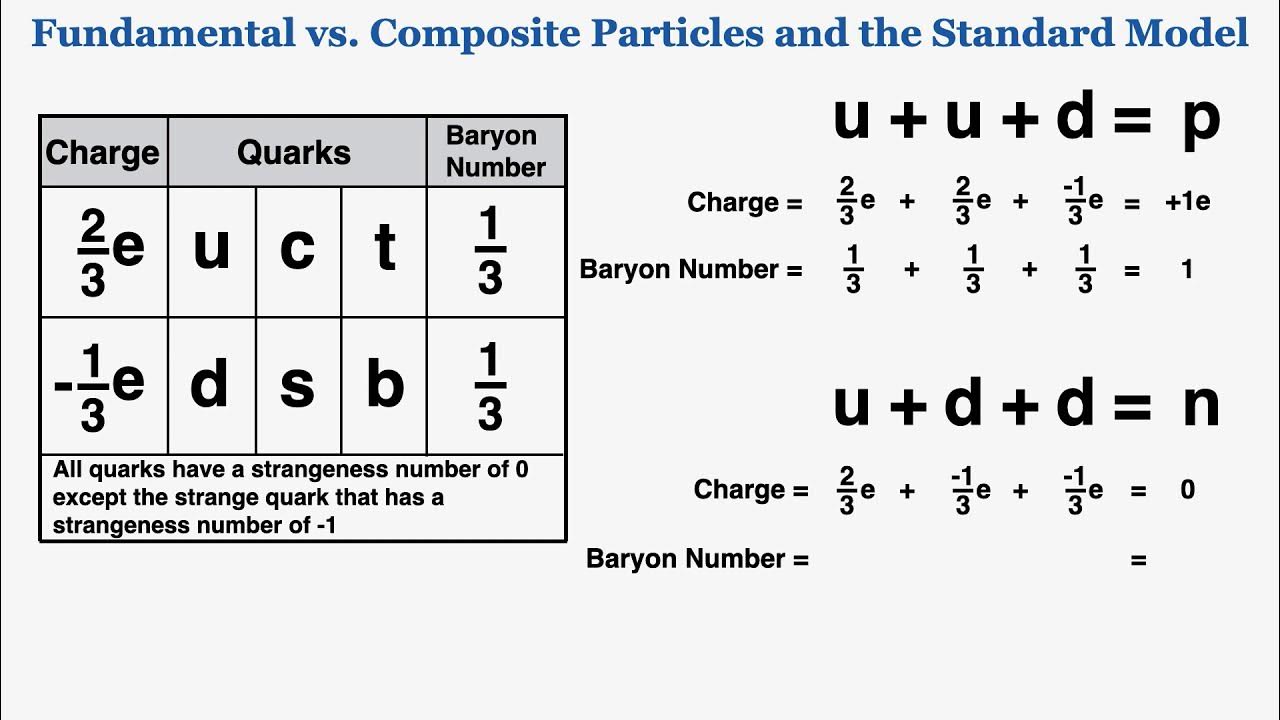
- 🧬 The combination of quarks to form baryons and mesons is explained, noting that these composite particles must be color singlets to exist as free particles in nature.
- 🔬 The speaker also discusses the concept of gluons, which are particles that carry the color charge in QCD, and how they interact with each other to form flux tubes that contribute to the confinement of quarks.
- 🧲 The dynamics of quantum chromodynamics are more complex than electrodynamics due to the self-interaction of gluons, which are unlike photons that do not interact with each other.
Q & A
What is the main topic of the lecture?
-The main topic of the lecture is the exploration of group theory, specifically SU(2) and SU(3), in the context of quantum mechanics and quantum chromodynamics (QCD), including the representation of particles such as quarks and gluons under these groups.
What is a representation in group theory?
-A representation of a group is a way of expressing the abstract elements of the group as linear transformations of a vector space. It is a one-to-one correspondence between the group elements and matrices, where the group operations correspond to matrix multiplication.
What is the significance of the determinant condition in unitary matrices?
-The determinant condition in unitary matrices ensures that the determinant of the matrix is equal to one. This condition is important because it maintains the conservation of probability in quantum mechanics and is consistent with the law of matrix multiplication.
How does the speaker describe the role of generators in group theory?
-The speaker describes generators as the infinitesimal elements of the group that are closely related to the small operations within the group. Generators are important because they can be used to build up any element of the group through a sequence of small operations and are associated with conserved quantities in physics.
What is the relationship between the electron spin and the SU(2) group?
-The electron spin is represented by the SU(2) group. The group elements, which are abstract rotations, are represented by 2x2 unitary matrices that act on the state vectors of the electron's spin. This representation is called a doublet, reflecting the two mutually orthogonal states of an electron's spin (spin up and spin down).
What does the speaker mean by 'color' in the context of quantum chromodynamics (QCD)?
-In the context of QCD, 'color' refers to a quantum number that quarks carry. It is analogous to electric charge in that it is a source of the gluon field. The 'color' property ensures that quarks and gluons interact in a way that prevents them from existing as isolated particles, leading to the phenomenon of confinement.
How does the speaker explain the concept of confinement in QCD?
-The speaker explains confinement by describing the gluon field lines that connect quarks. These lines of flux attract each other and form tubes, or flux tubes, with a uniform charge per unit distance. The energy of these flux tubes grows linearly with the distance between quarks, making it energetically unfavorable for quarks to separate, which results in confinement.
What is the significance of the singlet state in the context of particle interactions?
-The singlet state is significant because it represents a state of particles that does not transform under the action of the group, meaning it is invariant or 'neutral'. In QCD, particles that are observed as free are always in a color singlet state, which is analogous to being electrically neutral and does not interact strongly with other particles.
What is the role of the Higgs field in the standard model?
-The Higgs field, while not discussed in detail in the script, is known to play a crucial role in the standard model by providing a mechanism for electroweak symmetry breaking and the acquisition of mass by elementary particles such as quarks and leptons.
What are the three fundamental representations of SU(3) that the speaker discusses?
-The three fundamental representations of SU(3) discussed by the speaker are the triplet, the anti-triplet (or complex conjugate representation), and the singlet. These representations are significant for understanding the behavior of quarks and antiquarks under the color symmetry of QCD.
How does the speaker describe the process of combining two quarks or a quark and an antiquark to form a particle?
-The speaker describes that when two quarks are combined, they can form a particle that behaves like an anti-quark under the SU(3) symmetry, which is a bit odd but true. When a quark and an antiquark are combined, they can form a particle that is a color singlet, meaning it does not interact strongly with other particles and is analogous to a spin zero state in electrodynamics.
Outlines
📚 Introduction to Quantum Mechanics and Group Theory
The speaker begins by expressing enthusiasm for a topic they've wanted to address for years. They humorously suggest a commercial break for viewers to purchase their book, 'The Black Hole War.' The narrative then shifts to a discussion about theoretical physicists and introduces the concept of group theory, specifically rotation in space. The speaker explains the representation of quantum states using linear vector spaces and column vectors, varying in size depending on the system's complexity. The explanation includes the role of group theory in quantum mechanics, the concept of rotation group representations, and the implications for electron spin.
🧲 The Abstract Concept of Rotations and Matrices
The paragraph delves into the specifics of rotation groups and their matrix representations. It discusses how the size of matrices correlates with the number of orthogonal states in a system. The speaker uses the example of an electron's spin to illustrate the use of two by two matrices. The explanation extends to higher dimensions, such as three-dimensional matrices for spin one particles, and touches on the trivial case of spin zero particles. The importance of matrix dimensionality in relation to the abstract group element is emphasized, along with the concept of unitary matrices and their determinants.
🔄 The Connection Between Unitary Matrices and Rotations
The speaker explores the relationship between unitary matrices and rotations in three-dimensional space. They highlight the special unitary matrices, which are linked to the group of rotations, and discuss the determinant of these matrices. The explanation clarifies that while the determinant of a unitary matrix must be a pure phase, it is not necessarily real. The paragraph also corrects a previous statement, noting that there is a two-to-one correspondence between unitary operators and rotations, not a one-to-one correspondence, and introduces the concept of a doublet in the context of electron spin.
🚀 Generators of a Group and Their Role in Symmetry
The paragraph introduces the concept of group generators, which are related to infinitesimal elements of the group. The speaker explains that generators are crucial for constructing the entire group structure as they represent small transformations that can be compounded to form larger ones. The emphasis is on the generators' role in conserved quantities and their connection to conservation laws. The discussion also involves the properties of generators, such as being hermitian and having trace equal to zero, which are linked to observable quantities in quantum mechanics.
🎭 Representations and the Combining of Particle Spins
The speaker discusses the representation of groups and how combining systems can lead to new representations. Using the example of two half-spin particles, the paragraph explains the creation of new states that can be either spin zero or spin one. The discussion extends to the SU(3) group, which is relevant to particle physics, and the importance of understanding how spins combine. The speaker also touches on the concept of color in quantum chromodynamics and the role of the SU(3) group in representing quark colors.
🌈 The Color Symmetry in Quantum Chromodynamics
The paragraph focuses on the application of group theory to quantum chromodynamics (QCD), specifically the SU(3) group that represents color symmetry. The speaker describes the transformation properties of quarks and antiquarks under the SU(3) group and introduces the concept of the complex conjugate representation. The discussion also covers the creation of particles from quarks and antiquarks, known as mesons, and the properties of gluons, which are the force carriers in QCD. The speaker emphasizes that all observable particles are color singlets, meaning they do not transform under the SU(3) group.
🔬 The Spectrum of Strongly Interacting Particles
The speaker elaborates on the spectrum of strongly interacting particles, which includes baryons, mesons, and glueballs. They explain the process of creating singlets from quarks and antiquarks, and the importance of color singlets in particle physics. The paragraph also discusses the electric charges that result from combining quarks and the prohibition of fractional charges in nature. The speaker connects the concept of color singlets to the entanglement of particles and the challenges of manipulating such states.
🧬 The Tensor Nature of Particle Combinations
The paragraph delves into the tensor-like nature of objects with two indices, such as field operators that create particles. The speaker discusses the action of the SU(2) group on these composite objects and how they mix under group operations. The explanation highlights the discovery of certain combinations that remain invariant, or singlets, under these operations. The discussion also touches on the concept of entanglement in the context of particle physics and the challenges associated with such states.
🔍 The Multiplication of SU(3) Representations
The speaker explores the multiplication of SU(3) representations and the resulting combinations of quarks that form singlets or other representations. They discuss the existence of matrix representations for various dimensionalities and the importance of singlets in the context of neutral objects. The paragraph also covers the epsilon symbol and its role in creating color singlets from quark fields. The speaker provides a detailed look at the multiplication of two gluons and the resulting singlet state.
📐 The Structure of SU(3) Representations
The paragraph focuses on the structure of SU(3) representations, specifically the singlet and octet representations formed by the combination of two gluons. The speaker explains the existence of distinct ways to create octets and the concept of a 'Ken' in the context of gluon combinations. The discussion also touches on the 27-dimensional representation and the implications for the structure of the SU(3) group. The speaker emphasizes the block diagonal nature of matrices in certain bases and the simplification that occurs when considering specific combinations of states.
🚨 The Non-Existence of Free Quarks
The speaker discusses the concept that free quarks do not exist in nature, which is a fundamental aspect of quantum chromodynamics. They explain that the gluon field, unlike the photon in electrodynamics, is not neutral and thus quarks cannot exist independently. The paragraph also covers the historical development of the idea of color and gluons, and the realization that color singlets prevent the occurrence of fractionally charged particles. The speaker connects the concept of confinement to the strong coupling constant in QCD and the resulting energetic implications.
⚙️ The Dynamics of Quantum Chromodynamics
The paragraph delves into the dynamics of quantum chromodynamics (QCD), highlighting the differences between QCD and electrodynamics. The speaker explains that gluons, unlike photons, carry color charge and interact with each other, leading to a more complex theory. The discussion focuses on the concept of flux tubes, which form due to the attractive nature of gluon fields, and how these tubes contribute to the confinement of quarks. The speaker also describes the process of particle creation in QCD, where quark-antiquark pairs can be generated, leading to the formation of jets.
🧲 The Formation of Quark Bundles in QCD
The speaker discusses the phenomenon of three quarks coming together to form a neutral object in the context of QCD. They explain that the lines of flux from each quark can cancel each other out, resulting in a color-neutral state. The paragraph also touches on the concept of gauge theories, which are based on symmetries and conserved quantities, and how these theories are connected to photon-like fields. The speaker emphasizes the importance of the Higgs field in the standard model of particle physics and hints at the remaining components to be covered in future lectures.
🔬 Wrapping Up the Standard Model
The speaker summarizes the progress made in understanding the standard model of particle physics, specifically mentioning the study of the SU(3) and U(1) groups, and the introduction of the electric charge. They outline the plan to cover the remaining components, including the SU(2) group and the Higgs field, within the next few lectures. The speaker expresses optimism about completing the basic structure of the standard model and then exploring the puzzles and potential extensions beyond it.
Mindmap
Keywords
💡Quantum Chromodynamics (QCD)
💡Gluon
💡Quark
💡Color Charge
💡Flux Tube
💡SU(3)
💡Confinement
💡Standard Model
💡Higgs Field
💡Gauge Theory
💡SU(2)
Highlights
The speaker discusses the concept of group theory and its importance in theoretical physics, particularly in the context of quantum mechanics.
An explanation of the representation of rotations in space and how they act on physically relevant quantities is provided.
The talk explores the idea that the states of a system in quantum mechanics are represented by linear vector spaces.
The size of the column vectors representing a system's state depends on the number of mutually orthogonal possibilities for that system.
The concept of infinite-dimensional matrices representing the rotation group is introduced.
The speaker elaborates on the symmetry operations for a spin 1/2 particle, specifically the electron, and their representation by matrices.
Unitary matrices are defined, and their importance in the context of group theory and quantum mechanics is discussed.
The determinant of a unitary matrix is shown to be a pure phase, which is crucial for understanding the properties of these matrices.
The special unitary group SU(2) is identified as being isomorphic to the group of rotations in three-dimensional space.
The concept of generators of a group is introduced, emphasizing their role as the conserved quantities associated with the symmetry group.
The speaker explains the commutation relations of the generators, which are fundamental to the structure of the group.
The representation of quarks and antiquarks under the SU(3) group is discussed, highlighting the difference between them and their transformation properties.
The multiplication of representations in SU(3) is explored, leading to the discovery of various particle states such as baryons, mesons, and glueballs.
The principle that all observable particles are color singlets in quantum chromodynamics is explained.
The dynamics of quantum chromodynamics (QCD) are discussed, including the behavior of gluons and the formation of flux tubes.
The concept of confinement in QCD is introduced, explaining why quarks are never observed in isolation.
The Higgs field's role in the standard model of particle physics is alluded to, suggesting its importance in future discussions.
Transcripts
Browse More Related Video

Lecture 2 | New Revolutions in Particle Physics: Standard Model

Lecture 6 | New Revolutions in Particle Physics: Standard Model

What Makes The Strong Force Strong?

Revealing the Mysterious World Inside Protons
Fundamental vs. Composite Particles and the Standard Model - IB Physics

Lecture 5 | New Revolutions in Particle Physics: Standard Model
5.0 / 5 (0 votes)
Thanks for rating: