Kinetic Energy EXPLAINED in 5 Levels - Beginner to Advanced (Classical Physics by Parth G)
TLDRThis video delves into the concept of kinetic energy, exploring its definition, calculation, and implications across varying difficulty levels. It starts with the basics, explaining kinetic energy as the energy of motion, dependent on an object's speed and mass, and introduces the formula (1/2)mv^2. The discussion progresses to reference frames, highlighting how kinetic energy can differ depending on the observer's perspective. It then transitions into special relativity, connecting the classical kinetic energy equation to the relativistic framework and explaining the role of momentum and mass. Finally, the video touches on rotational kinetic energy and quantum mechanics, offering a comprehensive understanding of kinetic energy in various physical contexts.
Takeaways
- 🌟 Kinetic energy is the energy an object possesses due to its motion, directly depending on its speed.
- 📐 The formula for calculating kinetic energy is (1/2)mv^2, where m is the mass and v is the speed of the object.
- 🔄 Doubling the speed of an object results in a fourfold increase in its kinetic energy due to the squared factor in the equation.
- 🏎️ Objects with greater mass traveling at the same speed have more kinetic energy, which influences the potential impact in a collision.
- 📏 Speed in the context of kinetic energy is a scalar quantity, meaning only the magnitude matters, not the direction.
- 🪐 Kinetic energy can vary in value depending on the reference frame, but the change in energy is consistent across frames.
- 🚀 In special relativity, the relativistic kinetic energy equation incorporates momentum and the speed of light, leading to E=mc^2 for stationary objects.
- 🔧 The classical kinetic energy formula (1/2)mv^2 is derived from the relativistic equation under the binomial approximation for non-relativistic speeds.
- 🔄 Beyond linear kinetic energy, there's also rotational kinetic energy, which depends on the object's moment of inertia and angular speed.
- ⚛️ Quantum mechanics views kinetic energy through the lens of operators and measurements, with the system collapsing into a specific state upon measurement.
Q & A
What is the basic concept of kinetic energy?
-Kinetic energy is the energy that an object possesses due to its motion. The faster an object is moving, the more kinetic energy it has. It is directly related to the object's speed and its mass, and is measured in Joules.
How is kinetic energy calculated?
-The kinetic energy of an object with mass 'm' moving at speed 'v' is calculated using the formula KE = (1/2)mv^2. This equation shows that the kinetic energy is directly proportional to the square of the object's speed and also depends on its mass.
What happens to an object's kinetic energy if its speed doubles?
-If an object's speed is doubled, its kinetic energy increases by a factor of four, because kinetic energy is proportional to the square of the speed. This means the kinetic energy increases significantly with even small changes in speed.
Why does the mass of an object affect its kinetic energy?
-The mass of an object affects its kinetic energy because it is a factor in the kinetic energy equation. Heavier objects moving at the same speed as lighter objects have more kinetic energy, which is why a larger vehicle like a truck is more dangerous in a collision than a smaller car.
What is the significance of the 1/2 factor in the kinetic energy equation?
-The 1/2 factor in the kinetic energy equation is a result of the way energy is transferred in classical mechanics. It arises naturally when deriving the equation from more fundamental principles, and its presence ensures that the equation correctly predicts the energy transfer in collisions.
How does the concept of reference frames affect the understanding of kinetic energy?
-Different reference frames can perceive an object's kinetic energy differently. While one observer might see an object with no kinetic energy because it appears stationary, another observer moving relative to the first observer might see the object with significant kinetic energy. The key point is that while the absolute values of kinetic energy can differ, the change in kinetic energy (such as during a collision) is consistent across all reference frames.
What is special relativity's perspective on kinetic energy?
-Special relativity, developed by Albert Einstein, modifies the classical view of kinetic energy at high speeds close to the speed of light. It introduces the concept that the energy of an object is equal to its mass times the speed of light squared (E=mc^2), and for moving objects, the relativistic kinetic energy is derived from the momentum-energy equation, which includes the object's momentum and rest mass.
How does quantum mechanics treat kinetic energy?
-In quantum mechanics, the kinetic energy of an object is not a fixed property but rather a potential outcome when a measurement is made. The kinetic energy is associated with an operator that, when applied to the object, causes the system to 'collapse' into a state with a specific momentum and kinetic energy, according to the Copenhagen interpretation of quantum mechanics.
What is the difference between linear and rotational kinetic energy?
-Linear kinetic energy is the energy an object has due to its motion in a straight line and depends on its mass and linear speed. Rotational kinetic energy, on the other hand, is the energy due to an object's rotation or angular motion and depends on the object's moment of inertia and its angular speed.
Why is it important to understand the different forms of kinetic energy?
-Understanding the different forms of kinetic energy is crucial because it allows us to accurately predict and calculate the behavior and interactions of objects in various scenarios, such as collisions, rotations, and in different physical contexts like relativistic speeds and quantum states.
How does the concept of kinetic energy apply to everyday situations?
-Kinetic energy principles are applied in various everyday situations, such as vehicle safety (e.g., seatbelts and crumple zones), sports (e.g., the force of a ball hit by a bat), and energy conservation in mechanical systems (e.g., generators and turbines). It helps us understand the transfer of energy and the potential for work to be done.
Outlines
🌟 Introduction to Kinetic Energy
The video begins with an introduction to the concept of kinetic energy, explaining it as a fundamental aspect of physics. The host, Parth, outlines the video's structure, which covers kinetic energy across five different levels of difficulty, allowing viewers to engage with the content according to their familiarity with the topic. He emphasizes the importance of energy in understanding how objects behave and interact, and specifically defines kinetic energy as the energy an object possesses due to its motion. The video encourages viewer interaction through likes, subscriptions, and topic suggestions, setting a collaborative tone for the educational content to follow.
📚 Understanding Kinetic Energy
This paragraph delves deeper into the specifics of kinetic energy, starting with a basic question about what it is. The explanation progresses from a general definition to a mathematical formula, (1/2)mv^2, which quantifies the kinetic energy of an object. The video uses the example of a wooden block to illustrate how changes in speed and mass affect an object's kinetic energy. It highlights the significance of speed squared in the equation and discusses the implications of mass on kinetic energy, using the analogy of a truck and a car to demonstrate the concept. The paragraph also touches on the scalar nature of speed versus the vector nature of velocity and hints at the importance of the 1/2 factor in the equation, promising further explanation in a later level.
🌍 Reference Frames and Kinetic Energy
The third level of the video introduces the concept of reference frames and their impact on the perception of an object's kinetic energy. It explains that the measured speed of an object is always relative to something else, using the example of observing an object's motion from Earth versus Mars. The video emphasizes that while different reference frames may assign different kinetic energies to an object, what's crucial is the change in energy. It draws an analogy with gravitational potential energy and the concept of setting a 'zero point' to clarify this idea. The level concludes with a brief mention of the transition from Newtonian physics to Einstein's theory of relativity, setting the stage for the next level's discussion on special relativity and its implications for kinetic energy.
🔌 Special Relativity and Kinetic Energy
This level explores the treatment of kinetic energy within the framework of special relativity, a concept developed by Albert Einstein. It challenges the classical addition of velocities, using the example of a car and a thrown ball to illustrate how velocities are not simply additive. The video explains that relativity requires a different perception of space and time, leading to a modified understanding of kinetic energy. It introduces the famous equation E=mc^2 and expands on it to include moving objects, using the concept of momentum and the speed of light. The video then connects this relativistic kinetic energy to the classical equation, explaining the origin of the 1/2 factor and how the classical equation is近似 to the full relativistic treatment. The level provides a deeper understanding of how kinetic energy is viewed in the context of modern physics.
🤔 Advanced Concepts in Kinetic Energy
In the final level, the video introduces additional aspects of kinetic energy beyond the linear motion discussed earlier. It differentiates between linear and rotational kinetic energy, explaining that the latter depends on an object's moment of inertia and angular speed. The video also touches on the concept of kinetic energy in quantum mechanics, highlighting the role of operators and measurements in determining an object's momentum and kinetic energy. It contrasts this with the classical and relativistic views, where the object is assumed to have a definite kinetic energy. The level concludes with a call to action for viewers to engage with the content, subscribe for more, and support the creator through Patreon and merchandise.
Mindmap
Keywords
💡Kinetic Energy
💡Energy
💡Speed
💡Mass
💡Reference Frames
💡Special Relativity
💡Momentum
💡Einstein's E=mc^2
💡Rotational Kinetic Energy
💡Quantum Mechanics
Highlights
Kinetic energy is a fundamental concept in physics that describes the energy an object possesses due to its motion.
Energy is a quantifying measure that provides insight into an object's behavior and allows for predictions of future actions.
Kinetic energy is directly proportional to the speed of an object, with faster moving objects possessing more kinetic energy.
An object at rest has no kinetic energy, whereas even a small change in speed results in kinetic energy.
The kinetic energy of an object is calculated using the equation KE = (1/2)mv^2, where m is the mass and v is the velocity of the object.
The factor of speed squared in the kinetic energy equation indicates that a doubling of speed results in a quadrupling of kinetic energy.
Mass plays a significant role in kinetic energy, with heavier objects having more kinetic energy at the same speed than lighter ones.
Kinetic energy is measured in Joules, the standard SI unit for energy, just like other forms of energy.
Reference frames play a crucial role in understanding kinetic energy, as the measured speed (and thus kinetic energy) of an object can vary depending on the observer's frame of reference.
The relativity of kinetic energy means that different observers may assign different kinetic energies to the same object, but agree on the change in energy.
Special relativity, developed by Albert Einstein, introduces a nuanced view of kinetic energy that differs from classical physics, especially at speeds close to the speed of light.
The famous equation E = mc^2 from relativity is part of a larger equation that describes the kinetic energy of moving objects in the relativistic framework.
The classical kinetic energy equation (1/2)mv^2 is derived from the relativistic equation under the assumption of non-relativistic speeds.
Linear kinetic energy is just one aspect of an object's total kinetic energy; rotational kinetic energy must also be considered for objects moving along a curved path or rotating.
The moment of inertia is a key factor in calculating rotational kinetic energy, taking into account an object's mass distribution and shape.
Quantum mechanics presents a different perspective on kinetic energy, treating it as a property that is only defined when a measurement is made.
The video provides a comprehensive exploration of kinetic energy across various levels of complexity, from basic physics to quantum mechanics.
The presenter encourages viewers to engage with the content by subscribing, liking, and supporting for more in-depth physics explanations.
Transcripts
Browse More Related Video

GCSE Physics - Kinetic Energy #2

Calculating kinetic energy | Modeling energy | High school physics | Khan Academy
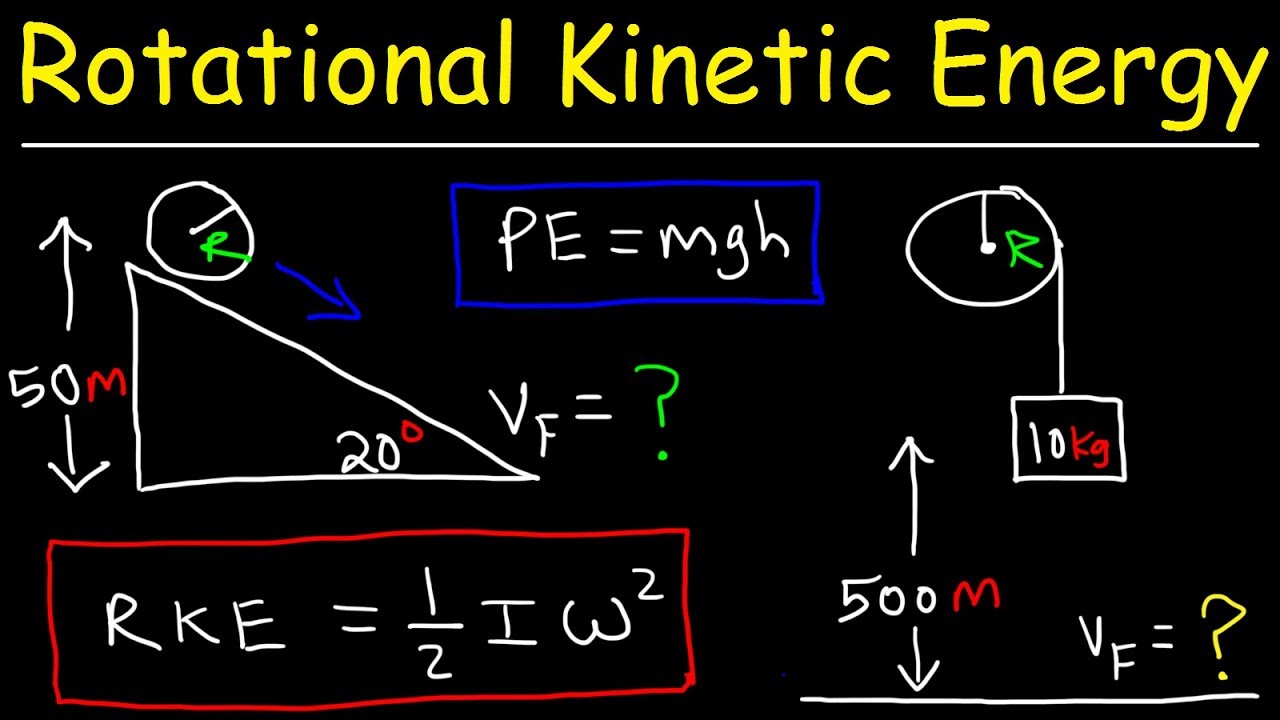
Rotational Kinetic Energy and Moment of Inertia Examples & Physics Problems

What is Kinetic Energy & Work-Energy Theorem in Physics? - [1-8]
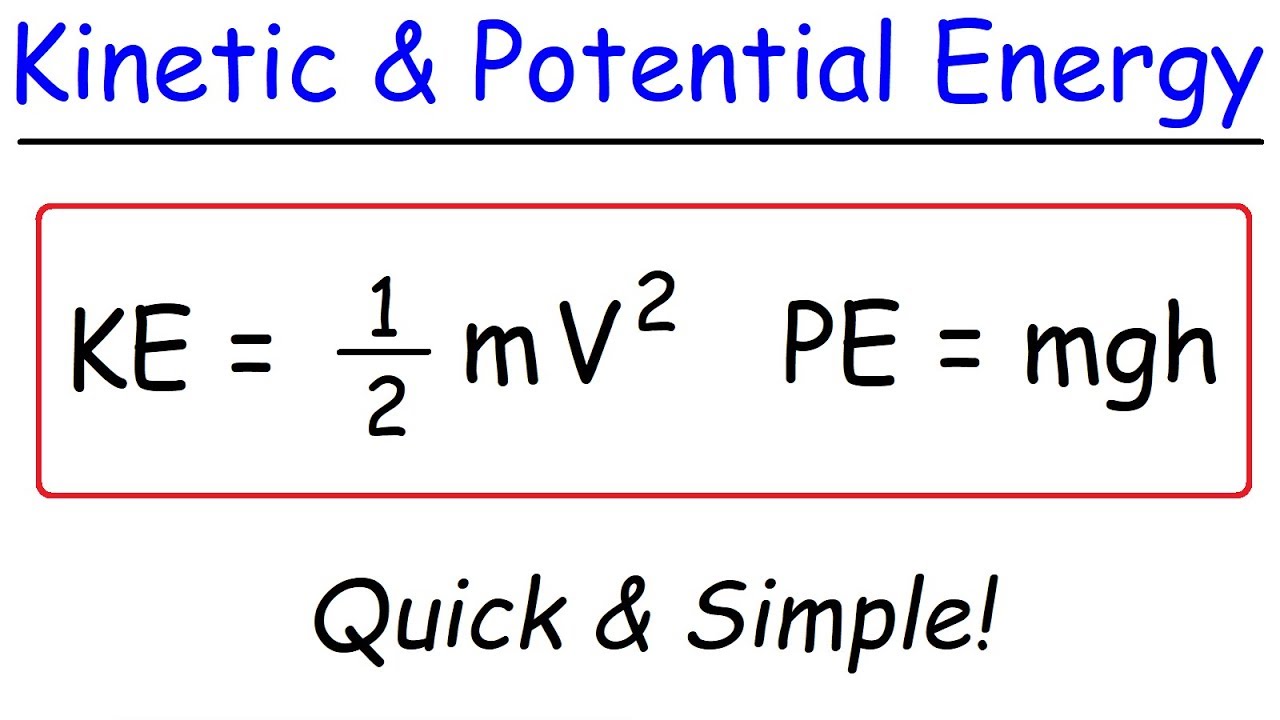
Kinetic Energy and Potential Energy

How to Calculate Kinetic Energy
5.0 / 5 (0 votes)
Thanks for rating: