Review of all concepts from AP Physics C mechanics 2020
TLDRThis comprehensive guide dives into the essential formulas needed for the AP Physics 1 Mechanics 2020 exam, emphasizing a deeper understanding beyond mere memorization. Given the exam's focus shift towards conceptual understanding, the video explores kinematics, Newton's laws, work, energy, power, momentum, and angular topics. It highlights the importance of grasping the conceptual foundations of these formulas to adeptly explain the physics principles in words. The tutorial also clarifies common misconceptions and provides strategic insights into effectively applying these concepts to solve problems, preparing students for a more conceptual and analytical approach to physics.
Takeaways
- 📊 Understanding key physics concepts is more crucial than memorizing formulas for the AP Physics II Mechanics 2020 exam.
- 📍 Topics included in the exam focus on kinematics, Newton's laws, work, energy, power, momentum, impulse, and angular concepts, excluding simple harmonic motion and gravitational potential energy derived from Newton's law.
- 🔬 Kinematic formulas are applicable only when acceleration is constant, and they can be used for any direction where this condition holds.
- 📡 Newton's laws emphasize the relationship between force and motion, with the first law stressing the importance of balanced forces for maintaining motion.
- 📈 Work and energy concepts highlight the transfer of energy and its conservation when no external work is done.
- 🤖 Momentum and impulse focus on the conservation of momentum in the absence of external impulses, highlighting the effect of forces over time on an object's motion.
- ⛓ The concept of angular momentum, including its conservation in the absence of external torques, plays a critical role in rotational dynamics.
- ⚙️ Torque and rotational inertia are key to understanding how forces cause angular acceleration and how mass distribution affects rotational motion.
- 💡 The interplay between linear and angular quantities, such as speed and angular speed, is crucial for solving problems in rotational dynamics.
- 🚨 Understanding the principles behind each formula and how they are derived provides a deeper insight into solving physics problems efficiently.
Q & A
Why is it important to understand the conceptual underpinnings of formulas for the AP Physics 2 Mechanics 2020 exam?
-Understanding the conceptual underpinnings of formulas is crucial because the exam emphasizes explaining ideas conceptually more than just performing calculations. It tests the ability to explain how formulas work and their applications in words.
What topics were still included in the AP Physics 2 Mechanics 2020 exam?
-The topics included kinematics (1D and 2D), Newton's laws including centripetal motion, work-energy and power, momentum and impulse, and angular topics.
What is the significance of understanding kinematic formulas for the AP Physics 2 exam?
-Kinematic formulas are significant because they apply under constant acceleration in any direction, essential for solving problems involving motion. Understanding these formulas and their calculus-based definitions helps in analyzing motion dynamics.
How does understanding Newton's first law assist in problem-solving for the AP Physics exam?
-Understanding Newton's first law is essential for recognizing that objects maintain their motion unless acted upon by an unbalanced force. This concept helps predict motion behaviors and solve problems involving forces and motion.
What is the role of torque and angular momentum in the AP Physics 2 Mechanics exam?
-Torque and angular momentum play crucial roles in understanding rotational dynamics. They help explain how forces cause angular acceleration and how rotational motion is conserved, which is pivotal in solving rotational motion problems.
Why is it necessary to differentiate between positive and negative work in physics?
-Differentiating between positive and negative work is necessary to understand energy transfer. Positive work indicates energy being given to an object, speeding it up, while negative work means energy is taken away, slowing it down.
How does the concept of conservation of momentum apply to collision problems in physics?
-Conservation of momentum is applied to collision problems to predict the outcome of collisions. If no external forces act on the system, the total momentum before the collision equals the total momentum after, aiding in solving collision problems.
Explain how angular motion is described by angular speed and angular acceleration.
-Angular motion is described by angular speed (omega), which is the rate of change of angle over time, and angular acceleration (alpha), which indicates how quickly angular speed changes. These concepts are essential for analyzing rotational movements.
What determines the rotational inertia of an object, and why is it important?
-The rotational inertia of an object is determined by its mass distribution relative to the axis of rotation. It's important because it influences how easily an object can be angularly accelerated, affecting its rotational dynamics.
Why is the understanding of forces and their effects on motion critical for AP Physics 2 Mechanics?
-Understanding forces and their effects on motion is critical because forces are fundamental to explaining changes in motion. Grasping how forces influence motion, speed, and direction is essential for solving a wide range of physics problems.
Outlines
📘 Overview of AP Physics 2 Exam Formulas
The speaker introduces the formulas needed for the AP Physics 2 Mechanics 2020 exam, emphasizing understanding over memorization. Key topics include kinematics, Newton's laws, work-energy-power, momentum-impulse, and angular concepts. The speaker highlights that the 2020 exam will focus on conceptual understanding due to changes in the syllabus, specifically noting that simple harmonic motion, Newton's law of gravity, and gravitational potential energy are not included in the exam. The importance of understanding the application and conceptual underpinnings of the formulas rather than just memorizing them is stressed.
🔍 Deep Dive into Kinematics
The speaker provides a detailed explanation of one-dimensional (1D) and two-dimensional (2D) kinematics, emphasizing the conditions under which the kinematic equations are applicable (constant acceleration). Concepts like velocity, acceleration, and the importance of directional consistency in calculations are discussed. The explanation transitions into how to apply these principles to various scenarios, such as objects moving along ramps, highlighting common mistakes and the importance of aligning directions in calculations. The segment concludes with a conceptual discussion of velocity and acceleration, including how acceleration affects velocity over time.
🔄 Understanding Newton's Laws
This section explores Newton's laws of motion, starting with the first law regarding the persistence of motion in the absence of external forces. The discussion emphasizes the conceptual challenges people face with this law and illustrates scenarios to clarify misunderstandings. The speaker then moves on to Newton's second law, explaining its application in calculating acceleration and addressing common pitfalls, like confusing forces with accelerations. A practical example involving air resistance demonstrates how to calculate changes in velocity over time, stressing the importance of understanding the law's implications fully.
🚀 Delving into Work, Energy, and Power
The focus shifts to the concepts of work, energy, and power. The speaker starts by defining work as the transfer of energy and explaining the significance of positive and negative work in terms of energy transfer between objects. The segment then discusses kinetic and potential energy, emphasizing the conditions under which energy is conserved. The speaker elaborates on the mathematical and conceptual aspects of power, including how it relates to the rate at which work is done and its implications for understanding energy transfer in physical systems.
🎯 Exploring Momentum and Impulse
This segment introduces momentum and impulse, explaining how they are conserved under specific conditions and their relevance in physics problems. The speaker clarifies the relationship between force, impulse, and change in momentum, using practical examples to highlight common misconceptions. The discussion underscores the importance of understanding the directional aspects of momentum and impulse, especially in the context of collisions and external forces.
🌌 Investigating Angular Motion and Torque
The discussion turns to angular motion, starting with the basics of rotational inertia and its impact on angular acceleration. The speaker explains torque, including its calculation and the factors that influence it, such as the lever arm and the angle of applied force. The importance of understanding the distinction between angular and linear quantities is emphasized, along with the conditions under which angular momentum is conserved. The speaker concludes by exploring practical applications and common errors in calculating angular motion.
📏 Applying Physics Concepts to Real-World Scenarios
In the final segment, the speaker applies the discussed physics concepts to real-world scenarios, including the comparison of sliding vs. rolling objects down an incline. The conservation of angular momentum and its implications for rotational motion are further examined. The speaker emphasizes the practical significance of these principles, encouraging students to understand the foundational concepts to solve physics problems effectively. The importance of integrating theoretical knowledge with practical application is highlighted, rounding off the discussion on preparing for the AP Physics 2 exam.
Mindmap
Keywords
💡Kinematics
💡Newton's Laws
💡Work-Energy Theorem
💡Momentum and Impulse
💡Angular Motion
💡Torque
💡Conservation Laws
💡Rotational Inertia
💡Simple Harmonic Motion
💡Gravitational Potential Energy
Highlights
Overview of formulas needed for the AP Physics II mechanics 2020 exam with an emphasis on understanding the conceptual foundations rather than memorization.
Kinematics 1d and 2d, Newton's laws including centripetal motion, work energy and power, momentum and impulse, and angular topics are still on the exam.
Simple harmonic motion, Newton's law of gravity, and gravitational potential energy derived from Newton's law of gravity are not included in the 2020 exam.
Kinematic formulas are applicable if acceleration is constant, and they can be adapted for any direction if the acceleration remains constant in that direction.
The relationship between velocity, acceleration, and their respective integrals and derivatives, highlighting the connection to calculus in understanding motion.
Newton's first law emphasizes the importance of balanced forces for an object to maintain its state of motion, challenging common misconceptions.
Newton's second law and its application to objects with forces that are functions of velocity, demonstrating the complexity of real-world physics problems.
The significance of Hookes law and spring potential energy within the context of Newton's laws and energy conservation.
Newton's third law's principle of equal and opposite forces and its implications for interactions between objects of differing masses.
The concept of work as the transfer of energy, with positive work indicating energy being given to an object and negative work indicating energy being taken from an object.
The distinction between kinetic and potential energy, and how energy conservation is a pivotal concept in physics, especially when external work is zero.
Momentum conservation as a key principle in collision problems, with the total initial momentum equaling the total final momentum in the absence of external forces.
The definition and importance of angular momentum, particularly its conservation in the absence of external torques.
The role of torque in generating angular acceleration and the relationship between linear and angular quantities.
The conservation of angular momentum illustrated through practical examples, highlighting its relevance in rotational dynamics.
Transcripts
Browse More Related Video
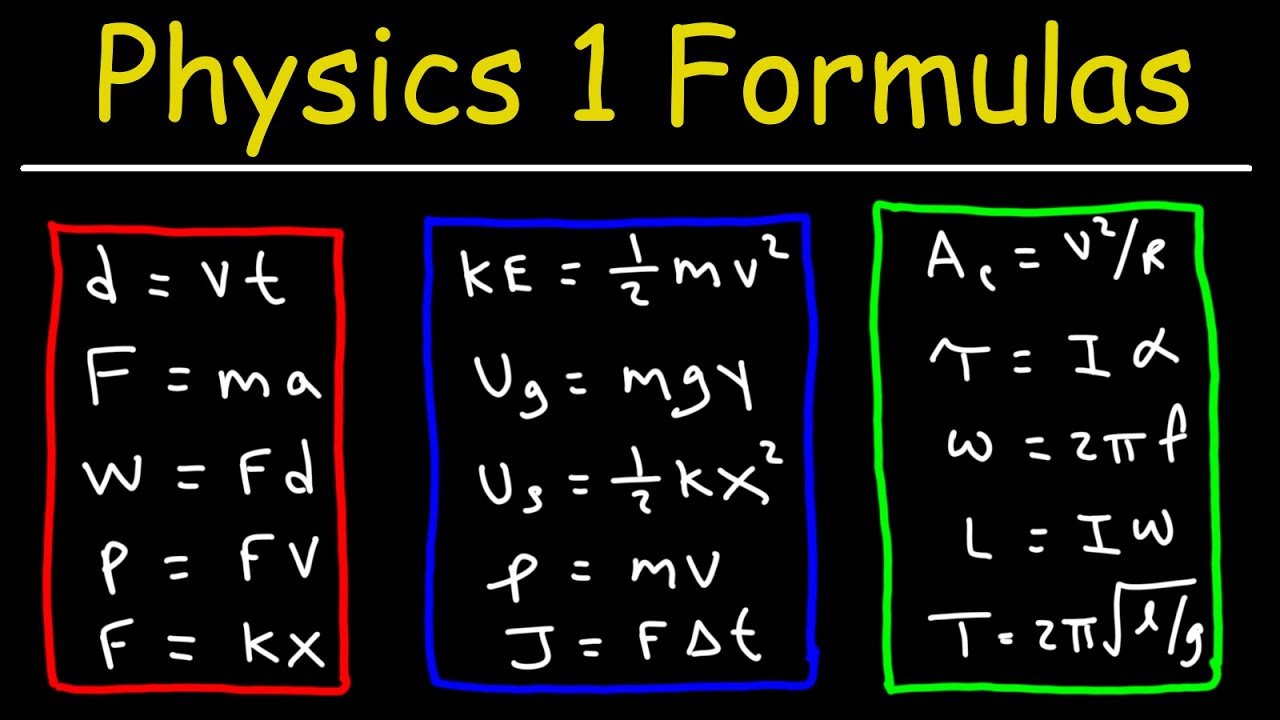
Physics 1 Formulas and Equations - Kinematics, Projectile Motion, Force, Work, Energy, Power, Moment

How to Get a 5 On AP Physics 1

2021 Live Review 8 | AP Physics 1 | Understanding Rotational Energy and Momentum

Physics 1 Final Exam Review
How to nail the hardest AP of all, AP PHYSICS 1?

MCAT Physics Equations You Need to Know to Get a Good Score
5.0 / 5 (0 votes)
Thanks for rating: