Scalar field line integral independent of path direction | Multivariable Calculus | Khan Academy

Khan Academy
5 Mar 201016:22
EducationalLearning
32 Likes 10 Comments

TLDRThe video script explores the concept of line integrals over a curve in a 2D plane, specifically addressing whether the direction of traversal (forward or reverse) affects the result of the integral of a scalar field over the curve. The explanation involves visualizing the line integral as the area of a 'curtain' with the curve as its base and the scalar field defining its height. Through intuitive reasoning and mathematical proof, it is demonstrated that the direction of travel along the curve does not influence the value of the line integral, as both forward and reverse traversals yield the same result, akin to calculating the area of the 'curtain' from different directions but arriving at the same total area.

Takeaways
  • 📈 The concept of a line integral over a curve in the x-y plane was introduced, focusing on how changing the direction of parameterization affects the result.
  • 🔄 The video compares line integrals of a scalar field over a curve and its reverse, aiming to understand if the direction of traversal matters.
  • 🤔 The intuitive idea is that the line integral should represent the area of a 'curtain' with the curve as its base and the scalar field as its height, regardless of the direction.
  • 📊 The line integral is defined as the integral of the scalar field function times the differential arc length of the curve.
  • 🌀 When reversing the curve, the derivatives of the parameterization change, but the overall integral expression should remain equivalent after proper substitution.
  • 🎨 The video uses a visual approach to illustrate the concept of line integrals, with the scalar field represented as a surface above the x-y plane.
  • 🧠 The process of parameterizing the curve and its reverse is explained, highlighting the mathematical expressions involved in each case.
  • 🔢 A detailed calculation is provided to show that, after accounting for the change in direction, the line integral expressions for the curve and its reverse are indeed the same.
  • 🌐 The video script serves as an educational tool to help viewers understand the properties of line integrals in the context of scalar fields.
  • 📚 The mathematical proof provided in the script reinforces the concept that the direction of traversal (forward or backward) does not affect the value of the line integral.
  • 🏁 The conclusion is that the line integral of a scalar field over a curve is independent of the direction in which the curve is traversed, as long as the curve itself remains unchanged.
Q & A

  • What is the main concept discussed in the video regarding parameterized curves?

    -The video discusses how parameterized curves in the xy-plane can be reparameterized to trace the same curve but in the opposite direction, and how this affects the calculation of line integrals over scalar fields along these curves.

  • How does the direction of a parameterized curve affect the line integral over a scalar field?

    -The direction of the parameterized curve does not affect the line integral over a scalar field. Whether the curve is traced in the original direction or the opposite direction, the line integral over the scalar field will yield the same result.

  • What analogy is used to visualize the concept of a line integral over a scalar field?

    -The analogy of a 'curtain' with the curve as its base and the ceiling defined by the scalar field's surface is used to visualize the line integral. This visualization helps in understanding that the line integral calculates the area of the 'curtain'.

  • What is the significance of the substitution u = a + b - t in the derivation?

    -The substitution u = a + b - t is used to simplify the expression for the line integral over the curve traced in the opposite direction. It helps in demonstrating that the line integral over the reverse curve is equivalent to the line integral over the original curve.

  • Why does swapping the boundaries of integration negate the negative sign in the integral expression?

    -Swapping the boundaries of integration from [b, a] to [a, b] negates the negative sign because, in standard integral calculus, reversing the limits of integration changes the sign of the integral. This operation cancels out the negative sign introduced by the substitution.

  • How is the integral of a scalar field over a curve mathematically represented?

    -The integral of a scalar field f(x, y) over a curve is represented as the integral of f(x(t), y(t)) times the square root of (dx/dt)^2 + (dy/dt)^2, integrated with respect to t from a to b, where x(t) and y(t) define the curve in terms of the parameter t.

  • What does ds represent in the context of the line integral?

    -In the context of the line integral, ds represents a differential element of arc length along the curve, contributing to the computation of the 'curtain's' area under the scalar field.

  • What is the impact of the direction of traversal on the line integral over a vector field, as hinted in the video?

    -While the video does not delve into the specifics, it suggests that the direction of traversal may have a different impact on the line integral over a vector field, hinting at a discussion in a subsequent video.

  • Why are the derivatives dx/dt and dy/dt necessary in calculating the line integral?

    -The derivatives dx/dt and dy/dt represent the rate of change of the x and y coordinates with respect to the parameter t, respectively. They are necessary for calculating the differential element of arc length ds, which is used in the line integral over a scalar field.

  • What conclusion can be drawn about the relationship between the direction of a curve and the line integral over a scalar field?

    -The conclusion is that the direction in which a curve is traversed does not affect the value of the line integral over a scalar field. Whether the curve is traced forwards or backwards, the integral, representing the area of a 'curtain' under the scalar field, remains the same.

Outlines
00:00
🔄 Understanding Line Integrals Over Scalar Fields in Opposite Directions

This section introduces the concept of parameterizing curves in the x-y plane to explore how line integrals of scalar fields behave when traversing a curve in opposite directions. It sets up the question of whether the direction matters when integrating over a scalar field along a curve. The video script provides a visual analogy of a curve on the x-y plane under a scalar field, comparing the area calculation (viewed as a 'curtain' area above the curve) for both directions of the curve. The intuition suggests that the direction might not affect the result of the line integral for scalar fields, contrasting with the known effect of direction on integrals in a one-dimensional context.

05:05
📐 Setting Up the Integral Formulation for Both Directions

This part dives into the mathematical setup for evaluating line integrals over a scalar field along a curve and its reverse. It details the parameterization of the curve (C) and its reverse (-C), introduces the concept of derivatives with respect to the parameter t for both curves, and formulates the integral expressions for each direction. This setup is crucial for comparing the line integrals over the curve C and its reverse, highlighting the difference in parameterization and the implications for the integrals' evaluation.

10:08
🔍 Simplifying and Comparing the Integrals

The script explains the process of simplifying the integral expression for the reverse curve (-C) through a substitution method, transforming it into a format that's directly comparable to the original curve (C) integral. This section walks through the technical steps of this simplification, including changing the integration limits and applying a substitution to show that the line integral over -C is mathematically equivalent to the integral over C. This reveals that, despite initial appearances, the line integrals over both directions of the curve yield the same result, thus proving the intuition suggested earlier.

15:09
✅ Conclusion: Directionality in Scalar Field Line Integrals

The final part of the script concludes the discussion by directly comparing the simplified integral expressions for the curve and its reverse, demonstrating their equivalence. It confirms that the direction of traversal over a curve does not affect the result of a line integral in a scalar field context, aligning with the intuition provided at the beginning. This conclusion underscores a fundamental difference in behavior between scalar field line integrals and traditional one-dimensional integrals, where direction can change the integral's sign.

Mindmap
Keywords
💡Parameterization
Parameterization involves representing a curve using a set of equations that express its coordinates as functions of a single parameter, typically 't'. In the video, this concept is essential for describing how a curve in the x-y plane can be expressed through two functions, x(t) and y(t), where 't' varies between two values, a and b. This approach allows for the exploration of curves in a more flexible manner, enabling the examination of the same curve in different directions by altering the parameterization.
💡Scalar Field
A scalar field refers to a mathematical function that assigns a scalar value (often representing a physical quantity like temperature or pressure) to every point in a space. In the video, the scalar field is visualized as a surface above the x-y plane, where each point (x, y) on the plane is associated with a height 'z', representing the scalar value. This concept is crucial for understanding the idea of a line integral over a scalar field, as it involves integrating along a curve within this field.
💡Line Integral
A line integral is a type of integral where a function is integrated along a curve. The video distinguishes between line integrals over scalar fields and vector fields. Specifically, it focuses on line integrals over scalar fields, illustrating how such integrals represent the 'area under the curve' of the scalar field along a specific path, effectively summing up the scalar field's values along that path.
💡Reverse Curve
The concept of a reverse curve refers to taking a path or curve and then tracing it back in the opposite direction. In the context of the video, it examines how the line integral over a scalar field would change (or not change) if one were to integrate over the curve in the reverse direction, thus providing insight into the intrinsic properties of line integrals over scalar fields.
💡Derivative
Derivatives play a fundamental role in the parameterization of curves and the evaluation of line integrals. In the video, derivatives of the parameterized functions x(t) and y(t), denoted as x' and y', are used to compute the differential elements along the curve, which are necessary for the line integral calculation. This highlights the importance of understanding the rate of change of the curve's coordinates with respect to the parameter 't'.
💡Substitution
Substitution is a mathematical strategy used to simplify integrals, by replacing variables with others according to a set rule. In the video, substitution is employed to transform the integral over the reverse curve into a more familiar form. This is done by introducing a new variable 'u' that simplifies the expression, allowing for direct comparison between the line integrals of the original and the reverse curves.
💡Integration Bounds
Integration bounds define the limits between which the integration is performed. In the video, changing the direction of the curve effectively swaps the integration bounds from [a, b] to [b, a]. This aspect is crucial for understanding how the integral's value is affected by the direction in which the curve is traversed, particularly in the context of reversing the curve.
💡Square Root
The square root in the line integral formula arises from the need to calculate the magnitude of the differential length element along the curve, denoted as ds. In the video, it's explained that this involves taking the square root of the sum of the squares of the derivatives of x and y with respect to 't', reflecting the geometric interpretation of the Pythagorean theorem in measuring distances along the curve.
💡Differential Area
Differential area refers to the infinitesimally small 'slice' or section of the area being considered in the process of integration. In the context of line integrals over a scalar field, the video describes how multiplying a small change in distance along the curve (ds) by the scalar field's value (height) at that point yields a differential area, which, when summed up, gives the total 'curtain area' under the scalar field along the curve.
💡Intuition and Proof
The video bridges the gap between intuition and rigorous mathematical proof. Initially, it presents an intuitive argument that the direction of traversal should not affect the value of a line integral over a scalar field, likening the integral to measuring the area of a 'curtain'. It then proceeds to prove this intuition correct through a detailed mathematical exploration, involving parameterization, substitution, and careful consideration of differential elements.
Highlights

Introduction to curve parameterization and the concept of reversing the direction of a curve.

Exploration of whether the direction of traversal affects the line integral of a scalar field.

Distinguishing line integrals over scalar fields from those over vector fields.

Visualization of line integrals as the area of a 'curtain' defined by a curve on the XY plane and a scalar field.

Intuition suggesting that the direction of traversal might not affect the integral's value for scalar fields.

Introduction of a specific curve parameterization for the reverse direction.

Explanation of calculating the derivative using the chain rule for the reverse parameterization.

Comparative analysis of line integrals over direct and reverse paths in scalar fields.

Implementation of a substitution strategy to simplify the integral calculation for the reverse path.

Clarification that swapping the integration limits negates the integral value.

Final demonstration that the line integrals for direct and reverse paths are equivalent.

Proof that the direction of traversal does not affect the line integral over a scalar field.

Confirmation of initial intuition about the independence of integral value from path direction.

Emphasis on the distinction between scalar and vector field integrals regarding path direction.

Conclusion affirming the conceptual understanding of line integrals over scalar fields with respect to curve direction.

Transcripts

Browse More Related Video

Vector field line integrals dependent on path direction | Multivariable Calculus | Khan Academy

Example of closed line integral of conservative field | Multivariable Calculus | Khan Academy

Parametrization of a reverse path | Khan Academy

Line integral example 1 | Line integrals and Green's theorem | Multivariable Calculus | Khan Academy

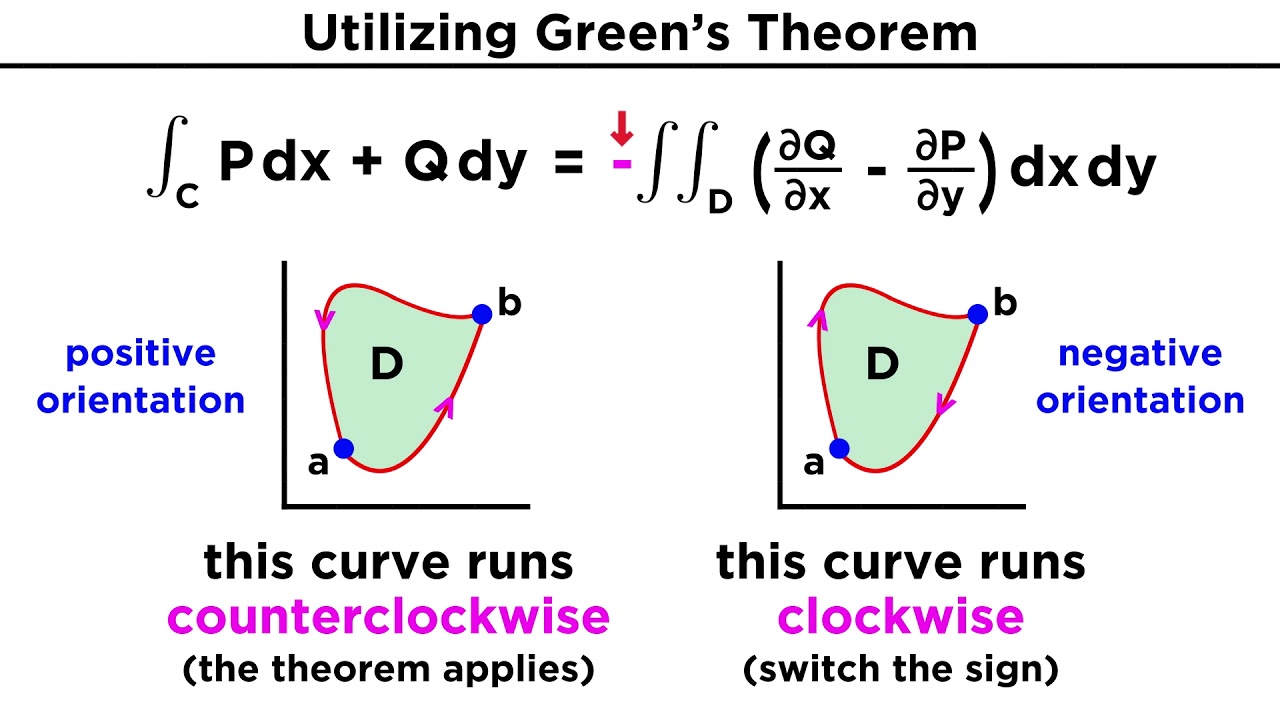
Green's Theorem

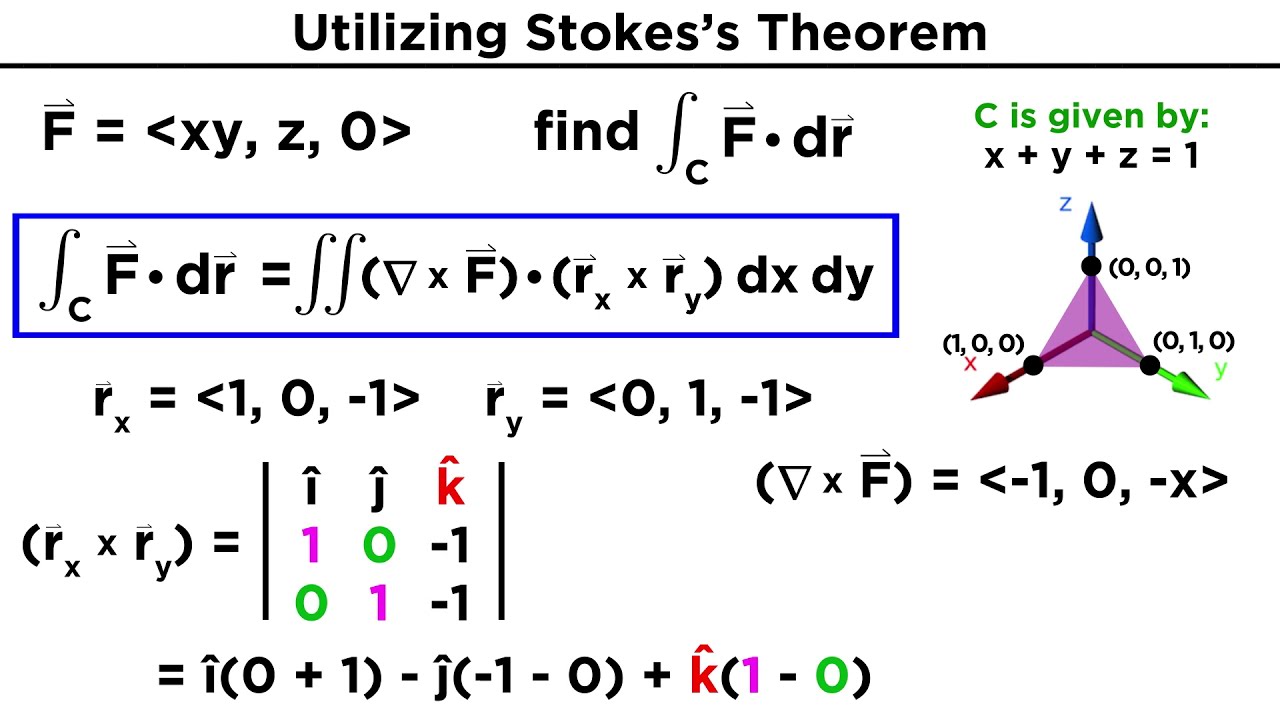
Stokes's Theorem

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
MathematicsLine IntegralsScalar FieldsDirectional ImpactVisualizationArea CalculationCurve AnalysisParameterizationDifferential EquationsIntegral Comparison