Double integrals 5 | Double and triple integrals | Multivariable Calculus | Khan Academy

Khan Academy
14 Aug 200809:51
EducationalLearning
32 Likes 10 Comments

TLDRThe video script discusses the concept of double integrals with variable boundaries, using the specific example of a surface defined by z = xy^2. The focus is on visualizing and understanding the boundaries for integration rather than the integration process itself. The example given involves integrating over a bounded domain where x ranges from 0 to 1 and the lower boundary in terms of y is a curve defined by y = x^2. The script emphasizes the importance of identifying the correct bounds for the integral and suggests that once these are established, the actual integration is relatively straightforward.

Takeaways
  • 📈 The concept of double integrals is being discussed, specifically where the boundaries on x and y are variables.
  • 🌟 The main focus of the lesson is to visualize and understand the boundaries of integration rather than the integration process itself.
  • 🔍 The difficulty in double integral problems lies in identifying the correct boundaries for integration.
  • 📊 An example is given where the surface is defined by z = xy^2 and the boundaries are not fixed but variable.
  • 🖌️ The讲师 draws the axes and the boundary on the xy-plane to help visualize the problem, emphasizing the importance of understanding the boundaries.
  • 📐 The讲师 describes the process of calculating the volume under the surface by summing up small areas (da) and multiplying by the function's value.
  • 🔄 The讲师 suggests integrating first with respect to x, then y, to find the volume under the surface.
  • 🌐 The讲师 illustrates that the lower bound of integration for x is determined by the curve y = x^2 (or x = √y).
  • 🔢 The upper bound for x is a fixed value (x = 1), while the lower bound for y is 0 and the upper bound for y is 1.
  • 📝 The讲师 writes down the double integral in terms of x and y with their respective bounds of integration.
  • 🚀 The讲师 mentions that the actual evaluation of the integral will be covered in the next video.
Q & A

  • What is the main focus of the video script?

    -The main focus of the video script is to demonstrate how to visualize and think about double integral problems where the boundaries on x and y are variables, rather than fixed constants.

  • What is the significance of visualizing the boundaries in double integral problems?

    -Visualizing the boundaries is significant in double integral problems because it helps to understand the region of integration and is crucial for setting up the correct integration limits, which is often the most challenging part of solving these problems.

  • What is the surface described in the script?

    -The surface described in the script is z = xy^2.

  • How does the script suggest approaching the integration process?

    -The script suggests approaching the integration process by first visualizing the boundaries and understanding the region of integration, then breaking the volume into small elements (dx * dy), and finally summing these elements over the defined domain.

  • What are the variable boundaries mentioned in the script for the x and y axes?

    -For the x-axis, the boundary varies from 0 to 1, and for the y-axis, the lower bound is 0, while the upper bound is 1.

  • How is the lower bound for the x-axis determined when integrating with respect to x?

    -The lower bound for the x-axis when integrating with respect to x is determined by the curve y = x^2, which represents the function x as a function of y (or x = √y).

  • What is the process for calculating the volume under the surface described?

    -The process for calculating the volume under the surface involves summing the products of small rectangular areas (dx * dy) times the height of the surface (f(xy^2)) over the defined domain, and then integrating along the remaining axis (y-axis).

  • What is the role of the curve y = x^2 in the double integral problem?

    -The curve y = x^2 serves as the lower boundary for the x-axis when integrating with respect to x, indicating where the integration starts along the x-axis for each value of y.

  • How does the script illustrate the concept of breaking down the volume into small elements?

    -The script illustrates the concept by imagining the volume as a series of small rectangles, each with a base of dx and a height of dy, and then summing these elements over the defined domain to approximate the total volume under the surface.

  • What is the final form of the double integral as presented in the script?

    -The final form of the double integral presented in the script is ∫ from y=0 to y=1 of (∫ from x=√y to x=1 of xy^2 dx) dy.

Outlines
00:00
📚 Introduction to Variable Boundaries in Double Integrals

The paragraph begins by contrasting previous double integral problems with the current one, where the boundaries for x and y are variables rather than fixed values. The focus is on visualizing and understanding the problem, as identifying the boundaries is the most challenging aspect. The example provided involves a surface defined by z = xy^2 and the goal is to find the volume under the surface, but with variable boundaries. The x-axis ranges from 0 to 1, and the y-axis is defined by a curve y = x^2, which simplifies to x = √y since we are only considering the first quadrant. The visualization of the problem is emphasized, with the speaker drawing abstract representations of the axes and the bounded domain to calculate the volume.

05:02
📈 Visualizing Integration Bounds and Setting Up the Double Integral

This paragraph delves deeper into the process of setting up the double integral with variable bounds. It emphasizes the importance of understanding the bounds of integration, which is the key to solving the problem. The speaker illustrates the concept by drawing the xy-plane and explaining how to visualize the integration process. The paragraph explains that the volume is calculated by summing up the areas of small rectangles (dx * dy) above the curve y = x^2, with the height of each rectangle determined by the function z = xy^2. The process of integrating with respect to x while holding y constant is described, with the lower bound of integration being the curve x = √y. The paragraph concludes by setting up the double integral in the order of integrating with respect to x first from 0 to 1, and then integrating with respect to y from 0 to 1. The speaker acknowledges that the actual evaluation of the integral will be covered in the next video.

Mindmap
Keywords
💡double integrals
Double integrals are a mathematical concept used to calculate the volume under a surface defined by a function of two variables, typically x and y. In the video, the focus is on understanding how to visualize and compute double integrals when the boundaries for x and y are variable, rather than fixed. This is demonstrated through the example of finding the volume under the surface z = xy^2 within specific variable bounds.
💡boundaries
In the context of double integrals, boundaries refer to the limits or edges within which the integration takes place. Determining the correct boundaries is often the most challenging part of solving a double integral problem. The video emphasizes the importance of visualizing and understanding these boundaries, which are crucial for setting up the integral correctly.
💡integration
Integration is a fundamental concept in calculus that involves finding the accumulated quantity, such as volume, under a curve. In the context of the video, integration is used to calculate the volume under a surface defined by a function z = f(xy^2). The process involves breaking down the volume into infinitesimally small layers and summing up these layers across the defined boundaries.
💡volume
Volume, in the context of the video, refers to the three-dimensional space occupied by the region under the surface defined by the function z = xy^2. The goal is to calculate this volume using double integrals by summing up the volumes of infinitesimally thin layers defined by the boundaries of integration.
💡variable bounds
Variable bounds are boundaries for the variables in an integral that are not constant values but are instead defined by functions. In the video, the lower bound for x is a function of y (x = sqrt(y)), which makes the problem more complex and emphasizes the need for a clear understanding of how these bounds interact with the function being integrated.
💡xy-plane
The xy-plane, also known as the Cartesian plane, is a two-dimensional coordinate system where each point is defined by two coordinates, x and y. In the context of the video, the xy-plane is the reference plane on which the boundaries for the double integral are drawn and where the integration domain is visualized.
💡surface
In the context of the video, a surface is a three-dimensional shape that is defined by a mathematical function z = f(x, y). The surface is what the double integral will be used to analyze, specifically to calculate the volume under this surface within certain boundaries.
💡visualization
Visualization in mathematics refers to the process of creating a mental or graphical representation of a problem to better understand and solve it. In the video, visualization is crucial for comprehending the double integral problem, particularly in determining and understanding the boundaries of integration.
💡summing
Summing, in the context of this video, refers to the process of adding together the infinitesimally small volumes (dx * dy * f(xy^2)) to find the total volume under the surface. This is the fundamental idea behind integrating, where the area or volume is built up by summing small contributions over the entire domain.
💡curve
A curve is a continuous, smooth shape in a two-dimensional plane, defined by a mathematical function. In the video, the curve y = x^2 represents the lower boundary of the region of integration in the xy-plane, which is a key part of setting up the double integral.
Highlights

The discussion focuses on double integrals with variable boundaries, which is a more complex scenario than fixed boundaries.

The main challenge in double integral problems is visualizing and understanding the boundaries, rather than the integration itself.

The example provided involves a surface defined by z = xy^2, which was previously discussed, but now with variable boundaries.

The boundaries are defined by x ranging from 0 to 1 and a curved boundary in the y direction defined by y = x^2.

The visualization of the problem is emphasized over the actual drawing of the surface, as the boundaries are the key to solving the integral.

The method of calculating the volume under the surface involves breaking it down into infinitesimally small rectangular areas (da) and summing their volumes.

The process of integrating can be done by first summing over x (integrating with respect to x) or y (integrating with respect to y).

For a fixed y, the lower bound of integration in the x direction is given by the curve x = sqrt(y), which is a variable boundary.

The upper bound for the x integration is x = 1, a straightforward constant boundary.

After calculating the volume above a single column, the next step is to sum these volumes over the entire domain by integrating with respect to y.

The lower bound for the y integration is y = 0, and the upper bound is y = 1, defining the complete region of integration.

The double integral is expressed as an integral from sqrt(y) to 1 of xy^2 dx dy, with y ranging from 0 to 1.

The video emphasizes the importance of understanding the geometric setup of the problem, which is crucial for setting up the correct integral.

The video concludes with a promise to evaluate the double integral in the subsequent video, maintaining the viewer's interest.

The approach to solving the problem is to first visualize the boundaries and then apply the principles of double integration.

The video serves as a lesson in not only mathematics but also in developing visual and spatial reasoning skills for problem-solving.

Transcripts

Browse More Related Video

Triple integrals 3 | Double and triple integrals | Multivariable Calculus | Khan Academy

Surface integral ex2 part 1: Parameterizing the surface | Multivariable Calculus | Khan Academy

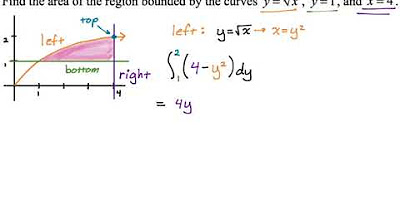
Area Between Curves: Integrating with Respect to y (Example 3)

Area Between y=x^3 and y=3x-2 | MIT 18.01SC Single Variable Calculus, Fall 2010

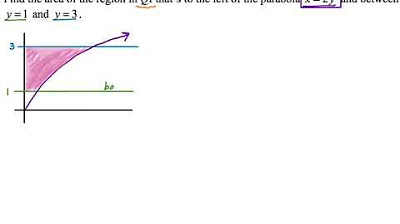
Area Between Curves: Integrating with Respect to y (Example 1)

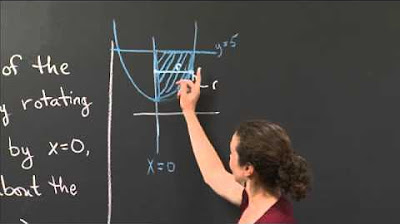
Volume of a Paraboloid via Disks | MIT 18.01SC Single Variable Calculus, Fall 2010

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Double IntegralsVariable BoundariesCalculus ConceptsIntegration TechniquesMathematical VisualizationVolume CalculationAbstract SurfacesEducational ContentProblem SolvingMathematics Tutorial