The secrets of Einstein's unknown equation – with Sean Carroll
TLDRIn this engaging lecture, the speaker explores the beauty and power of equations in physics, particularly Einstein's famous E=mc² and his theory of general relativity. The talk delves into the historical context of these equations, their mathematical intricacies, and their profound implications for our understanding of the universe, including the prediction of black holes and the curvature of spacetime. The speaker emphasizes the elegance and precision of equations, and how they reveal deeper insights into the fabric of reality.
Takeaways
- 🌟 The importance of equations in physics is highlighted, with humor suggesting that while they may seem scary, they offer a deep understanding of the universe.
- 📐 Einstein's famous equation, E=mc², is introduced as an example of the simplicity and power of equations, despite its perceived complexity.
- 🔍 The true essence of Einstein's equation for physicists is the dynamical equation describing how spacetime curvature responds to energy, mass, and momentum.
- 🎓 The lecture emphasizes the universality of physical laws, such as Newton's laws of motion and gravity, which apply to all objects regardless of their mass.
- 🌌 The concept of spacetime and its geometry, as proposed by Minkowski, is discussed as a unification of space and time into a single framework.
- 🔄 The principle of equivalence proposed by Einstein suggests that gravity and acceleration are indistinguishable, leading to the idea that gravity is not a force but a feature of spacetime geometry.
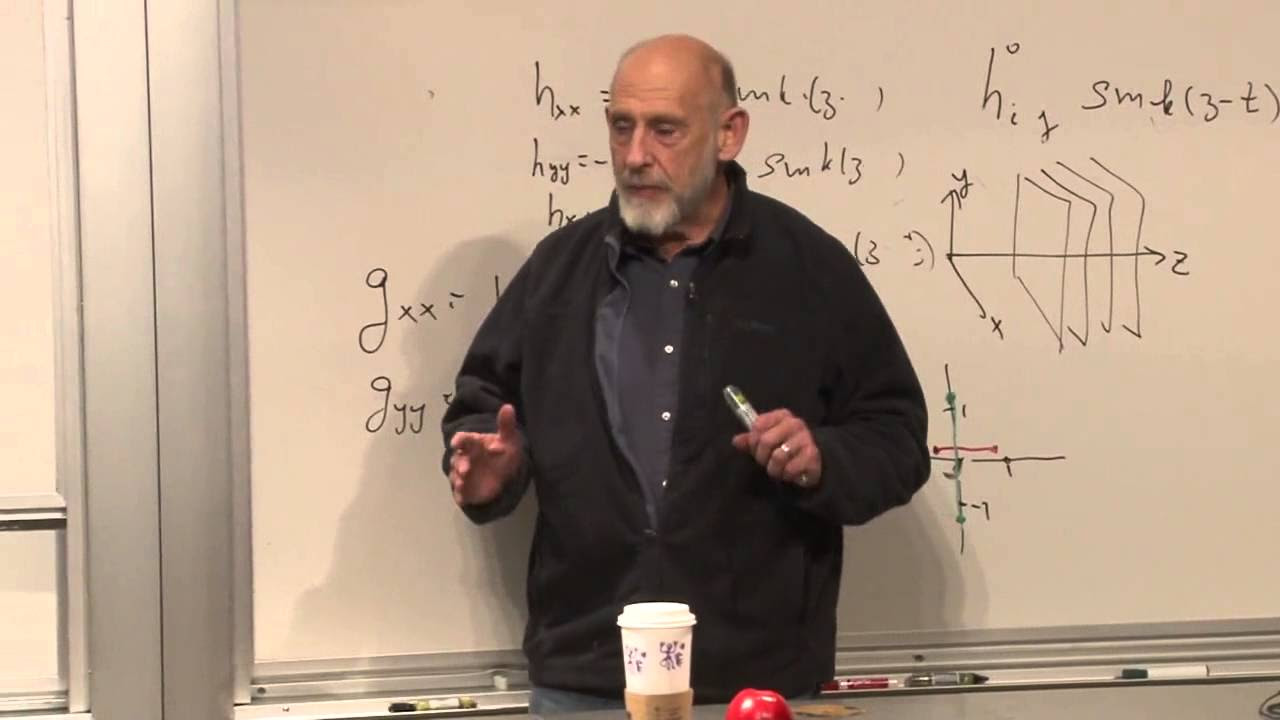
- 📚 Riemannian geometry is introduced as the mathematical foundation that Einstein used to describe the curvature of spacetime in his theory of general relativity.
- 🌐 The metric tensor and the Riemann tensor are key components in characterizing the geometry and curvature of spacetime, central to Einstein's field equations.
- 💡 The energy-momentum tensor is identified as the source of gravity in Einstein's theory, encapsulating mass, energy, momentum, and other related quantities.
- 🚀 The Schwarzschild metric is presented as a solution to Einstein's field equations, describing the spacetime geometry outside a spherical mass like a star.
- 🌑 The concept of the event horizon and black holes is touched upon, illustrating how the equations can predict phenomena not initially considered or understood by the scientists who developed them.
Q & A
What is the significance of the video screens at the Royal Institution showing various clips?
-The video screens at the Royal Institution display a variety of content, including clips of David Attenborough and other demonstrations, to showcase the fascinating aspects of science and engage the audience before a lecture. It sets the stage for the speaker to discuss the importance of equations in physics, despite their perceived complexity and the common reluctance to share them with others.
What does the equation E=mc^2 represent?
-The equation E=mc^2, given by Albert Einstein, represents the principle that the energy (E) of an object is equal to its mass (m) times the speed of light (c) squared. This equation is a fundamental concept in physics, illustrating the relationship between mass and energy and underpinning concepts such as nuclear power and the release of massive amounts of energy in nuclear reactions.
Why did Stephen Hawking say that every equation halves book sales?
-Stephen Hawking's comment about equations halving book sales reflects the common perception that mathematical equations can be intimidating and off-putting to the general public. This suggests that including complex equations in popular science books might deter some readers who find them difficult to understand, thereby reducing the potential audience and sales of the book.
What is the dynamical equation that physicists associate with Einstein?
-The dynamical equation associated with Einstein, which is less commonly shown to the public, is the Einstein field equation. It describes how the curvature of spacetime responds to the distribution of energy, mass, and momentum in the universe. The equation is typically written as Rμν - (1/2)Rgμν = (8πG/c^4)Tμν, where Rμν is the Ricci curvature tensor, R is the scalar curvature, gμν is the metric tensor, G is the gravitational constant, c is the speed of light, and Tμν is the stress-energy tensor.
What does the equation F=ma represent?
-The equation F=ma, introduced by Sir Isaac Newton, stands for the fundamental principle that the force (F) acting on an object is equal to the mass (m) of the object times its acceleration (a). This is known as Newton's second law of motion and is a cornerstone of classical mechanics, describing the relationship between force, mass, and acceleration for objects.
How did Newton's law of universal gravitation unify the understanding of gravity?
-Newton's law of universal gravitation unified the understanding of gravity by stating that the force of gravity acting between two objects is inversely proportional to the square of the distance between them and directly proportional to the product of their masses. This law showed that the same force that causes an apple to fall from a tree also governs the motion of the planets around the sun, thereby unifying the celestial and terrestrial forces under a single principle.
What is the significance of the Minkowski spacetime and its relation to special relativity?
-Minkowski spacetime is significant because it combines space and time into a single, four-dimensional continuum, which is fundamental to the understanding of special relativity. Hermann Minkowski proposed that the concepts of space and time should be merged into spacetime, which allowed for a more mathematically elegant formulation of Einstein's special relativity. This framework is crucial for understanding phenomena such as time dilation and the relativity of simultaneity.
What does the principle of equivalence state?
-The principle of equivalence, a key concept in Einstein's theory of general relativity, states that it is impossible to locally distinguish between the effects of gravity and the effects of acceleration. In other words, within a small enough region of space, the effects of gravity and the effects of being in a uniformly accelerating reference frame are indistinguishable. This principle underlies the idea that gravity is not a force but rather a curvature of spacetime caused by mass and energy.
How does the metric tensor encapsulate the geometry of spacetime?
-The metric tensor, denoted as gμν, is a fundamental object in general relativity that encodes the geometry of spacetime. It provides the information needed to calculate the length of every infinitesimal curve within spacetime, which in turn defines the curvature of spacetime. The metric tensor allows for the generalization of distance concepts, such as the Pythagorean theorem, to arbitrary geometries and is crucial for understanding the behavior of objects moving in curved spacetime.
What is the Einstein summation convention?
-The Einstein summation convention, also known as the summation convention, is a notational shorthand used in tensor calculus. It stipulates that when an index variable appears twice in a single term and is not otherwise defined, it should be summed over all of its possible values. This convention simplifies the expression of tensor operations by eliminating the need to write out summation symbols, making the equations more compact and easier to handle.
What is the significance of the Schwarzschild metric?
-The Schwarzschild metric is a solution to Einstein's field equations that describes the spacetime geometry outside a spherically symmetric, non-rotating, and uncharged massive object, such as a star or a planet. It was the first exact solution to Einstein's equations and is particularly important because it predicts the existence of the Schwarzschild radius, which is the critical radius at which an object becomes a black hole. This metric has been crucial in the development of our understanding of black holes and the behavior of objects in strong gravitational fields.
Outlines
🌟 The Marvel of Equations in Physics
This paragraph introduces the speaker's appreciation for equations in physics, highlighting their precision and power. The speaker humorously contrasts the audience's potential desire for equations with the entertaining but less informative video clips playing at the Royal Institution. The importance of equations is emphasized, with Stephen Hawking's quote about their impact on book sales. The speaker then introduces Einstein's famous E=mc² equation as an example of the beauty and accessibility of equations, despite their perceived complexity.
🚀 Newton's Equations and Their Universality
The speaker delves into Sir Isaac Newton's classical mechanics, specifically the equation F=ma, which describes the force acting on an object as proportional to its acceleration and mass. The universality of this equation is emphasized, explaining that it applies to all objects in the universe's history. The speaker also discusses Newton's law of universal gravitation, illustrating how it unifies the motion of falling apples and celestial bodies. The paragraph concludes with the historical context of these equations and their significance in understanding the universe before the advent of relativity and quantum mechanics.
🌌 Einstein's Relativity and the Geometry of Spacetime
This paragraph discusses the transition from Newtonian mechanics to Einstein's theory of relativity. The speaker explains how Einstein's special relativity, which unified space and time, led to the development of general relativity. The importance of the mathematician Hermann Minkowski is noted for his elegant formulation of spacetime. The speaker then describes Einstein's struggle with the mathematical demands of Minkowski's work and his eventual realization of the importance of spacetime geometry in understanding gravity.
🔢 The Mathematical Foundation of General Relativity
The speaker explains the mathematical foundation of general relativity, focusing on the work of mathematicians such as Riemann and Gauss. The concept of non-Euclidean geometry and its relevance to understanding the curvature of spacetime is introduced. The speaker describes how Riemann's generalization of geometry allows for the description of spacetime's curvature through the metric tensor. The complexity of these mathematical concepts is acknowledged, but their necessity for a deeper understanding of the universe is emphasized.
🌠 Einstein's Equation and the Geometry of Spacetime
The speaker continues to explore the geometric aspects of spacetime as described by Einstein's equation. The concept of the metric tensor and its role in defining the geometry of spacetime is discussed, as well as the Riemann tensor's importance in capturing spacetime's curvature. The speaker also touches on the challenges Einstein faced in formulating his equation and the eventual success of Schwarzschild in finding a solution, known as the Schwarzschild metric, which describes the spacetime outside a spherical mass.
💫 The Schwarzschild Radius and Black Holes
This paragraph discusses the Schwarzschild radius and its significance in understanding black holes. The speaker explains how the Schwarzschild metric predicts a point where time dilation becomes infinite, known as the event horizon. The speaker also talks about the historical context of black holes, from theoretical predictions to their confirmation through modern observations. The paragraph concludes with a reflection on the power of equations to reveal insights about the universe that even their originators may not have foreseen.
Mindmap
Keywords
💡Equations
💡Physics
💡Spacetime
💡Curvature
💡Metric Tensor
💡Riemann Tensor
💡General Relativity
💡Energy-Momentum Tensor
💡Schwarzschild Radius
💡Black Holes
💡Event Horizon
Highlights
The importance of equations in physics is emphasized, with the humorous remark that they are often considered scary, as Stephen Hawking noted that each equation halves book sales.
Einstein's famous equation, E=mc^2, is introduced as an icon of inscrutability, but it is explained as not being as hard to understand as it seems.
The true Einstein's equation, describing how the curvature of spacetime responds to energy, mass, and momentum, is revealed to be more complex and intimidating than E=mc^2.
The beauty of equations is compared to that of poetry, with their precision and power to provide deep understanding.
Newton's classical mechanics and his equation F=ma are discussed, highlighting the universality of force and acceleration.
The insight that the acceleration due to gravity is independent of an object's mass is considered profound and counterintuitive.
The Apollo astronauts' experiment with a hammer and a feather on the moon is mentioned, demonstrating that in the absence of air resistance, all objects fall at the same rate.
The transition from classical mechanics to the need for quantum mechanics and relativity is outlined, setting the stage for the 20th-century revolutions in physics.
Special relativity and its implications, such as time dilation, are briefly mentioned as a stepping stone to general relativity.
The significance of Hermann Minkowski's contribution to the understanding of spacetime and the unification of space and time is discussed.
Einstein's principle of equivalence is introduced, stating that gravity and acceleration are indistinguishable from each other.
The role of Marcel Grossman and Riemannian geometry in Einstein's development of general relativity is highlighted.
The concept of the metric tensor is explained as a way to capture the geometry of spacetime in a usable form.
The Riemann tensor, derived from the metric tensor, is described as capturing the curvature of spacetime.
Einstein's field equations, Rμν - 1/2 Rgμν = 8πGTμν, are presented as the correct generalization of Newton's equation for gravity in the relativistic context.
The Schwarzschild metric is introduced as the solution to Einstein's equation for a spherically symmetric, non-rotating mass.
The theoretical prediction of black holes is discussed, showing how the equations can predict phenomena not initially considered or understood by the physicists who developed them.
The importance of equations in physics is reiterated, emphasizing that they often contain insights and predictions that surpass the understanding of their creators.
Transcripts
Browse More Related Video

Warped Spacetime, Gravitational Lensing, and Gravitational Waves (Corroborating General Relativity)
General Relativity Lecture 10

Carlo Rovelli: The Man Expanding Our Understanding of the Universe | UNFILTERED

Brian Greene Explains That Whole General Relativity Thing

Beyond Einstein: Gravitational Rainbows

Sean Carroll, "The Biggest Ideas in the Universe: Space, Time, and Motion"
5.0 / 5 (0 votes)
Thanks for rating: