5.2 Mass Percents and Empirical and Molecular Weights | High School Chemistry
TLDRIn this chemistry lesson, Chad explains how to determine mass percents and empirical molecular formulas. He uses calcium carbonate as an example to illustrate calculations and emphasizes the difference between molecular and empirical formulas. The lesson also covers how to convert mass percents to empirical formulas and the importance of molar mass in determining molecular formulas.
Takeaways
- 😀 The lesson focuses on mass percents and empirical molecular formulas in the context of stoichiometry in high school chemistry.
- 📚 Chad's Prep aims to make science understandable and enjoyable, releasing weekly lessons throughout the 2020-21 school year.
- 🔔 Subscribers are encouraged to click the bell for notifications on new lesson releases.
- 📉 The script uses a 3.2-gram sample of calcium carbonate (CaCO3) to demonstrate calculations of mass percents for carbon and oxygen.
- 📊 Mass percent is an intensive property, meaning it remains the same regardless of the sample size.
- ⚖️ To find the mass percent of an element, divide the mass of that element by the total mass of the sample and multiply by 100.
- 🧪 The molar mass of a compound is used to simplify calculations by using a 100-gram sample, which corresponds to one mole.
- 📝 The empirical formula represents the simplest whole number ratio of atoms in a compound, whereas the molecular formula shows the actual number of atoms.
- 🔢 Converting mass percents to moles involves dividing the mass of each element by its molar mass to find the mole ratio.
- 🧩 To determine the empirical formula, divide the mole ratios by the smallest number to achieve whole numbers.
- 🔮 The molecular formula can be deduced from the empirical formula if the molar mass of the compound is known, as it will be a multiple of the empirical formula's molar mass.
- 📈 The script provides an example where the empirical formula CH3 could be the molecular formula or any multiple thereof, and knowing the molar mass helps to identify the exact molecular formula.
Q & A
What is the main topic of the second lesson in the stoichiometry chapter?
-The main topic of the second lesson is mass percents and empirical molecular formulas.
What is Chad's goal with his high school chemistry playlist?
-Chad's goal is to make science understandable and learning it enjoyable.
Why did Chad choose a 3.2-gram sample of calcium carbonate for the calculations?
-Chad chose a 3.2-gram sample of calcium carbonate because it has a molar mass of 100 grams, which makes the calculations easy.
What is the definition of an intensive property in the context of mass percents?
-An intensive property is one that remains the same regardless of the sample size, meaning the mass percents are the same for any size of the sample.
How does Chad calculate the percent carbon in calcium carbonate?
-Chad calculates the percent carbon by dividing the mass of carbon in one mole of calcium carbonate (12 grams) by the total mass of one mole (100 grams) and then multiplying by 100.
What is the difference between a molecular formula and an empirical formula?
-A molecular formula gives the exact number of atoms of every type in a molecule, while an empirical formula represents the most reduced whole number ratio of those atoms.
Why can't the mass percents directly represent the mole ratio in an empirical formula?
-The mass percents represent the ratio by mass, not by moles, and to get the empirical formula, a mole ratio is needed, which requires conversion from grams to moles.
How does Chad determine the empirical formula when given mass percents of elements?
-Chad first converts the mass percents to grams for a 100-gram sample, then converts these grams to moles using the molar masses of the elements, and finally divides by the smallest number to get whole numbers for the empirical formula.
What is the significance of the molar mass in determining the molecular formula from the empirical formula?
-The molar mass is crucial because it allows you to determine how many times the empirical formula must be multiplied to match the given molecular weight.
How does Chad ensure that the empirical formula is in whole numbers?
-Chad divides all the mole numbers by the smallest mole number to find a common factor, ensuring that the resulting ratio is in whole numbers.
What should you do if the empirical formula results in a fraction instead of a whole number?
-If the empirical formula results in a fraction, you should multiply all the numbers by a common factor that will convert the fraction into a whole number.
Outlines
🧪 Chemistry of Mass Percents and Empirical Formulas
The first paragraph introduces the topic of mass percents and empirical molecular formulas in the context of stoichiometry, part of Chad's high school chemistry playlist. Chad, the instructor, welcomes viewers to his educational channel, Chad's Prep, with a goal to make science understandable and enjoyable. He explains the concept of mass percents as parts over total times 100 and clarifies that these are intensive properties, meaning the sample size does not affect the result. Using calcium carbonate (CaCO3) as an example, Chad demonstrates how to calculate the mass percent of carbon and oxygen in the compound by considering the molar mass and the number of atoms in the formula. The paragraph concludes with Chad emphasizing the simplicity of calculations when using a 100-gram sample size and the concept of empirical formulas as the most reduced whole number ratio of atoms.
📚 Calculating Empirical Formulas from Mass Percents
The second paragraph delves into the process of deriving empirical formulas from given mass percents. Chad clarifies the misconception about directly using mass percents to determine the ratio of atoms in a compound. He uses an 80.0% carbon and 20.0% hydrogen compound as an example, explaining that mass percents must be converted into moles to find the mole-to-mole ratio. Chad demonstrates the calculation by choosing a 100-gram sample for simplicity and converting the grams of carbon and hydrogen into moles using their respective molar masses. He then addresses the need to adjust the resulting mole ratio to whole numbers to obtain the empirical formula, emphasizing that only whole numbers are acceptable in empirical formulas. The paragraph concludes with Chad explaining how to determine the molecular formula from the empirical formula using the molar mass of the compound.
🔍 Determining Molecular Formulas with Molar Mass
The third paragraph focuses on the distinction between empirical and molecular formulas and the process of identifying the molecular formula using the molar mass. Chad illustrates that while the empirical formula represents the simplest whole number ratio of atoms, the molecular formula may be a multiple of this empirical formula. He uses the example of a compound with an empirical formula of CH3 and a molar mass of 30 to show how doubling the empirical formula results in the molecular formula. Chad emphasizes that without the molar mass, one can only determine the empirical formula, not the molecular formula. The paragraph ends with an invitation for viewers to like, share, and comment on the video, as well as to explore Chad's premium course for further study materials.
Mindmap
Keywords
💡Mass Percents
💡Empirical Formula
💡Molecular Formula
💡Molar Mass
💡Intensive Property
💡Stoichiometry
💡Calcium Carbonate (CaCO3)
💡Benzene (C6H6)
💡Mole Ratio
💡Molecular Weight
Highlights
Introduction to mass percents and empirical molecular formulas in stoichiometry.
The concept of intensive property in chemistry and its relevance to mass percent calculations.
Explanation of how to calculate mass percent of an element in a compound using molar masses.
The convenience of using a 100-gram sample size for mass percent calculations.
The difference between molecular and empirical formulas in chemistry.
How to derive the empirical formula from mass percents without knowing the compound's formula.
The process of converting grams to moles for the calculation of empirical formulas.
The importance of using whole numbers in empirical formulas and the method to achieve this.
The potential for multiple molecular formulas based on the empirical formula.
How molar mass can be used to determine the molecular formula from the empirical formula.
The example of calculating the molecular formula for a compound with a molar mass of 30 amu.
The significance of the molar mass being a multiple of the empirical formula's mass.
The method to avoid fractions in empirical formulas by doubling or other common factors.
The caution against rounding decimals to the nearest whole number in empirical formulas.
The practical approach to stoichiometry problems demonstrated through the lesson.
Invitation for feedback, questions, and support for the educational channel.
Information about additional resources and practice problems available on chadsprep.com.
Transcripts
Browse More Related Video

Molecular and Empirical Formulas
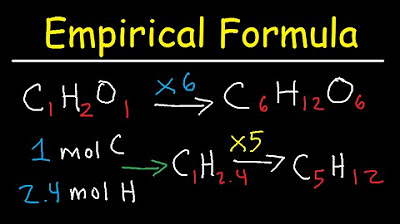
Writing Empirical Formulas From Percent Composition - Combustion Analysis Practice Problems
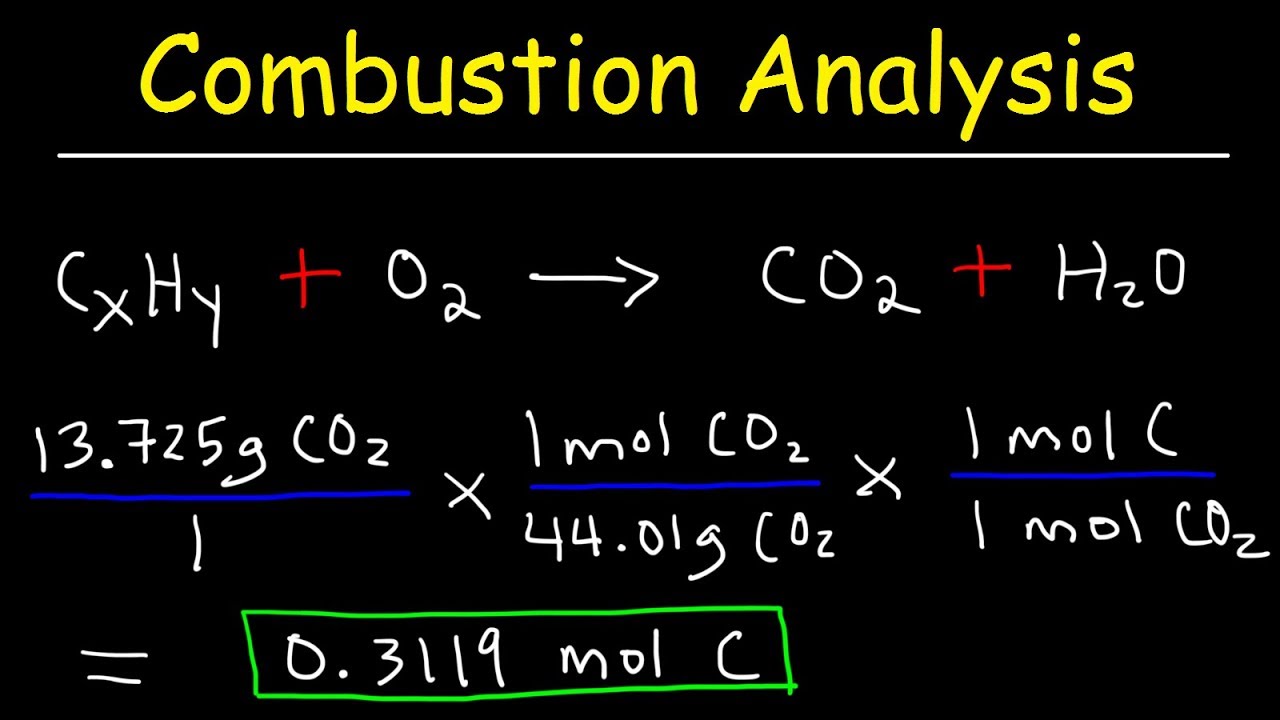
Introduction to Combustion Analysis, Empirical Formula & Molecular Formula Problems

Molecular and Empirical Forumlas from Percent Composition

Empirical Formula and Molecular Formula | Basic Concept | Numerical Problems

Empirical and Molecular Formulas from Stoichiometry
5.0 / 5 (0 votes)
Thanks for rating: