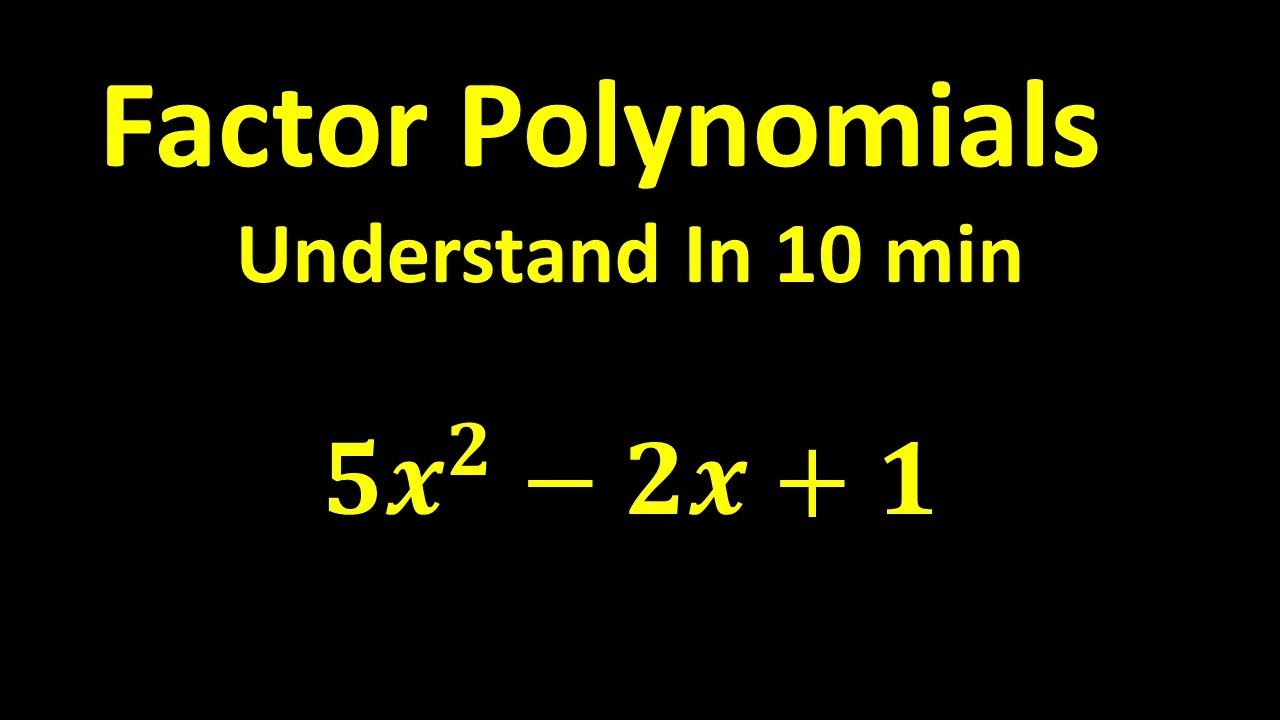
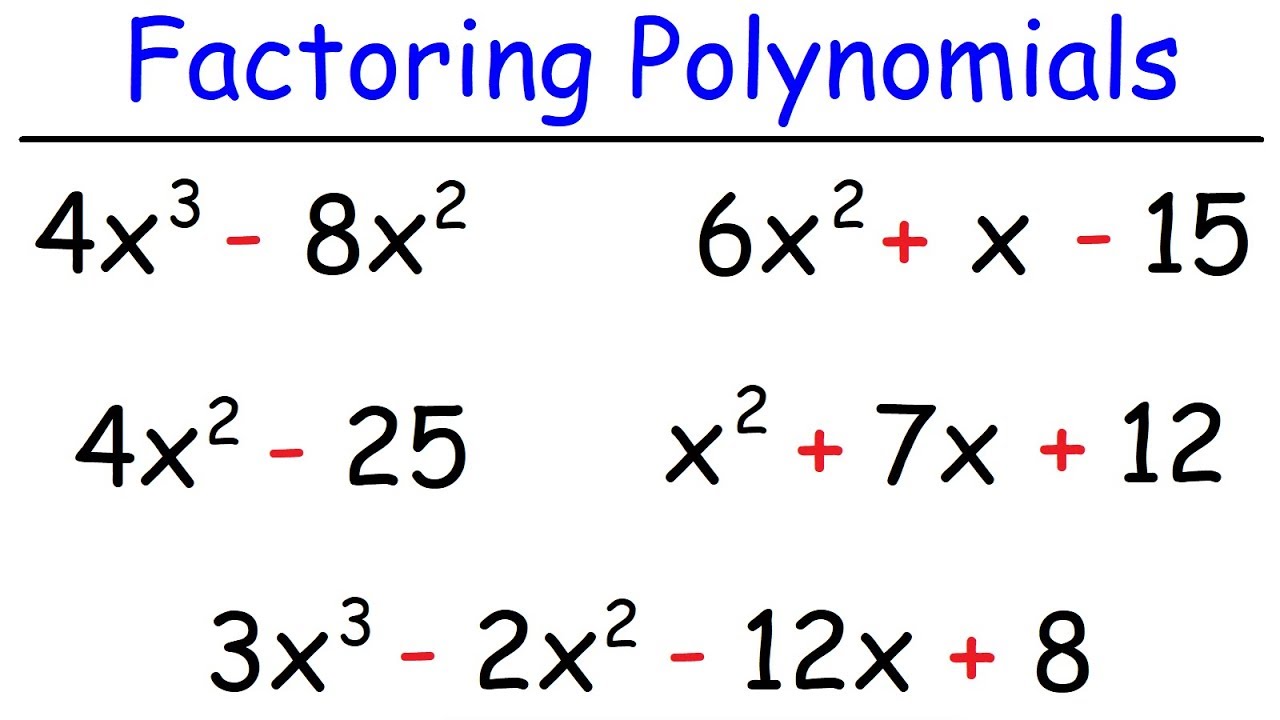
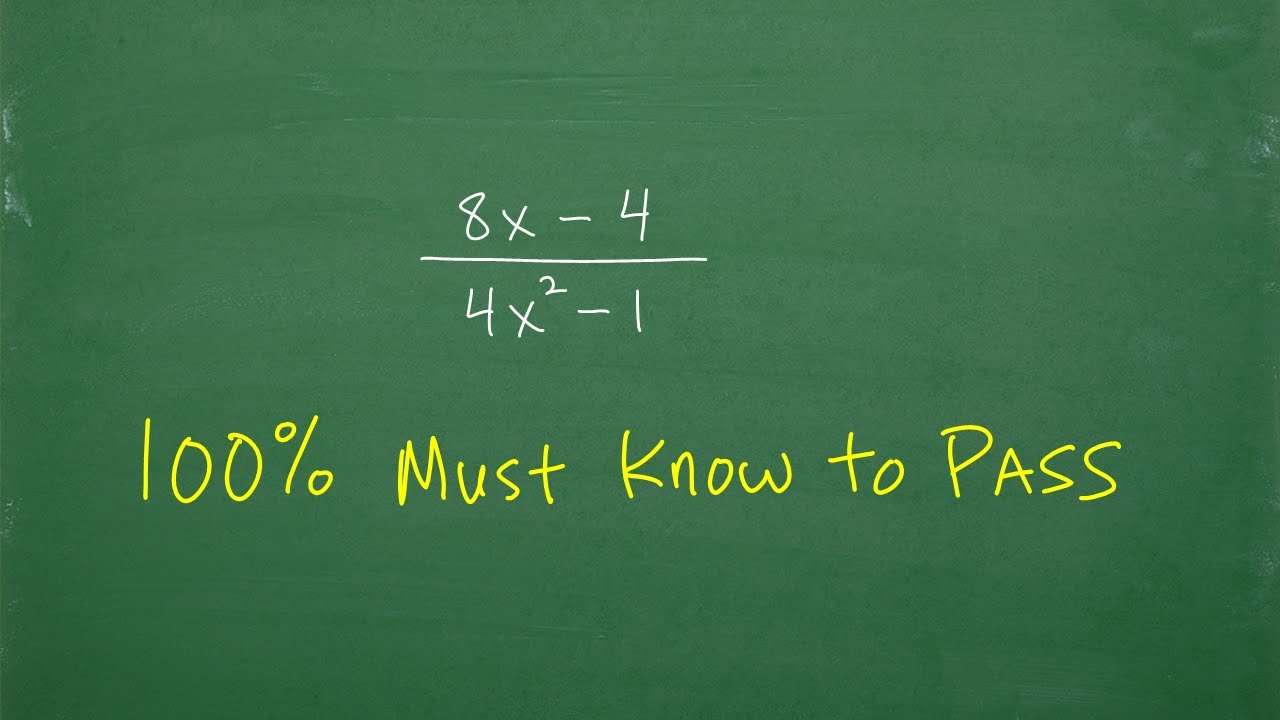Best FACTORING Hack EVER! - Part 2

This paragraph introduces the topic of the video, which is factoring trinomials with a leading coefficient other than one. It references a previous video (Part 1) that focused on trinomials with a leading coefficient of one. The speaker, John, founder of Taba Class Math and a middle/high school math teacher, emphasizes the importance of mastering factoring for success in algebra. He mentions his online math help program, which includes over 100 courses and test preparation for various exams. John also stresses the importance of note-taking for learning and offers comprehensive math notes for different levels. The paragraph concludes with a brief overview of factoring techniques, including using the greatest common factor (GCF) and special factoring rules like the difference of two squares.

The second paragraph delves into the specifics of factoring trinomials where the leading coefficient is not one. John explains that the technique used in the first part of the video series can be extended to handle these cases. He provides an example of factoring a trinomial with a leading coefficient of three and a constant term of eight, resulting in the product of 24. The process involves listing the factors of 24, looking for a pair that sums up to the middle term's coefficient (10 in this case), and then rewriting the trinomial using these factors. The goal is to find a pair of factors of the constant term that add up to the linear coefficient, which in this example is achieved with the factors 4 and 6.

This paragraph continues the detailed explanation of factoring trinomials, focusing on the step-by-step process after identifying the correct pair of factors. It describes how to rewrite the middle term using the identified factors and then proceed with group factoring. John illustrates this with the example of factoring 3y^2 + 10y + 8, showing how to break down 10y into 4y + 6y, and then factor out the greatest common factor (GCF) from each group. The process results in the factored form of (3y + 4)(y + 2). The paragraph emphasizes the importance of practice and provides reassurance that the technique will become easier over time.

The fourth paragraph presents two practice problems to apply the factoring techniques discussed earlier. John works through the problems, demonstrating how to find the correct factors of the constant term that sum up to the linear coefficient. The first problem involves factoring a trinomial with a leading coefficient of two and a constant term of ten, leading to the factored form of (2x + 5)(x + 2). The second problem involves a trinomial with a leading coefficient of three and a constant term of four, resulting in the factored form of (3a + 1)(a + 4). The paragraph encourages viewers to practice these techniques to improve their factoring skills.

In the concluding paragraph, John summarizes the importance of the factoring techniques covered in the video and encourages viewers to continue learning and practicing these skills. He reminds viewers of the significance of factoring in algebra and suggests revisiting the part 1 video for additional context. John also promotes his algebra course for a more comprehensive understanding of factoring. He ends the video by inviting viewers to like, subscribe, and follow the links in the description for more resources, emphasizing his decades of experience in teaching mathematics and his confidence in the effectiveness of the techniques presented.