Scientists Trapped Electrons In a Quantum Fractal (And It's Wild!)

Seeker
21 Feb 201905:40
EducationalLearning
32 Likes 10 Comments

TLDRThe video explores the beauty and potential applications of fractals, self-similar patterns that can be infinitely complex. It explains how fractals, both natural and mathematically generated like the Mandelbrot set, can be harnessed in technology. The script delves into fractal dimensions, such as the Koch snowflake with an infinite perimeter but finite area, and discusses real-world uses like antennas, computer simulations of natural phenomena, and molecular-level electronics. It also highlights groundbreaking research where electrons exhibit fractal behavior, suggesting a future where fractal geometries could revolutionize electronics, from stretchable devices to neural implants.

Takeaways
  • 🌿 Fractals are self-similar shapes that can be found in nature and are infinitely complex when mathematically generated.
  • 🔍 The concept of fractals includes the idea that smaller parts of a fractal resemble the whole, but in nature, they are not mathematically perfect.
  • 📚 Pure fractals are mathematically infinite and can be generated using simple formulas with recursion, like the Mandelbrot set.
  • 🔬 The Koch snowflake is an example of a fractal that has a constant size but an infinite perimeter, demonstrating the unique properties of fractal geometry.
  • 📏 Fractal mathematics is necessary to understand and measure the properties of fractals, as they cannot be accurately measured with traditional geometry tools.
  • 📶 Fractals have practical applications in modern technology, such as in the design of compact and versatile antennas for electronic devices.
  • 🧠 Fractal patterns can be used to model natural phenomena, aiding in the understanding of complex systems like the circulatory and nervous systems.
  • 🔬 Recent research has shown that fractal patterns can be created at the molecular level with electrons, opening up new possibilities for electronics.
  • 🤖 Fractal circuit elements can produce a larger output signal compared to traditional circuitry, suggesting a higher efficiency in electronic components.
  • 👨‍🔬 Fractal wire patterns can be used to create stretchable electronics, which could have applications in wearable technology.
  • 🧠 The neurons in our brain exhibit fractal patterns, and the development of fractal electronics could enhance the capabilities of neural implants.
Q & A

  • What are fractals and why are they significant?

    -Fractals are self-similar shapes that exhibit the same pattern at different scales. They are significant because they can be found in nature and have applications in various fields such as electronics, computer simulations, and even molecular-level electronics.

  • Can you give an example of a natural fractal?

    -Yes, examples of natural fractals include ferns, Romanesco broccoli, snowflakes, and the blood vessels in lungs, where each smaller part resembles a miniature version of the whole.

  • What is the difference between natural fractals and pure mathematical fractals?

    -Natural fractals are not mathematically perfect and may break down at the cellular, molecular, or atomic levels. In contrast, pure mathematical fractals are infinite and adhere to a specific pattern without deviation.

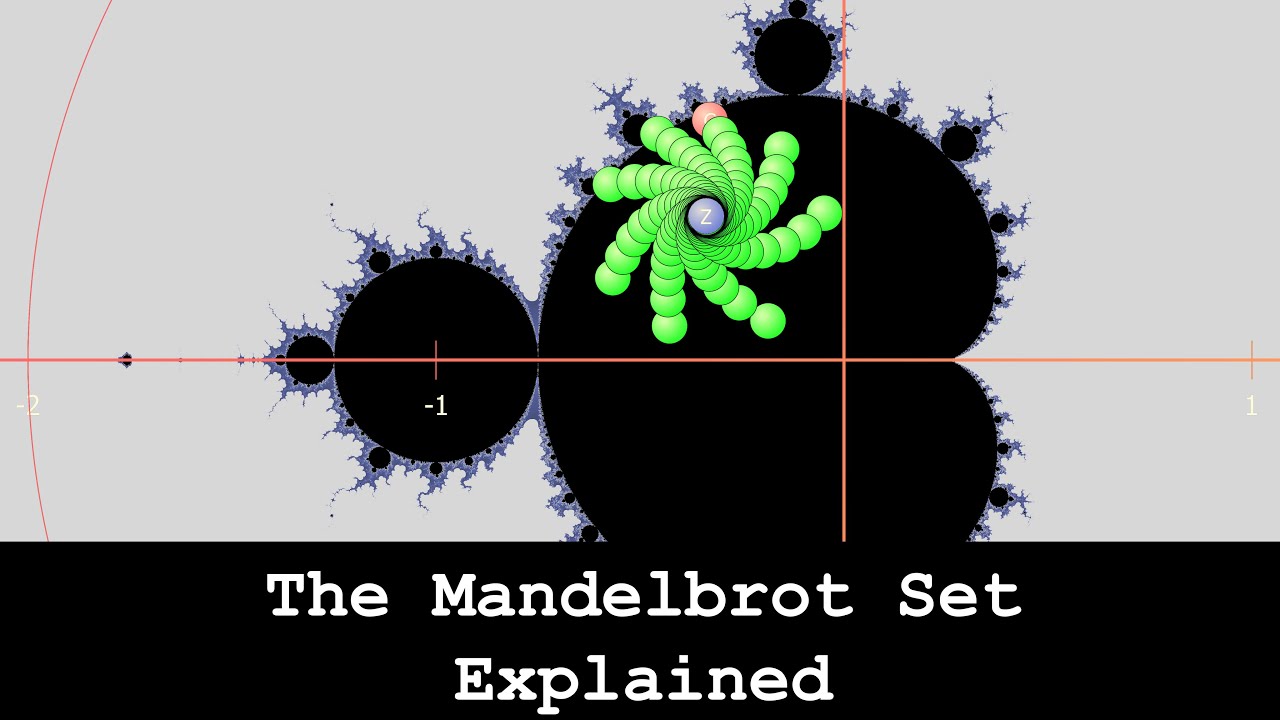
  • What is the Mandelbrot set and how is it related to fractals?

    -The Mandelbrot set is a famous visualization of a mathematical fractal. It is generated using a simple formula that includes recursion, which applies the same mathematical step to each iteration of the shape, creating an infinitely complex pattern.

  • Can you explain the concept of recursion in fractals?

    -Recursion in fractals refers to the process of applying the same mathematical step repeatedly to each iteration of the shape. This process can continue indefinitely, creating intricate and infinitely complex patterns.

  • What is the Koch snowflake and how does it demonstrate the properties of fractals?

    -The Koch snowflake is a fractal shape created by starting with an equilateral triangle and recursively adding smaller triangles to its sides. It demonstrates fractal properties by having an infinite perimeter but a finite area, and it maintains self-similarity at different scales.

  • How does the fractal dimensionality differ from regular dimensions?

    -Fractal dimensionality is a measure that indicates a fractal's complexity and self-similarity. It can be a non-integer value, unlike regular dimensions which are always whole numbers. For example, the Koch snowflake has approximately 1.26 fractal dimensions.

  • What are some real-world applications of fractals?

    -Fractals have applications in electronics, such as compact antennas that can receive multiple signal types; in computer simulations for modeling natural phenomena like circulatory and nervous systems; and in molecular-level electronics, which can improve the efficiency and versatility of electronic devices.

  • How do fractal patterns impact the design of modern antennas?

    -Fractal patterns allow for the design of compact antennas that can receive a wider range of signal types. By using fractal geometry, more shapes can be packed into a smaller space, enhancing the antenna's capabilities.

  • What is the significance of creating a fractal out of electrons?

    -Creating a fractal out of electrons, such as a Sierpinski triangle, demonstrates that electrons can exhibit fractional dimensions and behave differently based on their configuration. This could lead to advancements in the efficiency and versatility of electronic devices.

  • How can fractal electronics potentially improve neural implants?

    -Fractal electronics could improve the bioelectric interface of neural implants due to the fractal nature of neurons in our brain. This could potentially enhance the compatibility and functionality of such implants.

  • What is the potential impact of fractal geometries on the efficiency and versatility of electronics?

    -Fractal geometries can lead to larger output signals compared to input signals in electronic circuitry and enable the creation of stretchable electronics. These advancements could revolutionize the field of electronics by making devices more efficient and adaptable.

Outlines
00:00
🌐 The Beauty and Complexity of Fractals

This paragraph introduces the concept of fractals, which are self-similar patterns that can be infinitely complex. It explains how fractals can be found in nature, such as in ferns, Romanesco broccoli, snowflakes, and blood vessels, but also notes that natural fractals are not mathematically perfect. The paragraph also contrasts these natural occurrences with pure mathematical fractals, like the Mandelbrot set, which are generated using recursion. It further delves into the Koch snowflake as an example of a fractal with an infinite perimeter but finite area, highlighting the unique properties of fractals, such as their fractional dimensionality.

05:00
🔌 Real-World Applications of Fractal Mathematics

The second paragraph discusses the practical applications of fractal mathematics in various fields. It mentions how fractal thinking has been applied to antenna design, allowing for more compact and versatile devices capable of receiving a wider range of signal types, such as those used in cell phones. The paragraph also touches on how fractal patterns can be used to model natural phenomena for better understanding of systems like the circulatory and nervous systems, watersheds, and brain waves. It concludes with recent research on fractal geometries on the molecular level, such as the creation of a fractal out of electrons, which could potentially revolutionize the efficiency and versatility of electronics.

Mindmap
Keywords
💡Fractals
Fractals are complex geometric shapes that are self-similar, meaning they exhibit the same pattern at any scale. They are the central theme of the video as they are not only aesthetically pleasing but also have practical applications in various fields. The script mentions natural examples like ferns and Romanesco broccoli, illustrating how each smaller part resembles the whole. Fractals are also contrasted with pure mathematical fractals, such as the Mandelbrot set, which are generated through an infinite process of recursion.
💡Self-similarity
Self-similarity is a key characteristic of fractals, where each part of the shape is a smaller copy of the whole. This concept is crucial to understanding fractals as it allows for the infinite complexity within a finite space. The video uses the example of a fern to explain self-similarity, where each frond is a miniature version of the larger plant.
💡Mandelbrot set
The Mandelbrot set is a famous mathematical representation of fractals, named after the mathematician Benoît Mandelbrot. It is generated using a simple iterative mathematical formula that involves recursion. The video mentions the Mandelbrot set as an example of a mathematically infinite fractal, which is used to create visually captivating screensavers.
💡Recursion
Recursion is a mathematical process where a function calls itself repeatedly, applying the same step to each iteration. In the context of fractals, recursion is used to generate shapes that repeat their pattern infinitely. The script refers to recursion when explaining the generation of the Mandelbrot set and the Koch snowflake, emphasizing its role in creating complex patterns from simple rules.
💡Koch snowflake
The Koch snowflake is a specific type of fractal that is created by repeatedly applying a simple rule to an initial shape, in this case, an equilateral triangle. The video describes the process of creating the Koch snowflake by replacing each line segment with four segments, each one-third the size of the original, and repeating this process infinitely. This fractal is highlighted for its unique property of having an infinite perimeter but a finite area.
💡Fractal dimension
Fractal dimension is a measure that characterizes the complexity and scaling properties of fractals. Unlike traditional dimensions, which are whole numbers, fractal dimensions can be non-integer values. The video explains that the Koch snowflake has a fractal dimension of approximately 1.26, indicating that it is fractionally dimensional and more complex than simple geometric shapes.
💡Antenna
In the context of the video, an antenna is a device that receives signals within a certain range of frequencies. The script discusses how fractal mathematics can be applied to antenna design, allowing for more compact devices that can receive a wider variety of signals. This application of fractals in technology is an example of how mathematical concepts can be translated into practical solutions.
💡Fractal circuit elements
The video mentions fractal circuit elements as a way to enhance the efficiency of electronics. These elements can produce a much larger output signal compared to traditional circuitry, suggesting that fractal geometries can improve the performance of electronic devices. This concept is an example of how fractals can be harnessed for technological advancements.
💡Stretchable electronics
Stretchable electronics are a novel application of fractal patterns that allow for the creation of flexible devices that can be worn on the skin. The video suggests that fractal wire patterns can be used to develop such electronics, which could have numerous applications in wearable technology and health monitoring.
💡Neural implants
Neural implants are devices that interact with the nervous system, often used for medical purposes. The video discusses how the fractal nature of neurons in the brain could limit the effectiveness of neural implants. However, by using fractal electronics, the bioelectric interface with the nervous system could be significantly improved, expanding the potential applications of such implants.
💡Sierpinski triangle
The Sierpinski triangle, also known as the Sierpinski gasket, is another example of a fractal that is created through a recursive process. The video describes a study where researchers were able to create a fractal pattern with electrons that behaved as if they were in 1.58 dimensions, which is the fractional dimension expected of a Sierpinski triangle. This illustrates the potential for fractals to influence the behavior of matter at the molecular level.
Highlights

Fractals are self-similar shapes that appear the same when zoomed in.

Fractals in nature are not mathematically perfect and break down at cellular, molecular, and atomic levels.

Pure mathematical fractals, like the Mandelbrot set, are infinite and generated through recursion.

The Koch snowflake is a fractal with a constant size but an increasing perimeter, theoretically infinite.

Fractal mathematics is necessary to measure complex shapes like the Koch snowflake.

Fractals have a fractional dimension, like the Koch snowflake with approximately 1.26 dimensions.

Fractal geometry is applied to modern antennas for compactness and versatility.

Fractal patterns can be used to model natural phenomena for better understanding of systems like the circulatory and nervous systems.

Fractal geometries can be found on molecular levels, influencing electron behavior.

Researchers have created a fractal out of electrons, the Sierpinski triangle, using a scanning tunneling microscope.

Fractal circuit elements can produce a larger output signal compared to traditional circuitry.

Fractal wire patterns are used to create stretchable electronics for wearable technology.

Fractal electronics could enhance the bioelectric interface of neural implants.

The potential applications of fractals in electronics are vast and largely unexplored.

Stay updated on the latest in fractal research and its applications by subscribing to the channel.

Transcripts

Browse More Related Video

Fractals are typically not self-similar

Sounds of the Mandelbrot Set

16. Fractals I

16.5 Fractals II

FRACTALS IN MUSIC - Exploration & Experiment

The Mandelbrot Set Explained

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
FractalsElectronicsMandelbrot SetKoch SnowflakeRecursive PatternsFractal MathematicsNatural PhenomenaSierpinski TriangleNeural ImplantsStretchable Electronics