Distance and Displacement | Physics

TeachTalk
17 Oct 202011:40
EducationalLearning
32 Likes 10 Comments

TLDRThe video script from 'Teach Talk' introduces viewers to the concepts of distance and displacement in a fun and educational manner. It explains that distance is a scalar quantity, representing the total path length traveled, while displacement is a vector quantity that includes both magnitude and direction from the initial to the final position. The script uses a boy going to school and a person walking east then north to illustrate these concepts. It demonstrates how to calculate displacement using the Pythagorean theorem for a right triangle and how to determine direction with a compass and protractor. A sample problem is solved, and viewers are encouraged to practice these skills. The video concludes with a quiz, reinforcing the importance of understanding distance and displacement in physics.

Takeaways
  • 📚 The video is from 'Teach Talk', a channel that makes learning fun and easy, focusing on science topics from grade 7 to grade 12.
  • 🔥 Viewers are encouraged to subscribe and hit the notification bell to stay updated on new videos.
  • 📍 The video introduces basic concepts of distance and displacement, emphasizing their differences and applications in physics.
  • 🚨 Distance is described as a scalar quantity representing the total path covered, without direction.
  • 🚩 Displacement is defined as a vector quantity, indicating the shortest path between two points, inclusive of magnitude and direction.
  • 🔫 Practical examples and problems are used to illustrate how to calculate distance and displacement, including the use of the Pythagorean theorem.
  • 📲 Tools like compasses and protractors are shown as essential for finding direction and angles in displacement calculations.
  • 💾 The channel plans to cover more advanced topics, including trigonometric functions in future videos.
  • 🎓 A sample problem is solved step-by-step to demonstrate how to find both distance and displacement in a specific scenario involving movement around a rectangular track.
  • 🚀 The video concludes with a quiz to engage viewers and test their understanding of the concepts discussed.
Q & A

  • What are the main topics covered by Teach Talk?

    -Teach Talk covers topics in general science, biology, chemistry, physics, and earth science for grades 7 to 12.

  • What is the difference between distance and displacement?

    -Distance is the total length of the path taken during a journey, while displacement is the straight-line distance between the starting and ending points, including direction.

  • What type of quantity is distance considered to be?

    -Distance is considered a scalar quantity, which means it only has magnitude without direction.

  • How is displacement represented in terms of magnitude and direction?

    -Displacement is represented as a vector quantity, which includes both magnitude and direction. It is often described using a compass and a protractor to determine the specific angle.

  • What is the formula used to find the displacement when the path forms a right triangle?

    -The Pythagorean theorem is used to find the displacement in a right triangle, where c^2 = a^2 + b^2, with c being the hypotenuse (displacement), and a and b being the legs of the triangle.

  • How can one find the direction of displacement using a compass?

    -A compass can be used to find the general direction (e.g., north, south, east, west), and a protractor can be used to find the specific angle for a more precise direction.

  • What is the distance covered when a person walks 4 meters east and then 3 meters north?

    -The total distance covered is the sum of the two individual distances, which is 4 meters + 3 meters = 7 meters.

  • How is the displacement calculated for a person who walks 4 meters east and then 3 meters north?

    -The displacement is calculated using the Pythagorean theorem to find the hypotenuse of the right triangle formed by the path, which results in a magnitude of 5 meters at an angle of approximately 36 degrees north east.

  • What is the formula for the perimeter of a rectangle?

    -The formula for the perimeter of a rectangle is 2(length + width), or 2l + 2w, where l is the length and w is the width.

  • In the sample problem, why is the displacement of the runner who travels around a rectangle track twice and then back to the starting point zero?

    -The displacement is zero because the runner ends up at the starting point, which is also the initial point, making the straight-line distance between the final and initial points zero.

  • What is the purpose of the short quiz at the end of the Teach Talk video?

    -The short quiz is designed to test the viewer's understanding of the concepts presented in the video, specifically distance and displacement, and to reinforce learning through practical problem-solving.

Outlines
00:00
📚 Introduction to Distance and Displacement

This paragraph introduces the topic of distance and displacement in the context of a student's journey to school. It explains that distance is the total length of the path taken and is a scalar quantity, meaning it only has magnitude without direction. Displacement, on the other hand, is the shortest distance between the starting and ending points and is a vector quantity, which includes both magnitude and direction. The paragraph uses the example of a boy walking to school to illustrate these concepts and introduces the use of a compass and protractor to determine direction and angle, respectively. It concludes with a sample problem involving a person walking east and then north to calculate both distance and displacement using the Pythagorean theorem.

05:00
🧮 Solving for Displacement with Direction

This paragraph continues the discussion on displacement by providing a method to find the direction using a compass and a protractor. It presents a sample problem where a person walks 4 meters east and then 3 meters north, forming a right triangle. The paragraph explains how to use the Pythagorean theorem to find the magnitude of displacement and then uses a compass to determine the direction, which is found to be 36 degrees north east or north of east. The paragraph emphasizes the importance of understanding both the magnitude and direction of displacement for a complete understanding of the concept.

10:03
🏃‍♂️ Runner's Distance and Displacement on a Rectangle Track

The final paragraph presents a problem involving a runner who travels around a rectangle track twice and then returns to the starting point. The problem provides the length and width of the rectangle and asks to determine both the distance and displacement. The paragraph explains that the distance is calculated by finding the perimeter of the rectangle and then multiplying by two, since the runner goes around twice. The displacement, however, is zero since the runner ends up at the starting point. This problem reinforces the concepts of distance as a scalar quantity and displacement as a vector quantity, with a focus on the initial and final positions.

Mindmap
Keywords
💡Distance
Distance is defined as the length of the path traveled by an object. In the context of the video, it is a scalar quantity, meaning it only has magnitude and no direction. For instance, when the boy goes to school, the entire path he walks is considered the distance covered, which is an essential concept in understanding motion and travel.
💡Displacement
Displacement refers to the shortest distance between an object's initial and final positions. It is a vector quantity, which means it has both magnitude and direction. The video uses the example of a student's movement from home to school to illustrate displacement, highlighting that it is the straight line distance from the starting point to the ending point, regardless of the actual path taken.
💡Scalar Quantity
A scalar quantity is a physical quantity that has magnitude but no direction. In the video, distance is described as a scalar because it only involves the total length of the path without considering the direction of travel. This is contrasted with vector quantities, which have both magnitude and direction.
💡Vector Quantity
A vector quantity has both magnitude and direction. Displacement is given as an example of a vector quantity in the video. It is important in physics because it conveys the full description of an object's change in position, including the direction of movement.
💡Reference Point
A reference point is a fixed location used as a baseline for measuring distances or displacements. In the script, the student's home is used as the reference point to determine the displacement to the school. It is crucial for establishing a starting point from which all movements are measured.
💡
💡Compass
A compass is a tool used for navigation and orientation that shows direction. In the video, a compass is mentioned for determining the direction of displacement. It helps to establish the orientation of the movement, such as southeast or northeast, which is part of describing the vector quantity of displacement.
💡Protractor
A protractor is an instrument used to measure angles. In the context of the video, it is used to find the specific angle of direction when determining displacement. For example, the angle of 30 degrees to the southeast is found using a protractor to quantify the direction component of the vector.
💡Pythagorean Theorem
The Pythagorean theorem is a mathematical principle used to find the length of the hypotenuse of a right-angled triangle. It is relevant in the video when calculating the magnitude of displacement, which forms a right triangle with the paths walked east and north as the other two sides.
💡Hypotenuse
The hypotenuse is the longest side of a right-angled triangle, opposite the right angle. In the video, the hypotenuse represents the displacement when the path forms a right triangle. It is calculated using the Pythagorean theorem to find the straight-line distance between two points.
💡Sample Problem
A sample problem is an example question used to illustrate how to apply a concept or solve a particular type of problem. In the video, a sample problem is provided to demonstrate how to calculate both distance and displacement when a person walks 4 meters east and then 3 meters north.
💡Trigonometric Functions
Trigonometric functions are mathematical functions of angles in a right-angled triangle. The video mentions that these functions will be discussed in more advanced videos, implying their use in calculating angles and other related quantities in physics problems.
💡Quiz
A quiz is a form of assessment consisting of a series of questions or problems designed to test knowledge. In the video, a short quiz is proposed to the audience to reinforce their understanding of distance and displacement concepts taught in the video.
Highlights

Introduction to Teach Talk, a channel that makes learning fun and easy.

Welcome to Shenzhen and an overview of topics covered from grade 7 to grade 12 in various science subjects.

Explanation of the concept of distance as the length of the path covered, illustrated with a student going to school.

Distance is described as a scalar quantity, having only magnitude without direction.

Introduction to displacement as the shortest distance between the initial and final position.

Displacement is a vector quantity, which includes both magnitude and direction.

Use of a compass to determine the direction of displacement.

Employing a protractor to find a more specific direction, illustrated with an example of 30 degrees.

Practice with a protractor before delving into trigonometric functions in advanced science videos.

Summary of the boy's displacement from his house to school as 10 meters at 30 degrees to the southeast.

Sample problem: A person walks 4 meters east then 3 meters north to determine distance and displacement.

Calculation of distance as a simple addition of the lengths walked.

Use of the Pythagorean theorem to find the magnitude of displacement in a right triangle scenario.

Determination of displacement direction using a compass and protractor, resulting in 5 meters at 36 degrees north east.

Recap of the concepts of distance and displacement, including how to find them and a sample problem solution.

Short quiz presented to the audience to test their understanding of distance and displacement.

Problem-solving approach for a runner traveling around a rectangle track twice and then back to the starting point.

Explanation of how to calculate the perimeter of a rectangle and multiply by two for the runner's distance.

Final determination that the runner's displacement is zero meters since they return to the starting point.

Invitation for viewers to comment on the difficulty of the problem and to engage with the Teach Talk community.

Closing remarks encouraging subscription, liking, sharing, and continuing the learning journey together.

Transcripts

Browse More Related Video

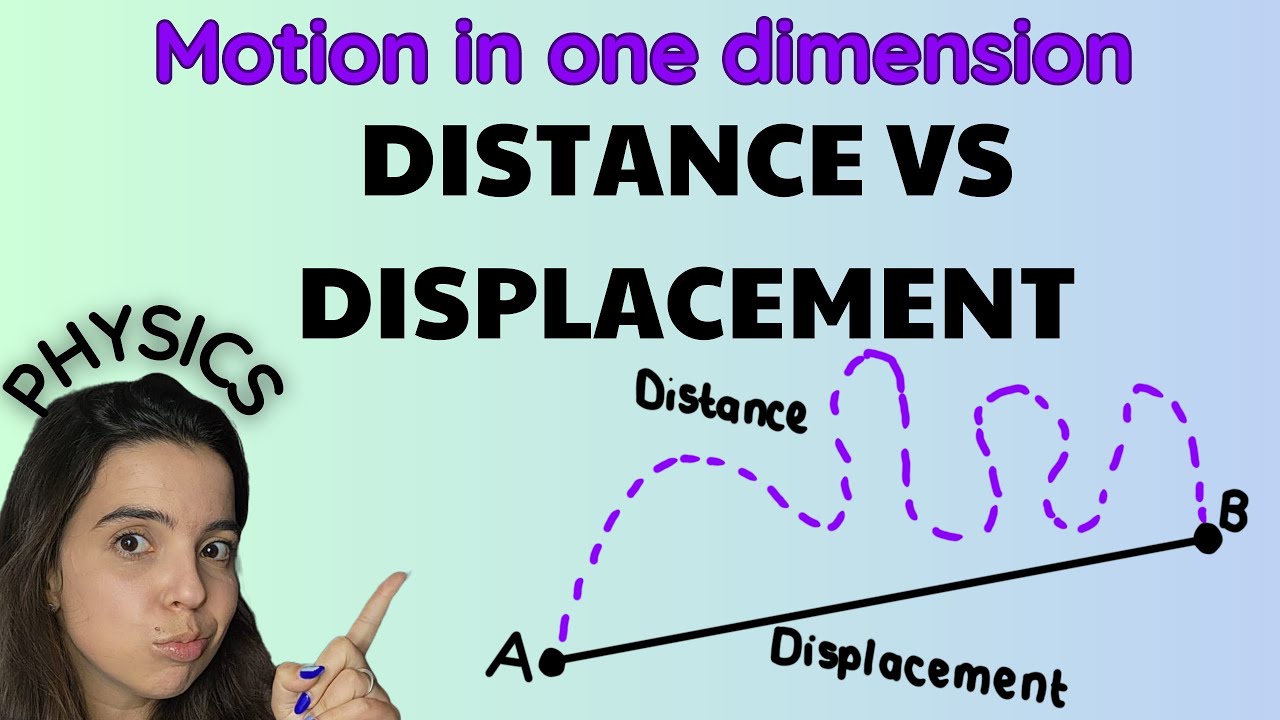
Displacement and Distance: Motion in One Dimension

Displacement and Velocity - How is it different from Distance and Speed? | Physics

Distance Versus Displacement

Distance vs. Displacement

10 - Learn Distance and Displacement in Physics (Displacement Formula Vs. Distance Formula)

What Are Distance and Displacement? | Physics in Motion

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Physics EducationTeacher ChainDistance LearningDisplacementScalar VectorScience ConceptsTrigonometryPythagorean TheoremEducational VideosStudent EngagementScience TutorialInteractive Learning