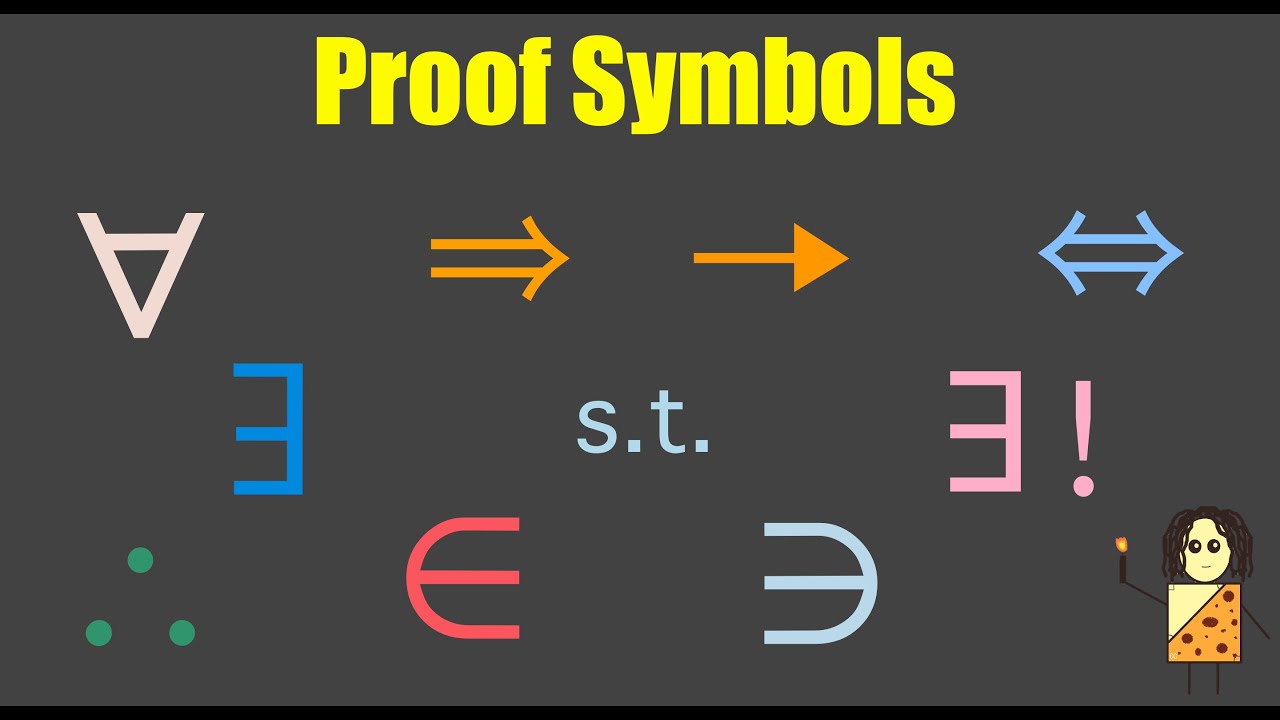
Mathematical Proof Writing
TLDRThe video script emphasizes the importance of understanding and mastering mathematical proofs to truly appreciate and excel in mathematics. The speaker shares their personal journey and transformation from enjoying calculus to falling in love with the beauty of mathematical logic and proofs. They discuss the challenges many face in grasping proof writing and offer reassurance that the struggle is normal. The script provides various tips and resources for learning to write proofs, including taking classes, studying under good professors, and utilizing books and online resources. The speaker also highlights the significance of feedback in improving proof-writing skills and encourages persistence, as learning to write proofs opens up the world of advanced mathematics.
Takeaways
- 📚 **Learning Proofs**: Understanding how to write mathematical proofs is a pivotal moment in appreciating the beauty of mathematics.
- 🤔 **Initial Struggles**: It's normal to struggle with proofs initially, and disliking them often stems from not understanding them well.
- 💡 **Understanding is Key**: Once the structure of proofs is understood, the process becomes more intuitive and enjoyable.
- 🎓 **Educational Resources**: Learning from the best sources, such as well-written books or quality professors, is crucial for mastering proof writing.
- 👨🏫 **Professor's Role**: A good professor who can write clear proofs is almost a necessity for most people to learn how to write proofs effectively.
- 📈 **Progression**: As proof-writing skills improve, one can tackle more advanced mathematics with a solid foundation.
- 📈 **Evolution Over Time**: Proof writing is a skill that evolves; it doesn't become easy overnight but improves with practice and time.
- 📚 **Multiple Sources**: It's beneficial to have access to various proof books to see different explanations and styles of proof writing.
- 📝 **Feedback Crucial**: Receiving feedback on your proofs is essential for learning and improvement, which is often best facilitated through a class setting.
- 🌐 **Online Options**: For those who cannot attend in-person classes, online courses can provide structure, accountability, and valuable feedback.
- 📈 **Proof Writing for Degree**: Mastering proof writing is a gateway to obtaining a degree in mathematics, as it is a fundamental skill across advanced math subjects.
Q & A
What is the key to truly appreciating and enjoying mathematics according to the speaker?
-The key to truly appreciating and enjoying mathematics is understanding how to write mathematical proofs. Once a person grasps the structure and logic behind proofs, it can significantly enhance their love for the subject.
Why do some people struggle with learning mathematical proofs?
-Some people struggle with learning mathematical proofs because it involves a different way of thinking compared to computational math. It requires understanding the structure and logic behind proving statements, which can be challenging and may not click easily for everyone.
What is the speaker's opinion on the best way to learn to write proofs?
-The speaker believes that the best way to learn to write proofs is by taking a class, ideally a face-to-face college class with a good professor who can provide clean proofs and valuable feedback.
Why are books recommended for learning how to write proofs?
-Books are recommended because they provide structured explanations and examples of proofs. However, the speaker also notes that once a person knows how to write proofs, they might find their own proofs more clear and clean, indicating a deeper understanding.
What is the advantage of having multiple sources or professors for learning proofs?
-Having multiple sources or professors can be beneficial because it exposes the learner to different styles and methods of proof writing. This can help in developing a more robust and versatile understanding of proofs.
Why does the speaker suggest getting as many proof books as one can afford?
-The speaker suggests getting multiple books because each book might explain a proof differently, and having various perspectives can help in understanding the logic and structure better. It also provides a chance to compare different explanations and find the one that clicks for the learner.
What does the speaker mean by 'feedback is critical for learning to write proofs'?
-Feedback is critical because it helps learners understand their mistakes and areas of improvement. It provides a way to correct misunderstandings and reinforces the correct approach to writing proofs.
Why does the speaker mention that learning to write proofs can make all of mathematics accessible?
-Once a person learns to write proofs, they have the foundational skills necessary to tackle more advanced areas of mathematics. Proof writing is a core skill in higher mathematics, and mastering it opens up the possibility to study and understand complex mathematical concepts.
What is the speaker's view on online courses for learning proof writing?
-The speaker views online courses as a good alternative to traditional classroom learning, especially for those who cannot attend college. The key advantage of online courses, as per the speaker, is the opportunity to receive feedback, which is crucial for learning proof writing.
What are some of the challenges faced by learners when writing proofs?
-Challenges include understanding the structure and logic of proofs, creating clear and correct proofs, and getting feedback on their work. Additionally, the complexity of mathematical concepts and the need to master various types of proofs, such as direct proof, proof by contradiction, and proof by contrapositive, can be daunting.
Why does the speaker emphasize the importance of struggling with proofs?
-The speaker emphasizes struggling with proofs because it is through this process that a deeper understanding is achieved. Struggling with proofs helps learners to construct their own arguments, which is a critical skill for anyone aiming to master mathematical proof writing.
What is the significance of the speaker's mention of famous books and their terse style?
-The mention of terse and challenging books like 'Principles of Mathematical Analysis by Walter Rudin' highlights that often, the learning process in mathematics involves deciphering complex and concise explanations. This can be a valuable exercise in learning to think critically and independently in mathematics.
Outlines
📚 The Beauty of Mathematical Proofs
The paragraph discusses the transformative experience of learning to write mathematical proofs. It emphasizes that understanding the structure of proofs is crucial for truly appreciating mathematics. The speaker shares personal anecdotes about their journey from enjoying calculus to the 'game-changer' moment of grasping proofs. They also acknowledge the struggle and frustration learners may face but encourage perseverance, as proofs are fundamental to advancing in mathematical studies. The paragraph concludes with a teaser about sharing tips for reaching this level of understanding.
🎓 Learning from the Best: The Importance of Quality Education
This paragraph highlights the importance of learning from the best sources, recommending books and, ideally, taking a class with a good professor. The speaker shares their positive experience with two distinct professors who taught proofs in different ways, which was highly beneficial. The paragraph also touches on the value of feedback, the challenge of self-learning, and the accessibility of online courses. It concludes with encouragement for those who may not be able to attend college, suggesting alternative resources such as YouTube and the speaker's website.
📚 A Collection of Recommended Proof Books
The speaker provides a list of books that they have found useful in learning to write proofs. They discuss the merits of each book, including 'Book of Proof' by Hammock, 'How to Read and Do Proofs' by Daniel Solow, and 'Proofs' by Jake Cummings. The paragraph also mentions the value of having multiple books to cross-reference explanations and the importance of affordability when building a proof-writing resource library. The speaker concludes with a personal anecdote about their extensive collection of math books and the journey of collecting them over almost two decades.
🧐 The Struggle and Reward of Proof Writing
This paragraph delves into the challenges of proof writing, the necessity of struggling through it to learn, and the benefits of having a good grasp of proof techniques for higher-level math classes. The speaker shares an anecdote about a teacher who did not assist with homework, instead incorporating those problems into tests. They discuss the rationale behind teachers choosing books without answers for assignments and exams. The paragraph concludes with encouragement for those aiming for a math degree, emphasizing that mastering proof writing is the key to success in advanced mathematics.
Mindmap
Keywords
💡Mathematical proofs
💡Logical flow of ideas
💡Proof structure
💡Abstract algebra
💡Real analysis
💡Proof writing courses
💡Feedback
💡Online courses
💡Books on proof writing
💡YouTube videos
💡Mathematical logic
Highlights
Learning to write mathematical proofs is a transformative experience that can deepen one's appreciation for mathematics.
Understanding the structure of proofs is crucial for progressing to more advanced levels of mathematics.
Initially struggling with proofs is common, but perseverance is key to eventually mastering this skill.
The logical flow of ideas in a well-constructed proof can be as beautiful and satisfying as solving complex mathematical problems.
Once comfortable with proof writing, one can independently study advanced topics such as abstract algebra and real analysis.
Proof writing is a foundational skill that, while challenging, is essential for a deep understanding of mathematics.
Learning from the best possible sources, such as high-quality textbooks and experienced professors, is highly recommended for mastering proof writing.
A face-to-face college class with a good professor is one of the most effective ways to learn to write proofs.
Feedback on your proofs is critical for learning and improvement, which is often best provided in a classroom setting.
Online courses can also be a viable alternative for learning proof writing, offering structure and feedback.
The author recommends several books for learning proof writing, including 'Book of Proof' by Hammack and 'How to Read and Do Proofs' by Daniel Solow.
Books like 'Proofs' by Jake Cummings and 'Transition to Advanced Mathematics' by Chartrand are praised for their comprehensive approach to teaching proofs.
The author suggests that having access to multiple proof books can help in understanding different explanations and approaches to proof writing.
Some books are intentionally terse and lack detailed explanations to encourage students to struggle and learn through the process.
Teachers may choose books without answers for assignments and tests, which can contribute to the scarcity of detailed proofs in some texts.
Proof writing is a skill that can make earning a math degree achievable, despite the initial difficulty.
The author shares personal experiences and the impact of having two courses focused on proof writing simultaneously.
Online resources such as YouTube playlists on set theory, function sets, and abstract algebra can supplement learning and provide clean, correct proofs.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: