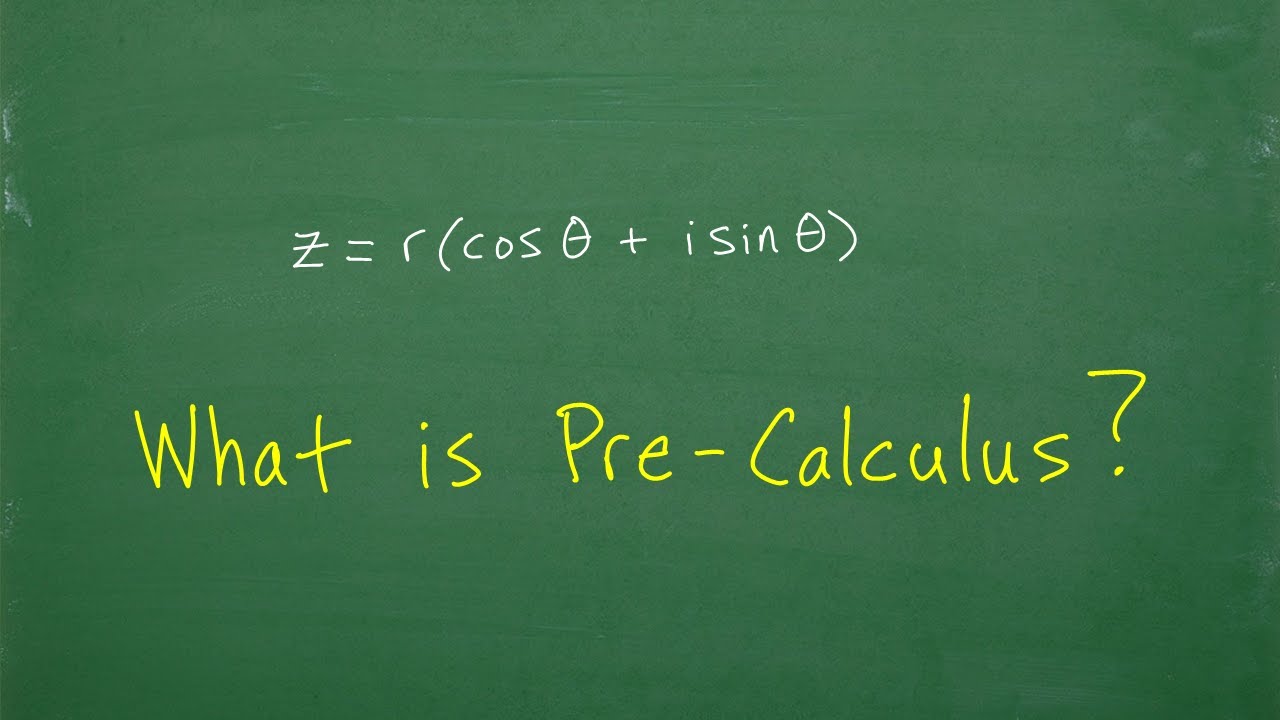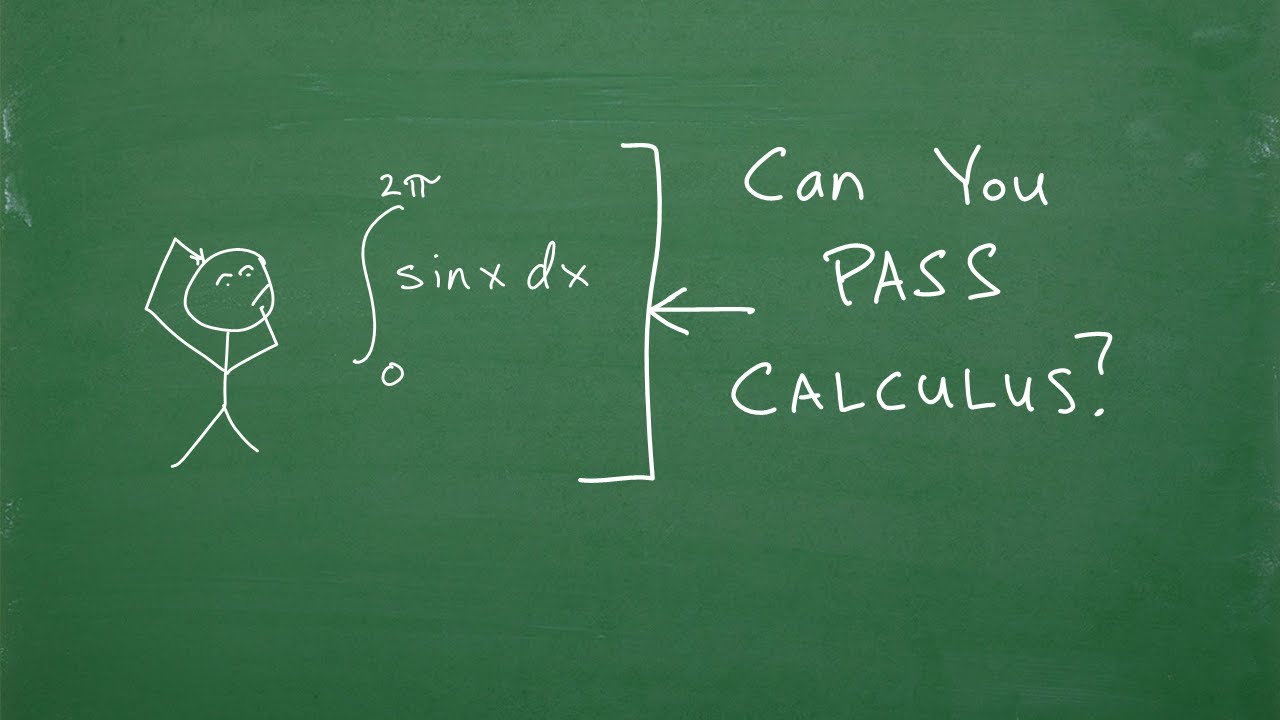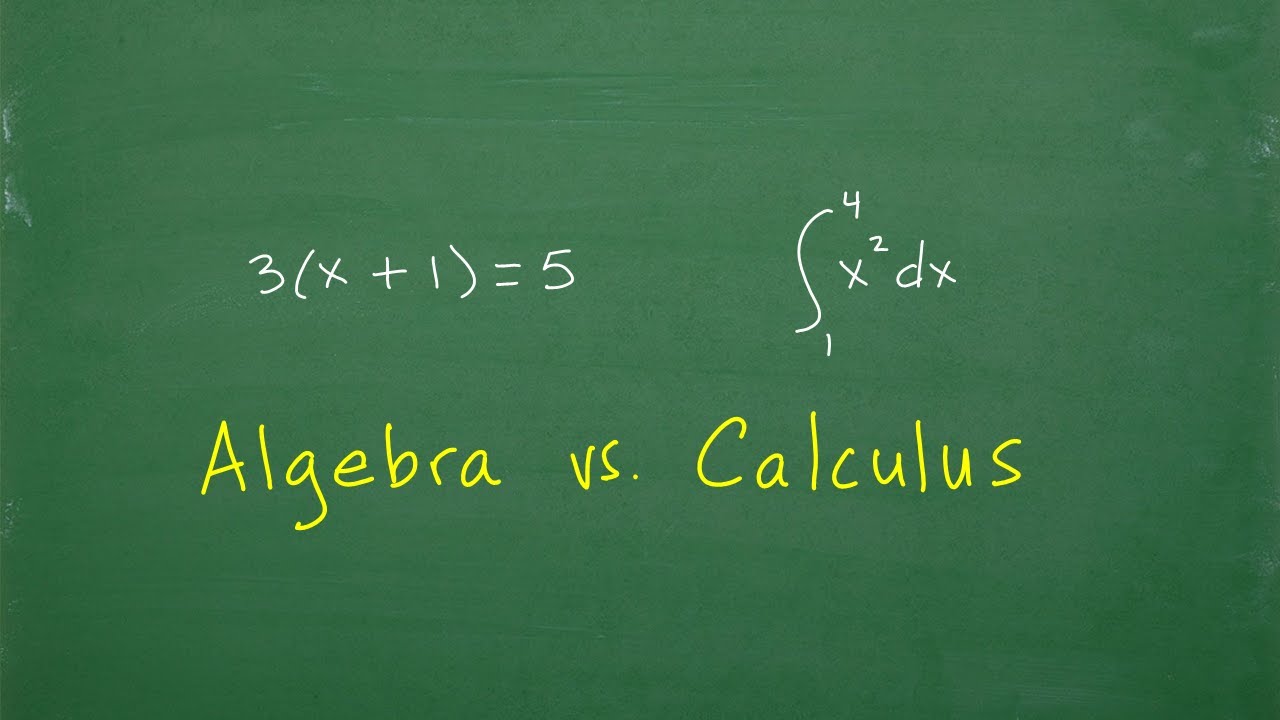Pre-Calculus vs. Calculus – What’s The Difference? Things you should consider…
TLDRIn this informative video script, John, the founder of TabletClass Math and an experienced math teacher, discusses the differences and similarities between pre-calculus and calculus. He emphasizes the importance of a strong foundation in pre-calculus for success in calculus, and outlines the typical curriculum for both subjects. John covers topics such as algebra, trigonometry, logarithms, and matrices in pre-calculus, and introduces limits, derivatives, and integration in calculus. He also provides advice for students on when to take these courses and how to prepare, especially for those considering STEM majors. The script offers a comprehensive look at the prerequisites and expectations in higher-level math courses, encouraging students to take thorough notes and master pre-calculus before moving on to calculus.
Takeaways
- 📚 **Pre-Calculus and Calculus Share a Name**: Both subjects are related and share the word 'calculus' in their titles, indicating a connection.
- ⚡ **Significant Differences**: Despite the shared name, there are substantial differences in the content and focus of pre-calculus versus calculus courses.
- 👨🏫 **Importance of Pre-Calculus**: Doing well in pre-calculus is emphasized as crucial for readiness to succeed in calculus.
- 🔗 **Pre-Calculus Course Recommendation**: The speaker, John, offers a comprehensive pre-calculus course and provides a link in the video description for those interested.
- 📈 **Understanding the Course Path**: Students typically take calculus in their first year of college, often after completing pre-calculus in 12th grade.
- 🏫 **College Placement**: Many colleges use placement exams like the ACCUPLACER to assess if students are prepared for calculus.
- 📘 **When to Take Calculus**: Calculus is generally taken for specific degree programs, particularly in STEM fields, rather than as a general degree requirement.
- 🔑 **Mastery of Pre-Calculus**: Mastery of pre-calculus topics is essential for a smooth transition into calculus, as these topics form the foundation for more advanced concepts.
- 📊 **Topics in Pre-Calculus**: Pre-calculus covers a wide range of topics including advanced trigonometry, logarithms, matrices, vectors, parametric equations, conic sections, sequences, series, and functions.
- 🧮 **Calculus Fundamentals**: Calculus introduces limits, derivatives, and integration, which are foundational for understanding more complex mathematical concepts.
- 🎓 **Educational Considerations**: Students are advised to consider their future educational and career paths when deciding whether to take pre-calculus or calculus, and to ensure they have a strong foundation in algebra before proceeding.
Q & A
What is the primary similarity between pre-calculus and calculus?
-The primary similarity between pre-calculus and calculus is that they both share the word 'calculus' in their titles.
Why is it important to do well in pre-calculus before taking calculus?
-It is important to do well in pre-calculus because it provides the foundational knowledge required for calculus. Concepts mastered in pre-calculus are essential for understanding calculus topics such as limits, derivatives, and integration.
Who is the founder of TabletClass Math and what is his role?
-John is the founder of TabletClass Math. He is also a middle and high school math teacher who provides comprehensive online pre-calculus courses and helps students with various math-related topics.
What is the general timeline for taking pre-calculus and calculus in high school?
-Most students take pre-calculus in the 12th grade, while calculus is typically taken in the first year of college. However, some students may take pre-calculus in the 11th grade and then an AP calculus course in the 12th grade.
What are some of the topics covered in a pre-calculus course?
-Topics in a pre-calculus course include advanced trigonometry, logarithms, matrices, vectors, parametric equations, conic sections, sequences and series, functions, domain and range, partial fractions, rational equations, and asymptotes.
Why might a student consider taking a statistics course instead of pre-calculus?
-A student might consider taking a statistics course instead of pre-calculus if they are certain that they will not pursue a STEM-related field or a major that requires calculus.
What are the two main components of single variable calculus?
-The two main components of single variable calculus are differentiation and integration.
What is the significance of mastering algebra before taking pre-calculus?
-Mastering algebra before taking pre-calculus is significant because algebraic concepts are foundational to more advanced topics in pre-calculus and are prerequisites for understanding calculus.
What does the speaker suggest for students who are unsure about their major and are considering taking pre-calculus?
-The speaker suggests that students who are unsure about their major should still consider taking pre-calculus to keep the option open for taking calculus in the future, as it prepares them for more advanced math courses.
Why is it recommended to take good notes and perform well in pre-calculus?
-Taking good notes and performing well in pre-calculus is recommended because it helps solidify the foundational concepts that are crucial for success in calculus and other advanced math courses.
What kind of support does the speaker offer for students preparing for exams with a math section?
-The speaker offers a math help program that provides comprehensive support for students preparing for exams with a math section, such as the GED, SAT, ACT, GRE, GMAT, ASVAB, Accuplacer, and CLEP exams.
Outlines
📚 Introduction to Pre-Calculus and Calculus
John, the founder of Tablet Class Math and a middle and high school math teacher, introduces the topic of pre-calculus and calculus. He emphasizes the importance of doing well in pre-calculus to prepare for calculus. John also mentions his comprehensive online pre-calculus course and offers help for various math levels and tests, including the GED, SAT, ACT, GRE, GMAT, ASVAB, ACCUPLACER, and CLEP exam. He encourages viewers to share their thoughts on pre-calculus in the comments.
🎓 When and Why to Take Calculus
The video discusses when students typically take calculus, which is often as a first-year college course. It also explores why one would take calculus, which is usually required for specific majors or degree programs in STEM fields. John advises that if a student is unsure of their major, taking pre-calculus is a good idea to keep the option open for calculus in the future. He also touches on the possibility of taking a statistics course for those certain they won't pursue engineering or a related field.
🔢 Key Topics in Pre-Calculus
John outlines the various topics covered in a pre-calculus course, including advanced trigonometry, logarithms, matrices, vectors, parametric equations, conic sections, sequences and series, and functions. He stresses that a strong foundation in algebra is crucial and that pre-calculus serves as an introduction to calculus concepts such as differentiation and integration. The paragraph also highlights the importance of mastering pre-calculus for success in calculus.
🧮 Calculus Fundamentals and Expectations
The final paragraph delves into the fundamentals of calculus, including limits, derivatives, and integration. John explains that calculus builds upon pre-calculus knowledge and introduces new concepts. He mentions that calculus is typically a three-semester course, covering multi-variable calculus. The importance of understanding the material in pre-calculus before entering calculus is reiterated, as the calculus course expects students to have mastered pre-calculus topics. John concludes by encouraging viewers to like the video, subscribe to his channel, and consider his math help program for further assistance.
Mindmap
Keywords
💡Pre-calculus
💡Calculus
💡Trigonometry
💡Derivative
💡Integration
💡Limits
💡Algebra
💡Logarithms
💡Matrices
💡Vectors
💡Conic Sections
Highlights
Pre-calculus and calculus share the word 'calculus' in their titles but have significant differences.
The importance of performing well in pre-calculus to prepare for calculus is emphasized.
The founder of tablet class math, John, is a middle and high school math teacher offering a comprehensive pre-calculus course.
John's teaching style involves breaking down complex concepts into clear, understandable segments.
Pre-calculus is often taken in the 12th grade, while calculus is typically a first-year college course.
AP calculus courses in high school are equivalent to university-level courses.
Placement exams like the Accuplacer are used by universities to assess readiness for calculus.
Calculus is generally required for STEM-related degrees, while other majors may not require it.
Pre-calculus covers a wide range of topics including advanced trigonometry, logarithms, and matrices.
Concepts such as limits, derivatives, and integration are introduced in pre-calculus but fully explored in calculus.
Students are advised to take good notes and perform well in pre-calculus for better understanding in calculus.
Calculus involves serious mathematical concepts and requires a solid foundation in pre-calculus topics.
John offers a math help program and resources, including a link to his pre-calculus course in the video description.
Differentiating and integrating are the two major components of single-variable calculus.
The video provides a general comparison between pre-calculus and calculus to help viewers make informed decisions about their math education.
John encourages viewers to engage with the content by liking, subscribing, and commenting on their thoughts about pre-calculus and calculus.
The video concludes with a reminder that calculus, while challenging, is passable with hard work and preparation.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: