Finding the Domain of Functions (Precalculus - College Algebra 4)
TLDRThis video script delves into the concept of domain, particularly for functions defined over real numbers. It explains that the domain is the set of all permissible input values (real numbers) for a function that result in a real number output. The script highlights two main problem areas in determining domain: square roots and denominators. For square roots, the radicand (the number under the root) must be non-negative to avoid imaginary outputs. For denominators, the critical rule is that the denominator cannot be zero, as this would lead to an undefined expression. The script also touches on the treatment of these issues when they appear together in a function and emphasizes the importance of understanding these concepts for more advanced topics like logarithms, which will be discussed later. The summary underscores the need to exclude values that would result in an undefined or imaginary output, and how to express these exclusions in set-builder and interval notations. The script concludes by noting that if a function lacks square roots, denominators, or logarithms, its domain is all real numbers, and it provides examples to illustrate these principles.
Takeaways
- 📚 Domain refers to the set of all possible input values (x-values) for which a function produces a real output.
- ❌ Square roots can cause domain restrictions because the radicand (the number under the square root) must be non-negative to avoid imaginary numbers.
- 🚫 Denominators must not be zero as it leads to undefined expressions; thus, values that make the denominator zero are not included in the domain.
- 🔍 When finding the domain, consider both square roots and denominators separately and then combine the restrictions to find the overall domain.
- 💡 For square roots, set the radicand greater than or equal to zero to ensure real number outputs.
- ⛔ For fractions, set the denominator equal to zero and solve to find values that cannot be in the domain because they would make the expression undefined.
- 📈 Interval notation and set-builder notation are two common ways to express the domain of a function.
- 🚷 Exclusions in the domain can be represented by parentheses (not including the endpoint) or brackets (including the endpoint) in interval notation.
- 🔑 Understanding the behavior of square roots and denominators is crucial for determining the domain of more complex functions, including those with logarithms.
- 📌 If a function does not contain square roots, denominators, or logarithms, its domain is all real numbers.
- ✅ A common mistake is confusing the process for finding domain restrictions for square roots with that for denominators; they are different and should not be conflated.
Q & A
What is the domain of a function defined over real numbers?
-The domain of a function defined over real numbers is the set of all inputs (x-values) which, when applied to the function, result in real number outputs.
Why is it important to consider the domain when dealing with square roots?
-It is important because the radicand (the number under the square root) must be non-negative to ensure that the output is a real number. Negative numbers under a square root would result in an imaginary number, which is not allowed in the context of real-number functions.
How do you find the domain of a function with a square root?
-To find the domain, you set the radicand (the expression under the square root) greater than or equal to zero and solve for the variable. This ensures that the function will only output real numbers.
What is a common mistake students make when dealing with square roots and denominators?
-A common mistake is confusing the two concepts. Students might incorrectly set the radicand of a square root equal to zero, which is not the correct approach since the goal is to ensure the radicand is positive or zero, not to solve for when it equals zero.
Why can't the denominator of a fraction be zero?
-The denominator cannot be zero because division by zero is undefined in mathematics. It would result in an expression that does not have a value, which is not acceptable when working with functions that must produce real number outputs.
How do you represent the domain of a function in interval notation?
-Interval notation is a way to express the domain using parentheses and brackets to indicate whether the endpoints are included or excluded. For example, (a, b) represents all real numbers greater than a and less than b, while [a, b] includes the endpoints a and b.
What is a vertical asymptote in the context of a function's graph?
-A vertical asymptote is a vertical line that the graph of a function approaches but never crosses. It occurs where the denominator of a fraction is zero, creating an undefined value for the function at that point.
How does the domain affect the graph of a function with a square root in the numerator and a linear term in the denominator?
-The domain will exclude values that make the denominator zero, as this would create a vertical asymptote. Additionally, the domain will include values that keep the radicand of the square root non-negative to ensure real number outputs.
What is the domain of a function that has no square roots, denominators, or logarithms?
-If a function does not contain square roots, denominators, or logarithms, its domain is all real numbers, which can be represented as X is a real number, or in interval notation as (-∞, ∞).
How do you find the domain of a function with both a square root and a denominator?
-First, set the radicand of the square root greater than or equal to zero to ensure real number outputs from the square root. Then, set the denominator equal to zero and solve to find the values that are not allowed, as these would make the denominator zero and the function undefined.
Why is the domain important when studying functions?
-The domain is important because it determines the set of all possible inputs for which the function is defined. Understanding the domain is crucial for the correct application of the function, its graphing, and for avoiding mathematical errors such as division by zero or taking the square root of a negative number.
Outlines
😀 Introduction to Domain and Functions Defined Over Real Numbers
This paragraph introduces the concept of domain, specifically for functions defined over real numbers. It clarifies that the domain represents the set of inputs that yield real number outputs. The paragraph also distinguishes between functions that can produce real, imaginary, or complex outputs, but focuses on real numbers for the purpose of this discussion. It sets the stage for exploring the two main problem areas in defining the domain: square roots and denominators, with a mention of logarithms to be discussed later. The importance of understanding domain is emphasized for functions that we typically graph and work with in mathematics.
🔍 Problem Areas in Domain: Square Roots and Denominators
The second paragraph delves into the specifics of the two main problem areas in determining the domain of a function: square roots and denominators. It explains that square roots must have a non-negative radicand to avoid imaginary numbers, hence the inside of the square root should be positive or zero. On the other hand, denominators should never be zero as it leads to an undefined expression. The paragraph also clarifies common student misconceptions about setting the inside of a square root or a denominator equal to zero and solving for it, which is incorrect. It emphasizes the need to consider the nature of the function to determine valid inputs.
📌 Writing Domain: Set Builder Notation and Interval Notation
This paragraph discusses how to express the domain of a function using set builder notation and interval notation. It provides examples of how to write the domain for square roots and denominators, emphasizing the importance of including or excluding certain numbers based on whether they would result in a positive radicand for square roots or a non-zero denominator. The paragraph also touches on the concept of vertical asymptotes and how they relate to the domain of a function, particularly when denominators are zero.
🚫 Exclusions in Domain: Zero Denominators and Negative Square Roots
The fourth paragraph focuses on the exclusions in the domain that arise from zero denominators and negative numbers under square roots. It explains the concept of vertical asymptotes and holes in the function due to these exclusions. The zero product property is introduced to find values that make the denominator zero, which are then excluded from the domain. The paragraph also highlights the difference between square roots, which require non-negative values, and denominators, which cannot be zero, and how these requirements affect the domain.
🤔 Understanding Domain: Positive Square Roots and Defined Denominators
The fifth paragraph emphasizes the importance of understanding why certain numbers are excluded from the domain. It reiterates that square roots should be positive to ensure real number outputs and denominators should not be zero to avoid undefined expressions. The paragraph also discusses how to represent the domain using interval notation, including how to exclude specific numbers that would make the denominator zero. It stresses the need to not confuse the concepts of square roots and denominators when determining the domain.
📐 Domain with No Restrictions: Polynomials and Real Numbers
In this paragraph, the scenario is presented where a function does not contain square roots, denominators with variables, or logarithms. In such cases, the domain of the function is all real numbers. The paragraph explains how to represent this unrestricted domain using set builder notation and interval notation. It also provides an example of a function with both a square root and a denominator, showing how to determine the domain by addressing each issue independently and then combining the restrictions.
🧩 Combining Restrictions: Domain with Square Roots and Denominators
The sixth paragraph illustrates how to find the domain when a function includes both a square root and a denominator. It shows how to solve for the domain by considering the restrictions from both elements separately and then combining them. The paragraph also demonstrates how to express the domain using both set builder notation and interval notation, highlighting the importance of excluding specific numbers that would result in an undefined expression.
📐 Domain with Fractions and No Restrictions: Constant Denominators
The seventh paragraph discusses the domain of fractions where the denominator is a constant, ensuring that it cannot be zero and thus the domain includes all real numbers. It also addresses the case of a square root in the denominator, explaining that if the expression under the square root, when squared and increased by one, cannot be negative, then the domain is all real numbers. The paragraph emphasizes the importance of understanding the nature of the expressions in the denominator and the square root to determine the domain.
🔚 Conclusion: Domain and Function Operations
The final paragraph concludes the discussion on domain and its importance in the context of functions. It summarizes the key points about square roots, denominators, and their interplay in determining the domain. The paragraph also hints at the upcoming topic of function operations and their domains, setting the stage for the next video in the series.
Mindmap
Keywords
💡Domain
💡Real Numbers
💡Square Roots
💡Denominators
💡Logarithms
💡Undefined
💡Imaginary Numbers
💡Set Builder Notation
💡Interval Notation
💡Zero Product Property
💡Vertical Asymptote
Highlights
The video discusses the concept of domain for functions defined over real numbers.
The domain is defined as the set of inputs for a function that results in a real number output.
Two main problem areas for domain are square roots and denominators of fractions.
Square roots should have a radicand (the number under the root) that is non-negative to avoid imaginary numbers.
Denominators in fractions must not be zero to avoid undefined expressions.
Logarithms, which will be discussed later, add another problem area for the domain of functions.
The video explains that square roots and denominators are treated differently when finding the domain.
For square roots, inputs must be chosen such that the radicand is positive or zero.
For fractions, the domain excludes values that make the denominator zero.
The video uses examples to illustrate how to find the domain for functions involving square roots and fractions.
Interval notation and set-builder notation are two methods introduced to express the domain.
The video emphasizes the importance of understanding domain for functions to avoid errors in calculations and graphing.
The concept of vertical asymptotes is briefly introduced in relation to the domain of functions with denominators.
The video clarifies common student misconceptions about setting the radicand of a square root or the denominator equal to zero.
The importance of domain is emphasized, especially in higher mathematics and calculus.
The video provides a recap to ensure understanding of the two main issues concerning the domain: square roots and denominators.
For functions without square roots, denominators, or logarithms, the domain is all real numbers.
The video concludes with a reminder that understanding the domain is crucial for accurate mathematical analysis and problem-solving.
Transcripts
Browse More Related Video
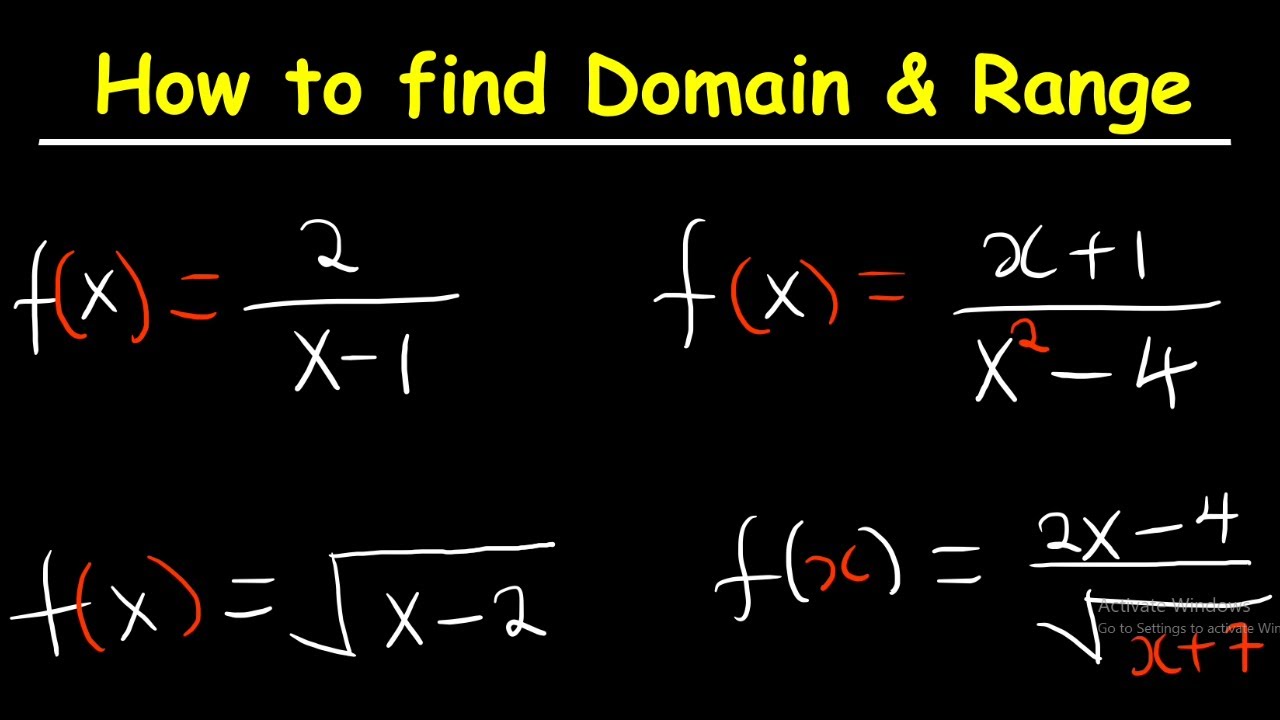
Domain and Range of a Function
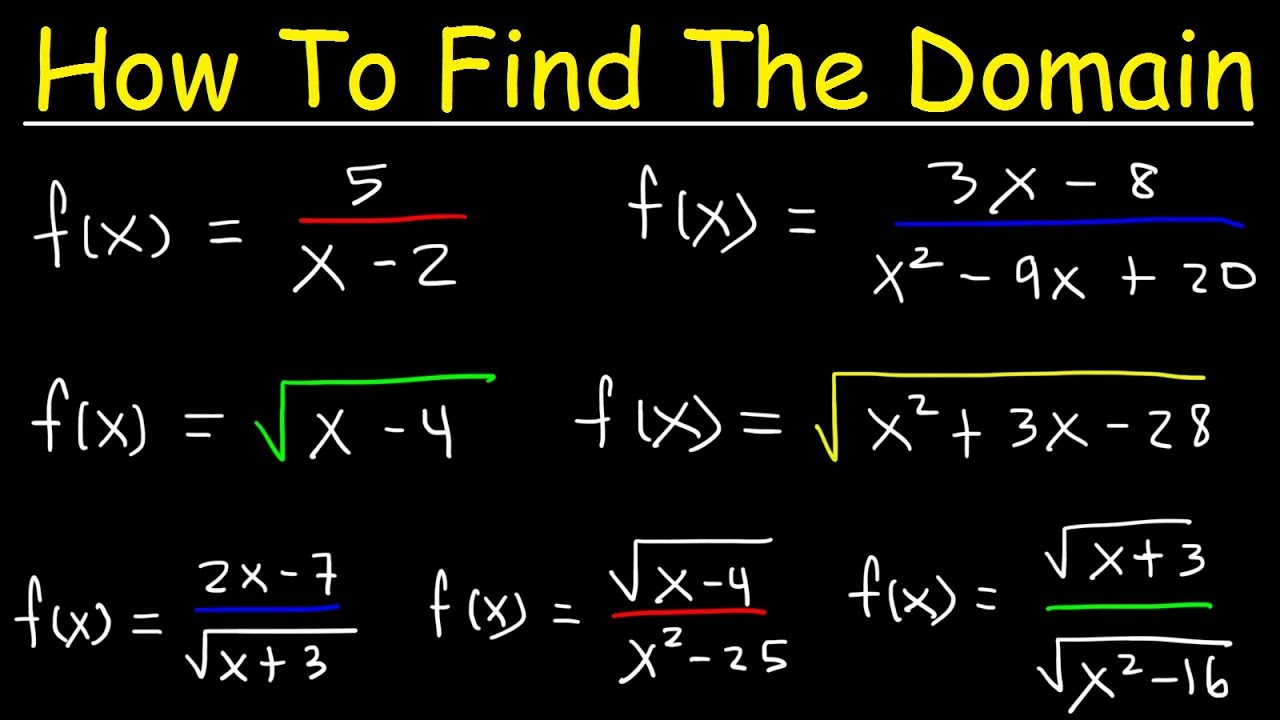
How To Find The Domain of a Function - Radicals, Fractions & Square Roots - Interval Notation

How to Evaluate Functions (Precalculus - College Algebra 3)
Imaginary Numbers Are Real [Part 1: Introduction]

Complex Numbers: Operations, Complex Conjugates, and the Linear Factorization Theorem

Features of Graphs, Domain, Range (Precalculus - College Algebra 7)
5.0 / 5 (0 votes)
Thanks for rating: