The Planck scale: Is there a fundamental limit to space and time?
TLDRThis script delves into the limits of human knowledge and the fundamental forces of nature, using the example of two electrons to explore the interplay between quantum mechanics, special relativity, and gravity. It explains how the gravitational force, though weak, is crucial for cosmic structures and life itself. The discussion introduces Heisenberg's uncertainty principle and Einstein's mass-energy equivalence, leading to the concept of the Planck length—a proposed lower limit to space and time scales where a new quantum theory of gravity is needed. The script challenges viewers to ponder the mysteries of the universe, encouraging curiosity and the pursuit of knowledge.
Takeaways
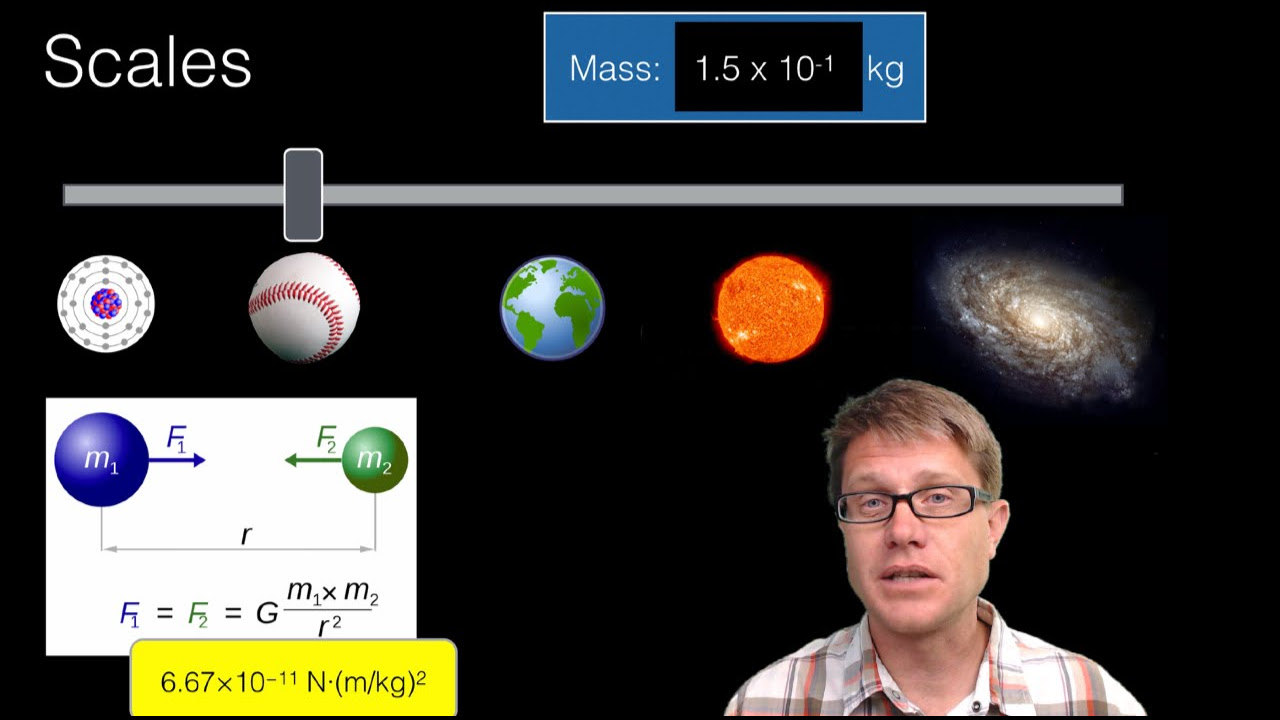
- 🔬 Science has expanded our understanding from the largest galaxies to the smallest subatomic particles, but our senses limit what we can directly experience.
- 👀 The human eye cannot see the trillions of molecules in a droplet of water or the protons and neutrons within an atom's nucleus.
- 📡 Modern technology has extended our senses, allowing us to explore smaller structures and revealing the complexity of the microcosm.
- 🤔 The deeper we probe into nature, the more we discover, but there might be a fundamental limit to this exploration in terms of size.
- 🧲 The forces acting on two electrons include gravitational attraction and electrostatic repulsion, with the latter being significantly stronger.
- ⚡ The electrostatic force between two electrons is approximately 10^42 times stronger than the gravitational force.
- 🌌 Despite its weakness, gravity plays a crucial role in the formation of stars, galaxies, and the evolution of life.
- 🚀 Quantum mechanics introduces uncertainty at small scales, causing jitters in particles like electrons when squeezed into smaller spaces.
- 🔗 Heisenberg's uncertainty principle and Einstein's theory of relativity suggest a limit to how small we can probe, known as the Planck length.
- 🌑 At extremely small scales, the gravitational force can become strong enough to create a black hole, preventing any information from escaping.
- ✨ The Planck length, derived from fundamental constants, represents a fundamental limit to our knowledge of space and the scales we can meaningfully probe.
Q & A
How has science expanded our understanding of the universe over the last 400 years?
-Science has pushed back the limits of what we know, from the largest spiral galaxies to the smallest subatomic particles, using technology to probe smaller structures and reveal detailed features of the micro cosmos.
What are the limitations of human senses in exploring the world?
-Human senses allow us to explore our immediate environment but place a fundamental limit on what can be directly experienced. For example, the unaided eye can see a water droplet but not the molecules or atoms inside it.
How do gravitational and electrostatic forces compare between two electrons?
-The electrostatic force between two electrons is significantly stronger than the gravitational force, by a factor of 10^42 times, because electrostatic forces obey an inverse square law, similar to gravitational forces.
What is the significance of Heisenberg's uncertainty principle in quantum mechanics?
-Heisenberg's uncertainty principle states that as we confine an object within a smaller region of space, the uncertainty in its momentum increases, leading to an increase in quantum mechanical jittering energy.
What happens when electrons are squeezed closer together to a very small distance?
-As electrons are squeezed closer, quantum mechanical jittering increases their energy, and at a certain point, this energy becomes enough to create new particles, ultimately leading to conditions where a black hole might form.
What is the Planck length and why is it significant?
-The Planck length, approximately 10^-35 meters, represents a fundamental limit to the distances at which we can probe nature. Beyond this limit, the combined effects of quantum mechanics and general relativity make meaningful measurements impossible.
How does the escape velocity of a planet relate to the formation of black holes?
-The escape velocity is the speed needed to overcome an object's gravitational pull. If an object is so dense that its escape velocity exceeds the speed of light, it forms a black hole, from which nothing, not even light, can escape.
How do the fundamental constants of nature, like Planck's constant, the speed of light, and gravitational constant, contribute to understanding the Planck length?
-These constants, when combined through dimensional analysis, help define the Planck length, which is derived from their respective units and indicates the scale at which quantum gravitational effects dominate.
What is the Planck time and what does it signify?
-The Planck time, approximately 10^-44 seconds, represents the time it takes light to travel the Planck length. It signifies the earliest stage of the universe where quantum gravitational effects dominate, and below this scale, the concept of time may lose its meaning.
Why is it challenging to understand space-time at extremely small scales?
-At scales smaller than the Planck length, space-time may not behave like a continuous fabric. Quantum gravitational effects become significant, and a new theory of quantum gravity is likely needed to understand these scales.
Outlines
🔬 The Expansion of Human Senses Through Science
This paragraph discusses the remarkable journey of science over the past 400 years, expanding our understanding from the vast spiral galaxies to the minute subatomic particles. It highlights the limitations of human senses and how technology has enhanced our ability to explore the microcosm. The script introduces a fundamental question about the limits of exploration in size, setting the stage for a deeper dive into the forces at play when two electrons are brought close together. It explains the gravitational and electrostatic forces, using Newton's and Coulomb's laws, and reveals the immense strength of electrostatic repulsion compared to gravitational attraction, emphasizing the latter's crucial role in the formation of cosmic structures.
🌌 The Role of Gravity and Quantum Mechanics in the Cosmos
The second paragraph delves into the unique role of gravity in the universe's large-scale structure despite its relative weakness, leading to the formation of stars and galaxies. It introduces the complexities added by quantum mechanics at small scales, particularly Heisenberg's uncertainty principle, which introduces a quantum jitter that affects the energy of particles as they are compressed. The script explores the implications of this principle, suggesting that at a certain point, the energy of this jitter can be equivalent to the mass of an electron, hinting at the interplay between quantum mechanics and Einstein's theory of relativity at extremely small scales.
🌐 The Interplay of Electrostatic and Gravitational Forces
This paragraph investigates the point at which gravitational force becomes comparable to electrostatic force as particles are compressed to smaller scales. It discusses the calculation of the distance at which this balance occurs and introduces the concept of a black hole, where gravity is so strong that not even light can escape. The script explains the theoretical formation of a black hole through the increase of mass due to quantum uncertainty and the corresponding increase in gravitational force, leading to an event horizon where information is lost.
🌒 The Formation of Black Holes and the Schwarzschild Radius
The fourth paragraph explores the concept of escape velocity and how it relates to the density of an object, leading to the formation of a black hole when the escape velocity exceeds the speed of light. It introduces the Schwarzschild radius as the critical size at which an object becomes a black hole. The script connects this concept back to the electron example, suggesting that as electrons are compressed, they increase in mass and could theoretically form a black hole, indicating a fundamental limit to how small we can probe the universe.
📏 The Planck Length and the Fundamental Limits of Space
This paragraph introduces the Planck length as the fundamental lower limit to our knowledge of space, derived from the interplay of quantum mechanics, relativity, and gravity. It explains how the Planck length represents the scale at which a black hole would form from the mass of two electrons, making further probing of smaller distances impossible. The script also touches on the Planck mass and the concept of a 'flea egg's worth of mass' as an analogy for the mass required to reach this scale, emphasizing the extreme nature of these scales in theoretical physics.
⏱️ The Planck Time and the Earliest Stages of the Universe
The final paragraph discusses the Planck time, an incredibly small unit of time that represents the earliest point in the universe's history that we can theoretically understand. It connects this concept to the Planck epoch, the initial stage of the universe before which our current physical theories break down. The script ponders the nature of space-time at these scales and the need for a new quantum theory of gravity, encouraging viewers to be curious and to continue exploring the mysteries of the universe.
Mindmap
Keywords
💡Subatomic Particles
💡Senses
💡Microcosm
💡Electrons
💡Gravitational Force
💡Electrostatic Force
💡Heisenberg's Uncertainty Principle
💡Einstein's Theory of Relativity
💡Black Hole
💡Planck Length
💡Quantum Gravity
Highlights
Science has expanded our understanding from the largest galaxies to the smallest subatomic particles.
Human senses are limited and cannot directly experience the subatomic world.
Modern technology has extended our senses to explore smaller structures in the microcosm.
There may be a fundamental lower limit to the size of objects or regions in space.
Electrons, as fundamental particles, exhibit both gravitational attraction and electrostatic repulsion.
The electrostatic force between two electrons is vastly stronger than the gravitational force.
Gravitational force, despite being weaker, plays a crucial role in the formation of stars and galaxies.
Quantum mechanics introduces uncertainty at small scales, affecting the behavior of electrons.
Heisenberg's uncertainty principle leads to a quantum jitter that increases electron energy as they are squeezed closer.
Einstein's theory of relativity connects energy and mass, influencing the scenario of two electrons being compressed.
A calculation of the distance at which quantum effects become significant in electron compression.
As electrons are pushed closer, gravity's influence increases due to added mass from quantum uncertainty.
The point at which gravitational and electrostatic forces balance is at an extremely small distance.
The formation of a black hole is related to the inability to probe smaller distances due to increased mass and gravity.
The Schwarzschild radius defines the size at which an object becomes a black hole, preventing information escape.
The Planck length represents a fundamental limit to our knowledge of space and the scales we can meaningfully probe.
The Planck mass and time are derived from fundamental constants and represent the scale of quantum gravitational effects.
The Planck era in cosmology represents the earliest stage of the universe's history, where physical theories are uncertain.
Theoretical physicists are considering the need for a new quantum theory of gravity to understand these extreme scales.
The concept of space-time atoms suggests a discrete structure at small scales, challenging the continuous view of relativity.
The challenge of understanding the universe's existence and the need for curiosity and persistence in scientific exploration.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: